
Optical tweezers are scientific instruments that use a highly focused laser beam to hold and move microscopic and sub-microscopic objects like atoms, nanoparticles and droplets, in a manner similar to tweezers. If the object is held in air or vacuum without additional support, it can be called optical levitation.

A polaron is a quasiparticle used in condensed matter physics to understand the interactions between electrons and atoms in a solid material. The polaron concept was proposed by Lev Landau in 1933 and Solomon Pekar in 1946 to describe an electron moving in a dielectric crystal where the atoms displace from their equilibrium positions to effectively screen the charge of an electron, known as a phonon cloud. This lowers the electron mobility and increases the electron's effective mass.
In physics, a Feshbach resonance can occur upon collision of two slow atoms, when they temporarily stick together forming an unstable compound with short lifetime. It is a feature of many-body systems in which a bound state is achieved if the coupling(s) between at least one internal degree of freedom and the reaction coordinates, which lead to dissociation, vanish. The opposite situation, when a bound state is not formed, is a shape resonance. It is named after Herman Feshbach, a physicist at MIT.
Heisenberg's microscope is a thought experiment proposed by Werner Heisenberg that has served as the nucleus of some commonly held ideas about quantum mechanics. In particular, it provides an argument for the uncertainty principle on the basis of the principles of classical optics.
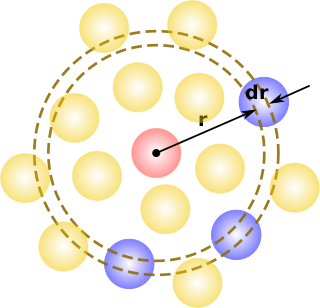
In statistical mechanics, the radial distribution function, in a system of particles, describes how density varies as a function of distance from a reference particle.

Neutron spin echo spectroscopy is an inelastic neutron scattering technique invented by Ferenc Mezei in the 1970s and developed in collaboration with John Hayter. In recognition of his work and in other areas, Mezei was awarded the first Walter Haelg Prize in 1999.
The Gross–Pitaevskii equation describes the ground state of a quantum system of identical bosons using the Hartree–Fock approximation and the pseudopotential interaction model.

William Allan Bardeen is an American theoretical physicist who worked at the Fermi National Accelerator Laboratory. He is renowned for his foundational work on the chiral anomaly, the Yang-Mills and gravitational anomalies, the development of quantum chromodynamics and the scheme frequently used in perturbative analysis of experimentally observable processes such as deep inelastic scattering, high energy collisions and flavor changing processes.
A composite fermion is the topological bound state of an electron and an even number of quantized vortices, sometimes visually pictured as the bound state of an electron and, attached, an even number of magnetic flux quanta. Composite fermions were originally envisioned in the context of the fractional quantum Hall effect, but subsequently took on a life of their own, exhibiting many other consequences and phenomena.
Microrheology is a technique used to measure the rheological properties of a medium, such as microviscosity, via the measurement of the trajectory of a flow tracer. It is a new way of doing rheology, traditionally done using a rheometer. There are two types of microrheology: passive microrheology and active microrheology. Passive microrheology uses inherent thermal energy to move the tracers, whereas active microrheology uses externally applied forces, such as from a magnetic field or an optical tweezer, to do so. Microrheology can be further differentiated into 1- and 2-particle methods.
Diffusing-wave spectroscopy (DWS) is an optical technique derived from dynamic light scattering (DLS) that studies the dynamics of scattered light in the limit of strong multiple scattering. It has been widely used in the past to study colloidal suspensions, emulsions, foams, gels, biological media and other forms of soft matter. If carefully calibrated, DWS allows the quantitative measurement of microscopic motion in a soft material, from which the rheological properties of the complex medium can be extracted via the microrheology approach.
The Vicsek model is a mathematical model used to describe active matter. One motivation of the study of active matter by physicists is the rich phenomenology associated to this field. Collective motion and swarming are among the most studied phenomena. Within the huge number of models that have been developed to catch such behavior from a microscopic description, the most famous is the model introduced by Tamás Vicsek et al. in 1995.

In quantum electrodynamics (QED), the Schwinger limit is a scale above which the electromagnetic field is expected to become nonlinear. The limit was first derived in one of QED's earliest theoretical successes by Fritz Sauter in 1931 and discussed further by Werner Heisenberg and his student Hans Heinrich Euler. The limit, however, is commonly named in the literature for Julian Schwinger, who derived the leading nonlinear corrections to the fields and calculated the rate of electron–positron pair production in a strong electric field. The limit is typically reported as a maximum electric field or magnetic field before nonlinearity for the vacuum of
Double ionization is a process of formation of doubly charged ions when laser radiation is exerted on neutral atoms or molecules. Double ionization is usually less probable than single-electron ionization. Two types of double ionization are distinguished: sequential and non-sequential.
The Optical Stretcher is a dual-beam optical trap that is used for trapping and deforming ("stretching") micrometer-sized soft matter particles, such as biological cells in suspension. The forces used for trapping and deforming objects arise from photon momentum transfer on the surface of the objects, making the Optical Stretcher - unlike atomic force microscopy or micropipette aspiration - a tool for contact-free rheology measurements.

Dieter Pohl is a German–Swiss physicist. He became known especially for his pioneering works in nano-optics, near field optics (NFO), and plasmonics.
Quantum microscopy allows microscopic properties of matter and quantum particles to be measured and imaged. Various types of microscopy use quantum principles. The first microscope to do so was the scanning tunneling microscope, which paved the way for development of the photoionization microscope and the quantum entanglement microscope.
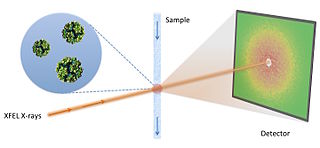
Fluctuation X-ray scattering (FXS) is an X-ray scattering technique similar to small-angle X-ray scattering (SAXS), but is performed using X-ray exposures below sample rotational diffusion times. This technique, ideally performed with an ultra-bright X-ray light source, such as a free electron laser, results in data containing significantly more information as compared to traditional scattering methods.
Many experimental realizations of self-propelled particles exhibit a strong tendency to aggregate and form clusters, whose dynamics are much richer than those of passive colloids. These aggregates of particles form for a variety of reasons, from chemical gradients to magnetic and ultrasonic fields. Self-propelled enzyme motors and synthetic nanomotors also exhibit clustering effects in the form of chemotaxis. Chemotaxis is a form of collective motion of biological or non-biological particles toward a fuel source or away from a threat, as observed experimentally in enzyme diffusion and also synthetic chemotaxis or phototaxis. In addition to irreversible schooling, self-propelled particles also display reversible collective motion, such as predator–prey behavior and oscillatory clustering and dispersion.
Spin squeezing is a quantum process that decreases the variance of one of the angular momentum components in an ensemble of particles with a spin. The quantum states obtained are called spin squeezed states. Such states have been proposed for quantum metrology, to allow a better precision for estimating a rotation angle than classical interferometers.

















