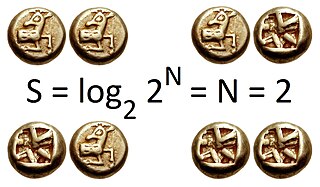For time invariant discrete memoryless sources
The source coding theorem states that for any  and any discrete-time i.i.d. source such as
and any discrete-time i.i.d. source such as  and for any rate less than the entropy of the source, there is large enough
and for any rate less than the entropy of the source, there is large enough  and an encoder that takes
and an encoder that takes  i.i.d. repetition of the source,
i.i.d. repetition of the source,  , and maps it to
, and maps it to  binary bits such that the source symbols
binary bits such that the source symbols  are recoverable from the binary bits with probability at least
are recoverable from the binary bits with probability at least  .
.
Let  be the total number of possible messages. Next map each of the possible source output sequences to one of the messages randomly using a uniform distribution and independently from everything else. When a source is generated the corresponding message
be the total number of possible messages. Next map each of the possible source output sequences to one of the messages randomly using a uniform distribution and independently from everything else. When a source is generated the corresponding message  is then transmitted to the destination. The message gets decoded to one of the possible source strings. In order to minimize the probability of error the decoder will decode to the source sequence
is then transmitted to the destination. The message gets decoded to one of the possible source strings. In order to minimize the probability of error the decoder will decode to the source sequence  that maximizes
that maximizes  , where
, where  denotes the event that message
denotes the event that message  was transmitted. This rule is equivalent to finding the source sequence
was transmitted. This rule is equivalent to finding the source sequence  among the set of source sequences that map to message
among the set of source sequences that map to message  that maximizes
that maximizes  . This reduction follows from the fact that the messages were assigned randomly and independently of everything else.
. This reduction follows from the fact that the messages were assigned randomly and independently of everything else.
Thus, as an example of when an error occurs, supposed that the source sequence  was mapped to message
was mapped to message  as was the source sequence
as was the source sequence  . If
. If  was generated at the source, but
was generated at the source, but  then an error occurs.
then an error occurs.
Let  denote the event that the source sequence
denote the event that the source sequence  was generated at the source, so that
was generated at the source, so that  Then the probability of error can be broken down as
Then the probability of error can be broken down as  Thus, attention can be focused on finding an upper bound to the
Thus, attention can be focused on finding an upper bound to the  .
.
Let  denote the event that the source sequence
denote the event that the source sequence  was mapped to the same message as the source sequence
was mapped to the same message as the source sequence  and that
and that  . Thus, letting
. Thus, letting  denote the event that the two source sequences
denote the event that the two source sequences  and
and  map to the same message, we have that
map to the same message, we have that

and using the fact that  and is independent of everything else have that
and is independent of everything else have that

A simple upper bound for the term on the left can be established as

for some arbitrary real number  This upper bound can be verified by noting that
This upper bound can be verified by noting that  either equals
either equals  or
or  because the probabilities of a given input sequence are completely deterministic. Thus, if
because the probabilities of a given input sequence are completely deterministic. Thus, if  then
then  so that the inequality holds in that case. The inequality holds in the other case as well because
so that the inequality holds in that case. The inequality holds in the other case as well because

for all possible source strings. Thus, combining everything and introducing some  , have that
, have that

Where the inequalities follow from a variation on the Union Bound. Finally applying this upper bound to the summation for  have that:
have that:

Where the sum can now be taken over all  because that will only increase the bound. Ultimately yielding that
because that will only increase the bound. Ultimately yielding that

Now for simplicity let  so that
so that  Substituting this new value of
Substituting this new value of  into the above bound on the probability of error and using the fact that
into the above bound on the probability of error and using the fact that  is just a dummy variable in the sum gives the following as an upper bound on the probability of error:
is just a dummy variable in the sum gives the following as an upper bound on the probability of error:

 and each of the components of
and each of the components of  are independent. Thus, simplifying the above equation yields
are independent. Thus, simplifying the above equation yields

The term in the exponent should be maximized over  in order to achieve the highest upper bound on the probability of error.
in order to achieve the highest upper bound on the probability of error.
Letting  see that the error exponent for the source coding case is:
see that the error exponent for the source coding case is: