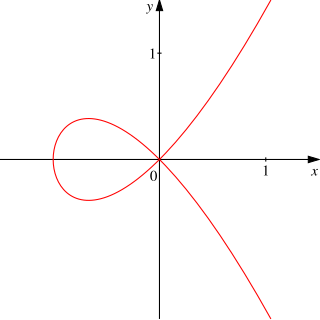Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical problems about these sets of zeros.
In algebra and algebraic geometry, the spectrum of a commutative ring R, denoted by , is the set of all prime ideals of R. It is commonly augmented with the Zariski topology and with a structure sheaf, turning it into a locally ringed space. A locally ringed space of this form is called an affine scheme.

In algebraic geometry and commutative algebra, the Zariski topology is a topology on algebraic varieties, introduced primarily by Oscar Zariski and later generalized for making the set of prime ideals of a commutative ring a topological space, called the spectrum of the ring.

Commutative algebra is the branch of algebra that studies commutative rings, their ideals, and modules over such rings. Both algebraic geometry and algebraic number theory build on commutative algebra. Prominent examples of commutative rings include polynomial rings; rings of algebraic integers, including the ordinary integers ; and p-adic integers.

In algebraic geometry, an algebraic group is a group that is an algebraic variety, such that the multiplication and inversion operations are given by regular maps on the variety.

Algebraic varieties are the central objects of study in algebraic geometry. Classically, an algebraic variety is defined as the set of solutions of a system of polynomial equations over the real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition.
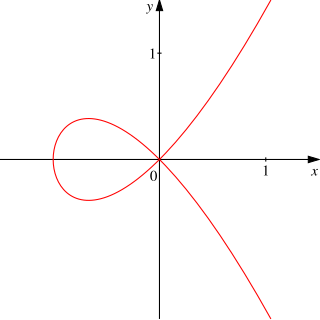
In algebraic geometry, an affine variety over an algebraically closed field k is the zero-locus in the affine n-space of some finite family of polynomials of n variables with coefficients in k that generate a prime ideal. If the condition of generating a prime ideal is removed, such a set is called an (affine) algebraic set. A Zariski open subvariety of an affine variety is called a quasi-affine variety.

In algebraic geometry, a projective variety over an algebraically closed field k is a subset of some projective n-space over k that is the zero-locus of some finite family of homogeneous polynomials of n + 1 variables with coefficients in k, that generate a prime ideal, the defining ideal of the variety. Equivalently, an algebraic variety is projective if it can be embedded as a Zariski closed subvariety of .
In mathematics, a scheme is a mathematical structure that enlarges the notion of algebraic variety in several ways, such as taking account of multiplicities and allowing "varieties" defined over any commutative ring.
In algebraic geometry, the Zariski tangent space is a construction that defines a tangent space at a point P on an algebraic variety V. It does not use differential calculus, being based directly on abstract algebra, and in the most concrete cases just the theory of a system of linear equations.
In algebraic geometry, an algebraic variety or scheme X is normal if it is normal at every point, meaning that the local ring at the point is an integrally closed domain. An affine variety X is normal if and only if the ring O(X) of regular functions on X is an integrally closed domain. A variety X over a field is normal if and only if every finite birational morphism from any variety Y to X is an isomorphism.
In mathematics, and specifically in algebraic geometry, the concept of irreducible component is used to make formal the idea that a set such as defined by the equation
In algebraic geometry, a generic pointP of an algebraic variety X is, roughly speaking, a point at which all generic properties are true, a generic property being a property which is true for almost every point.
This is a glossary of arithmetic and diophantine geometry in mathematics, areas growing out of the traditional study of Diophantine equations to encompass large parts of number theory and algebraic geometry. Much of the theory is in the form of proposed conjectures, which can be related at various levels of generality.
Affine geometry, broadly speaking, is the study of the geometrical properties of lines, planes, and their higher dimensional analogs, in which a notion of "parallel" is retained, but no metrical notions of distance or angle are. Affine spaces differ from linear spaces in that they do not have a distinguished choice of origin. So, in the words of Marcel Berger, "An affine space is nothing more than a vector space whose origin we try to forget about, by adding translations to the linear maps." Accordingly, a complex affine space, that is an affine space over the complex numbers, is like a complex vector space, but without a distinguished point to serve as the origin.
In mathematics, an algebraic cycle on an algebraic variety V is, roughly speaking, a homology class on V that is represented by a linear combination of subvarieties of V. Therefore, the algebraic cycles on V are the part of the algebraic topology of V that is directly accessible in algebraic geometry. With the formulation of some fundamental conjectures in the 1950s and 1960s, the study of algebraic cycles became one of the main objectives of the algebraic geometry of general varieties.

In algebraic geometry, the problem of resolution of singularities asks whether every algebraic variety V has a resolution, a non-singular variety W with a proper birational map W→V. For varieties over fields of characteristic 0 this was proved in Hironaka (1964), while for varieties over fields of characteristic p it is an open problem in dimensions at least 4.
In algebraic geometry, Zariski's main theorem, proved by Oscar Zariski (1943), is a statement about the structure of birational morphisms stating roughly that there is only one branch at any normal point of a variety. It is the special case of Zariski's connectedness theorem when the two varieties are birational.
In algebraic geometry, a morphism between algebraic varieties is a function between the varieties that is given locally by polynomials. It is also called a regular map. A morphism from an algebraic variety to the affine line is also called a regular function. A regular map whose inverse is also regular is called biregular, and they are isomorphisms in the category of algebraic varieties. Because regular and biregular are very restrictive conditions – there are no non-constant regular functions on projective varieties – the weaker condition of a rational map and birational maps are frequently used as well.