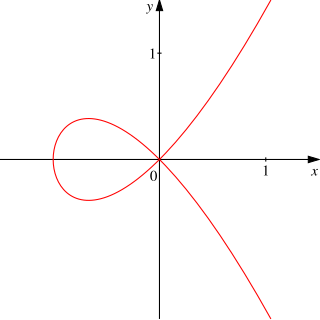Imaginary line (mathematics)

Last updated

In complex geometry, an imaginary line is a straight line that only contains one real point. It can be proven that this point is the intersection point with the conjugated line. [1]

In mathematics, complex geometry is the study of complex manifolds and functions of several complex variables. Application of transcendental methods to algebraic geometry falls in this category, together with more geometric aspects of complex analysis.

In geometry, a real point is a point in the complex projective plane with homogeneous coordinates (x,y,z) for which there exists a nonzero complex number λ such that λx, λy, and λz are all real numbers.

It is a special case of an imaginary curve.

An imaginary line is found in the complex projective plane P2(C) where points are represented by three homogeneous coordinates

In mathematics, the complex projective plane, usually denoted P2(C), is the two-dimensional complex projective space. It is a complex manifold described by three complex coordinates

Homogeneous coordinates

In mathematics, homogeneous coordinates or projective coordinates, introduced by August Ferdinand Möbius in his 1827 work Der barycentrischeCalcül, are a system of coordinates used in projective geometry, as Cartesian coordinates are used in Euclidean geometry. They have the advantage that the coordinates of points, including points at infinity, can be represented using finite coordinates. Formulas involving homogeneous coordinates are often simpler and more symmetric than their Cartesian counterparts. Homogeneous coordinates have a range of applications, including computer graphics and 3D computer vision, where they allow affine transformations and, in general, projective transformations to be easily represented by a matrix.

Boyd Patterson described the lines in this plane: [2]

The locus of points whose coordinates satisfy a homogeneous linear equation with complex coefficients
is a straight line and the line is real or imaginary according as the coefficients of its equation are or are not proportional to three real numbers.

Felix Klein described imaginary geometrical structures: "We will characterize a geometric structure as imaginary if its coordinates are not all real.: [3]

Felix Klein German mathematician, author of the Erlangen Program

Christian Felix Klein was a German mathematician and mathematics educator, known for his work with group theory, complex analysis, non-Euclidean geometry, and on the associations between geometry and group theory. His 1872 Erlangen Program, classifying geometries by their basic symmetry groups, was an influential synthesis of much of the mathematics of the time.

According to Hatton: [4]

The locus of the double points (imaginary) of the overlapping involutions in which an overlapping involution pencil (real) is cut by real transversals is a pair of imaginary straight lines.

Hatton continues,

Hence it follows that an imaginary straight line is determined by an imaginary point, which is a double point of an involution, and a real point, the vertex of the involution pencil.

See also

In geometry, in the context of a real geometric space extended to a complex projective space, an imaginary point is a point not contained in the embedded space.

An imaginary number is a complex number that can be written as a real number multiplied by the imaginary unit i, which is defined by its property i2 = −1. The square of an imaginary number bi is −b2. For example, 5i is an imaginary number, and its square is −25. Zero is considered to be both real and imaginary.

Related Research Articles

Analytic geometry study of geometry using a coordinate system

In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.

Sphere round geometrical and circular object in three-dimensional space; special case of spheroid

A sphere is a perfectly round geometrical object in three-dimensional space that is the surface of a completely round ball.

Plane (geometry) Flat, two-dimensional surface

In mathematics, a plane is a flat, two-dimensional surface that extends infinitely far. A plane is the two-dimensional analogue of a point, a line and three-dimensional space. Planes can arise as subspaces of some higher-dimensional space, as with a room's walls extended infinitely far, or they may enjoy an independent existence in their own right, as in the setting of Euclidean geometry.

Algebraic curve algebraic variety of dimension one

In mathematics, a plane real algebraic curve is the set of points on the Euclidean plane whose coordinates are zeros of some polynomial in two variables. More generally an algebraic curve is similar but may be embedded in a higher dimensional space or defined over some more general field.

In geometry, an incidence relation is a heterogeneous relation that captures the idea being expressed when phrases such as "a point lies on a line" or "a line is contained in a plane" are used. The most basic incidence relation is that between a point, P, and a line, l, sometimes denoted P I l. If P I l the pair (P, l) is called a flag. There are many expressions used in common language to describe incidence but the term "incidence" is preferred because it does not have the additional connotations that these other terms have, and it can be used in a symmetric manner. Statements such as "line l1 intersects line l2" are also statements about incidence relations, but in this case, it is because this is a shorthand way of saying that "there exists a point P that is incident with both line l1 and line l2". When one type of object can be thought of as a set of the other type of object then an incidence relation may be viewed as containment.

In geometry, a degenerate conic is a conic that fails to be an irreducible curve. This means that the defining equation is factorable over the complex numbers as the product of two linear polynomials.

In mathematics, a projective line is, roughly speaking, the extension of a usual line by a point called a point at infinity. The statement and the proof of many theorems of geometry are simplified by the resultant elimination of special cases; for example, two distinct projective lines in a projective plane meet in exactly one point.

Real projective plane

In mathematics, the real projective plane is an example of a compact non-orientable two-dimensional manifold; in other words, a one-sided surface. It cannot be embedded in standard three-dimensional space without intersecting itself. It has basic applications to geometry, since the common construction of the real projective plane is as the space of lines in R3 passing through the origin.

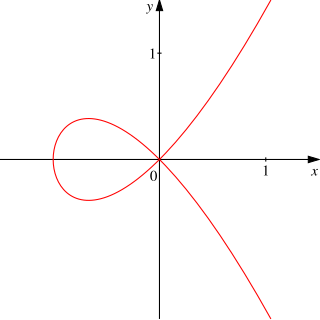
Cubic plane curve curve defined in projective plane by a cubic homogeneous polynomial

In mathematics, a cubic plane curve is a plane algebraic curve C defined by a cubic equation

Linear system of divisors

In algebraic geometry, a linear system of divisors is an algebraic generalization of the geometric notion of a family of curves; the dimension of the linear system corresponds to the number of parameters of the family.

Apollonian circles

Apollonian circles are two families of circles such that every circle in the first family intersects every circle in the second family orthogonally, and vice versa. These circles form the basis for bipolar coordinates. They were discovered by Apollonius of Perga, a renowned Greek geometer.

In projective geometry, the circular points at infinity are two special points at infinity in the complex projective plane that are contained in the complexification of every real circle.

In the geometry of quadratic forms, an isotropic line or null line is a line for which the quadratic form applied to the displacement vector between any pair of its points is zero. An isotropic line occurs only with an isotropic quadratic form, and never with a definite quadratic form.

In algebraic geometry an imaginary curve is an algebraic curve which does not contain any real points.

Conic section curve obtained as the intersection of a cone with a plane; are formed by cross-sections of a cone at various different angles

In mathematics, a conic section is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse. The circle is a special case of the ellipse, and is of sufficient interest in its own right that it was sometimes called a fourth type of conic section. The conic sections have been studied by the ancient Greek mathematicians with this work culminating around 200 BC, when Apollonius of Perga undertook a systematic study of their properties.

In geometry, line coordinates are used to specify the position of a line just as point coordinates are used to specify the position of a point.

In Euclidean and projective geometry, just as two (distinct) points determine a line, five points determine a conic. There are additional subtleties for conics that do not exist for lines, and thus the statement and its proof for conics are both more technical than for lines.

Cayley–Klein metric

In mathematics, a Cayley–Klein metric is a metric on the complement of a fixed quadric in a projective space which is defined using a cross-ratio. The construction originated with Arthur Cayley's essay "On the theory of distance" where he calls the quadric the absolute. The construction was developed in further detail by Felix Klein in papers in 1871 and 1873, and subsequent books and papers. The Cayley–Klein metrics are a unifying idea in geometry since the method is used to provide metrics in hyperbolic geometry, elliptic geometry, and Euclidean geometry. The field of non-Euclidean geometry rests largely on the footing provided by Cayley–Klein metrics.

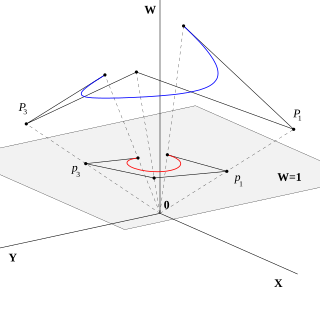
Conformal geometric algebra (CGA) is the geometric algebra constructed over the resultant space of a map from points in an n-dimensional base space ℝp,q to null vectors in ℝp+1,q+1. This allows operations on the base space, including reflections, rotations and translations to be represented using versors of the geometric algebra; and it is found that points, lines, planes, circles and spheres gain particularly natural and computationally amenable representations.

References

  1. ↑ Patterson, B. C. (1941), "The inversive plane", The American Mathematical Monthly, 48: 589–599, doi:10.2307/2303867, MR   0006034 .
  2. ↑ Patterson 590
  3. ↑ Klein 1928 p 46
  4. ↑ Hatton 1929 page 13, Definition 4

Cambridge University Press (CUP) is the publishing business of the University of Cambridge. Granted letters patent by King Henry VIII in 1534, it is the world's oldest publishing house and the second-largest university press in the world. It also holds letters patent as the Queen's Printer.

Julius Springer was a German publisher who founded the academic publishing house Springer Science+Business Media.

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.