In mathematics, the branch of real analysis studies the behavior of real numbers, sequences and series of real numbers, and real functions. Some particular properties of real-valued sequences and functions that real analysis studies include convergence, limits, continuity, smoothness, differentiability and integrability.

In mathematical analysis, the Dirac delta function, also known as the unit impulse, is a generalized function on the real numbers, whose value is zero everywhere except at zero, and whose integral over the entire real line is equal to one. Since there is no function having this property, modelling the delta "function" rigorously involves the use of limits or, as is common in mathematics, measure theory and the theory of distributions.
In mathematics, the Banach fixed-point theorem is an important tool in the theory of metric spaces; it guarantees the existence and uniqueness of fixed points of certain self-maps of metric spaces, and provides a constructive method to find those fixed points. It can be understood as an abstract formulation of Picard's method of successive approximations. The theorem is named after Stefan Banach (1892–1945) who first stated it in 1922.
In mathematics, the limit of a function is a fundamental concept in calculus and analysis concerning the behavior of that function near a particular input which may or may not be in the domain of the function.

In mathematics, the limit of a sequence is the value that the terms of a sequence "tend to", and is often denoted using the symbol. If such a limit exists, the sequence is called convergent. A sequence that does not converge is said to be divergent. The limit of a sequence is said to be the fundamental notion on which the whole of mathematical analysis ultimately rests.
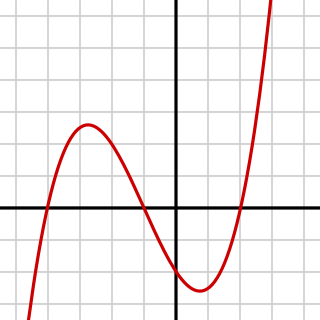
In mathematics, a differentiable function of one real variable is a function whose derivative exists at each point in its domain. In other words, the graph of a differentiable function has a non-vertical tangent line at each interior point in its domain. A differentiable function is smooth and does not contain any break, angle, or cusp.
In mathematics, the Cauchy principal value, named after Augustin-Louis Cauchy, is a method for assigning values to certain improper integrals which would otherwise be undefined. In this method, a singularity on an integral interval is avoided by limiting the integral interval to the non singular domain.
The Arzelà–Ascoli theorem is a fundamental result of mathematical analysis giving necessary and sufficient conditions to decide whether every sequence of a given family of real-valued continuous functions defined on a closed and bounded interval has a uniformly convergent subsequence. The main condition is the equicontinuity of the family of functions. The theorem is the basis of many proofs in mathematics, including that of the Peano existence theorem in the theory of ordinary differential equations, Montel's theorem in complex analysis, and the Peter–Weyl theorem in harmonic analysis and various results concerning compactness of integral operators.
In mathematics, deformation theory is the study of infinitesimal conditions associated with varying a solution P of a problem to slightly different solutions Pε, where ε is a small number, or a vector of small quantities. The infinitesimal conditions are the result of applying the approach of differential calculus to solving a problem with constraints. The name is an analogy to non-rigid structures that deform slightly to accommodate external forces.
In probability theory and related fields, Malliavin calculus is a set of mathematical techniques and ideas that extend the mathematical field of calculus of variations from deterministic functions to stochastic processes. In particular, it allows the computation of derivatives of random variables. Malliavin calculus is also called the stochastic calculus of variations. P. Malliavin first initiated the calculus on infinite dimensional space. Then, the significant contributors such as S. Kusuoka, D. Stroock, J-M. Bismut, Shinzo Watanabe, I. Shigekawa, and so on finally completed the foundations.
In mathematical analysis, and applications in geometry, applied mathematics, engineering, and natural sciences, a function of a real variable is a function whose domain is the real numbers , or a subset of that contains an interval of positive length. Most real functions that are considered and studied are differentiable in some interval. The most widely considered such functions are the real functions, which are the real-valued functions of a real variable, that is, the functions of a real variable whose codomain is the set of real numbers.
In measure theory, an area of mathematics, Egorov's theorem establishes a condition for the uniform convergence of a pointwise convergent sequence of measurable functions. It is also named Severini–Egoroff theorem or Severini–Egorov theorem, after Carlo Severini, an Italian mathematician, and Dmitri Egorov, a Russian physicist and geometer, who published independent proofs respectively in 1910 and 1911.
In mathematics, more specifically in dynamical systems, the method of averaging exploits systems containing time-scales separation: a fast oscillationversus a slow drift. It suggests that we perform an averaging over a given amount of time in order to iron out the fast oscillations and observe the qualitative behavior from the resulting dynamics. The approximated solution holds under finite time inversely proportional to the parameter denoting the slow time scale. It turns out to be a customary problem where there exists the trade off between how good is the approximated solution balanced by how much time it holds to be close to the original solution.
In mathematical analysis, the Russo–Vallois integral is an extension to stochastic processes of the classical Riemann–Stieltjes integral
In mathematical analysis, the Pólya–Szegő inequality states that the Sobolev energy of a function in a Sobolev space does not increase under symmetric decreasing rearrangement. The inequality is named after the mathematicians George Pólya and Gábor Szegő.
In mathematics, the Kardar–Parisi–Zhang (KPZ) equation is a non-linear stochastic partial differential equation, introduced by Mehran Kardar, Giorgio Parisi, and Yi-Cheng Zhang in 1986. It describes the temporal change of a height field with spatial coordinate and time coordinate :
In mathematics, the Kodaira–Spencer map, introduced by Kunihiko Kodaira and Donald C. Spencer, is a map associated to a deformation of a scheme or complex manifold X, taking a tangent space of a point of the deformation space to the first cohomology group of the sheaf of vector fields on X.
In additive number theory, an area of mathematics, the Erdős–Tetali theorem is an existence theorem concerning economical additive bases of every order. More specifically, it states that for every fixed integer , there exists a subset of the natural numbers satisfying
In mathematics, the field of logarithmic-exponential transseries is a non-Archimedean ordered differential field which extends comparability of asymptotic growth rates of elementary nontrigonometric functions to a much broader class of objects. Each log-exp transseries represents a formal asymptotic behavior, and it can be manipulated formally, and when it converges, corresponds to actual behavior. Transseries can also be convenient for representing functions. Through their inclusion of exponentiation and logarithms, transseries are a strong generalization of the power series at infinity and other similar asymptotic expansions.
In mathematics, the limiting absorption principle (LAP) is a concept from operator theory and scattering theory that consists of choosing the "correct" resolvent of a linear operator at the essential spectrum based on the behavior of the resolvent near the essential spectrum. The term is often used to indicate that the resolvent, when considered not in the original space (which is usually the space), but in certain weighted spaces (usually , see below), has a limit as the spectral parameter approaches the essential spectrum. This concept developed from the idea of introducing complex parameter into the Helmholtz equation for selecting a particular solution. This idea is credited to Vladimir Ignatowski, who was considering the propagation and absorption of the electromagnetic waves in a wire. It is closely related to the Sommerfeld radiation condition and the limiting amplitude principle (1948). The terminology – both the limiting absorption principle and the limiting amplitude principle – was introduced by Aleksei Sveshnikov.



























