Related Research Articles

In astronomy, a double planet is a binary satellite system where both objects are planets, or planetary-mass objects, and whose joint barycenter is external to both planetary bodies.

In celestial mechanics, the Lagrange points are points of equilibrium for small-mass objects under the gravitational influence of two massive orbiting bodies. Mathematically, this involves the solution of the restricted three-body problem.
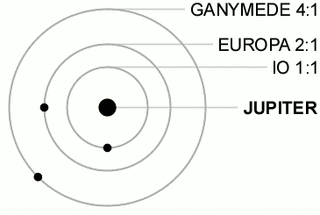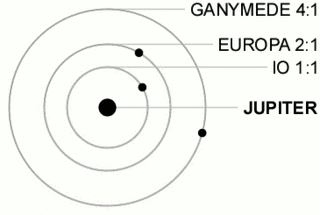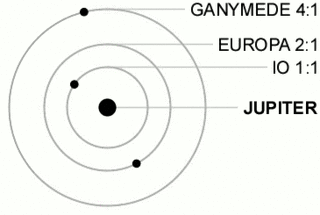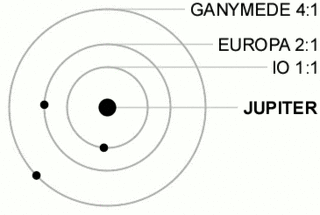
In celestial mechanics, orbital resonance occurs when orbiting bodies exert regular, periodic gravitational influence on each other, usually because their orbital periods are related by a ratio of small integers. Most commonly, this relationship is found between a pair of objects. The physical principle behind orbital resonance is similar in concept to pushing a child on a swing, whereby the orbit and the swing both have a natural frequency, and the body doing the "pushing" will act in periodic repetition to have a cumulative effect on the motion. Orbital resonances greatly enhance the mutual gravitational influence of the bodies. In most cases, this results in an unstable interaction, in which the bodies exchange momentum and shift orbits until the resonance no longer exists. Under some circumstances, a resonant system can be self-correcting and thus stable. Examples are the 1:2:4 resonance of Jupiter's moons Ganymede, Europa and Io, and the 2:3 resonance between Neptune and Pluto. Unstable resonances with Saturn's inner moons give rise to gaps in the rings of Saturn. The special case of 1:1 resonance between bodies with similar orbital radii causes large planetary system bodies to eject most other bodies sharing their orbits; this is part of the much more extensive process of clearing the neighbourhood, an effect that is used in the current definition of a planet.

The Solar System is the gravitationally bound system of the Sun and the objects that orbit it. It formed about 4.6 billion years ago when a dense region of a molecular cloud collapsed, forming the Sun and a protoplanetary disc. The Sun is a typical star that maintains a balanced equilibrium by the fusion of hydrogen into helium at its core, releasing this energy from its outer photosphere. Astronomers classify it as a G-type main-sequence star.

Tidal acceleration is an effect of the tidal forces between an orbiting natural satellite and the primary planet that it orbits. The acceleration causes a gradual recession of a satellite in a prograde orbit, and a corresponding slowdown of the primary's rotation. The process eventually leads to tidal locking, usually of the smaller body first, and later the larger body. The Earth–Moon system is the best-studied case.
Celestial mechanics is the branch of astronomy that deals with the motions of objects in outer space. Historically, celestial mechanics applies principles of physics to astronomical objects, such as stars and planets, to produce ephemeris data.
The orbital period is the amount of time a given astronomical object takes to complete one orbit around another object. In astronomy, it usually applies to planets or asteroids orbiting the Sun, moons orbiting planets, exoplanets orbiting other stars, or binary stars. It may also refer to the time it takes a satellite orbiting a planet or moon to complete one orbit.
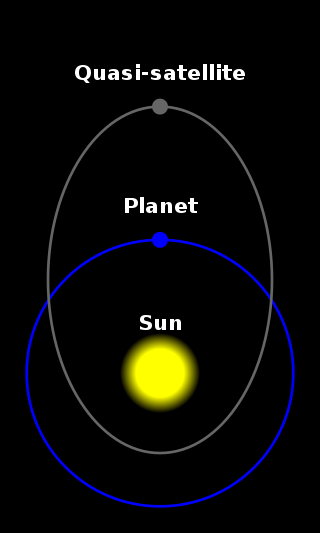
A quasi-satellite is an object in a specific type of co-orbital configuration with a planet where the object stays close to that planet over many orbital periods.

The Hill sphere is a common model for the calculation of a gravitational sphere of influence. It is the most commonly used model to calculate the spatial extent of gravitational influence of an astronomical body (m) in which it dominates over the gravitational influence of other bodies, particularly a primary (M). It is sometimes confused with other models of gravitational influence, such as the Laplace sphere or being named the Roche sphere, the latter causing confusion with the Roche limit. It was defined by the American astronomer George William Hill, based on the work of the French astronomer Édouard Roche.
A sphere of influence (SOI) in astrodynamics and astronomy is the oblate spheroid-shaped region where a particular celestial body exerts the main gravitational influence on an orbiting object. This is usually used to describe the areas in the Solar System where planets dominate the orbits of surrounding objects such as moons, despite the presence of the much more massive but distant Sun.

In celestial mechanics, a horseshoe orbit is a type of co-orbital motion of a small orbiting body relative to a larger orbiting body. The osculating (instantaneous) orbital period of the smaller body remains very near that of the larger body, and if its orbit is a little more eccentric than that of the larger body, during every period it appears to trace an ellipse around a point on the larger object's orbit. However, the loop is not closed but drifts forward or backward so that the point it circles will appear to move smoothly along the larger body's orbit over a long period of time. When the object approaches the larger body closely at either end of its trajectory, its apparent direction changes. Over an entire cycle the center traces the outline of a horseshoe, with the larger body between the 'horns'.

An exomoon or extrasolar moon is a natural satellite that orbits an exoplanet or other non-stellar extrasolar body.

There is evidence that the formation of the Solar System began about 4.6 billion years ago with the gravitational collapse of a small part of a giant molecular cloud. Most of the collapsing mass collected in the center, forming the Sun, while the rest flattened into a protoplanetary disk out of which the planets, moons, asteroids, and other small Solar System bodies formed.
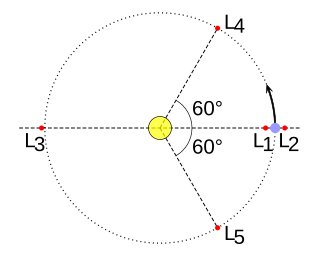
In astronomy, a trojan is a small celestial body (mostly asteroids) that shares the orbit of a larger body, remaining in a stable orbit approximately 60° ahead of or behind the main body near one of its Lagrangian points L4 and L5. Trojans can share the orbits of planets or of large moons.
In astronomy, a co-orbital configuration is a configuration of two or more astronomical objects orbiting at the same, or very similar, distance from their primary; i.e., they are in a 1:1 mean-motion resonance..

A galactic tide is a tidal force experienced by objects subject to the gravitational field of a galaxy such as the Milky Way. Particular areas of interest concerning galactic tides include galactic collisions, the disruption of dwarf or satellite galaxies, and the Milky Way's tidal effect on the Oort cloud of the Solar System.

The history of scientific thought about the formation and evolution of the Solar System began with the Copernican Revolution. The first recorded use of the term "Solar System" dates from 1704. Since the seventeenth century, philosophers and scientists have been forming hypotheses concerning the origins of the Solar System and the Moon and attempting to predict how the Solar System would change in the future. René Descartes was the first to hypothesize on the beginning of the Solar System; however, more scientists joined the discussion in the eighteenth century, forming the groundwork for later hypotheses on the topic. Later, particularly in the twentieth century, a variety of hypotheses began to build up, including the now–commonly accepted nebular hypothesis.

Retrograde motion in astronomy is, in general, orbital or rotational motion of an object in the direction opposite the rotation of its primary, that is, the central object. It may also describe other motions such as precession or nutation of an object's rotational axis. Prograde or direct motion is more normal motion in the same direction as the primary rotates. However, "retrograde" and "prograde" can also refer to an object other than the primary if so described. The direction of rotation is determined by an inertial frame of reference, such as distant fixed stars.
In astronomy, planetary mass is a measure of the mass of a planet-like astronomical object. Within the Solar System, planets are usually measured in the astronomical system of units, where the unit of mass is the solar mass (M☉), the mass of the Sun. In the study of extrasolar planets, the unit of measure is typically the mass of Jupiter (MJ) for large gas giant planets, and the mass of Earth (ME) for smaller rocky terrestrial planets.
This glossary of astronomy is a list of definitions of terms and concepts relevant to astronomy and cosmology, their sub-disciplines, and related fields. Astronomy is concerned with the study of celestial objects and phenomena that originate outside the atmosphere of Earth. The field of astronomy features an extensive vocabulary and a significant amount of jargon.
References
- ↑ Monthly Notices of the Royal Astronomical Society, Volume 391, Issue 2, December 2008, Pages 675–684, https://doi.org/10.1111/j.1365-2966.2008.13833.x
- ↑ D Souami, J Cresson, C Biernacki, F Pierret, On the local and global properties of gravitational spheres of influence, Monthly Notices of the Royal Astronomical Society, Volume 496, Issue 4, August 2020, Pages 4287–4297, https://doi.org/10.1093/mnras/staa1520
- ↑ Roy, A.E. (2004). Orbital Motion (4th ed.). CRC Press. https://doi.org/10.1201/9780367806620