Related Research Articles

In the part of mathematics referred to as topology, a surface is a two-dimensional manifold. Some surfaces arise as the boundaries of three-dimensional solids; for example, the sphere is the boundary of the solid ball. Other surfaces arise as graphs of functions of two variables; see the figure at right. However, surfaces can also be defined abstractly, without reference to any ambient space. For example, the Klein bottle is a surface that cannot be embedded in three-dimensional Euclidean space.

In mathematics, genus has a few different, but closely related, meanings. The most common concept, the genus of an (orientable) surface, is the number of "holes" it has, so that a sphere has genus 0 and a torus has genus 1. This is made more precise below.
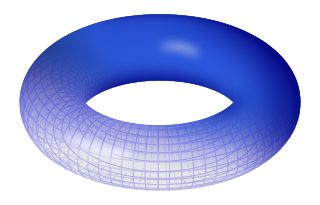
In geometry, a torus is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle.
In mathematics, homology is a general way of associating a sequence of algebraic objects such as abelian groups or modules to other mathematical objects such as topological spaces. Homology groups were originally defined in algebraic topology. Similar constructions are available in a wide variety of other contexts, such as abstract algebra, groups, Lie algebras, Galois theory, and algebraic geometry.

In mathematics, particularly in complex analysis, a Riemann surface is a one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed versions of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or several sheets glued together.

Charles-Augustin de Coulomb was a French military engineer and physicist. He is best known as the eponymous discoverer of what is now called Coulomb's law, the description of the electrostatic force of attraction and repulsion, though he also did important work on friction.

In mathematics, and particularly topology, a fiber bundle is a space that is locally a product space, but globally may have a different topological structure. Specifically, the similarity between a space and a product space is defined using a continuous surjective map
In topology, a topological space is called simply connected if it is path-connected and every path between two points can be continuously transformed into any other such path while preserving the two endpoints in question. The fundamental group of a topological space is an indicator of the failure for the space to be simply connected: a path-connected topological space is simply connected if and only if its fundamental group is trivial.
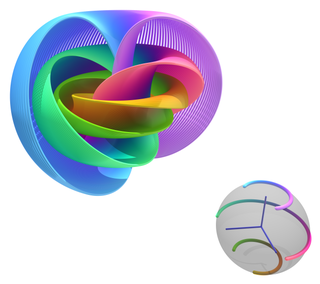
In the mathematical field of differential topology, the Hopf fibration describes a 3-sphere in terms of circles and an ordinary sphere. Discovered by Heinz Hopf in 1931, it is an influential early example of a fiber bundle. Technically, Hopf found a many-to-one continuous function from the 3-sphere onto the 2-sphere such that each distinct point of the 2-sphere is mapped to from a distinct great circle of the 3-sphere. Thus the 3-sphere is composed of fibers, where each fiber is a circle — one for each point of the 2-sphere.
In topology, a branch of mathematics, a Dehn surgery, named after Max Dehn, is a construction used to modify 3-manifolds. The process takes as input a 3-manifold together with a link. It is often conceptualized as two steps: drilling then filling.

In mathematics, a manifold is a topological space that locally resembles Euclidean space near each point. More precisely, each point of an n-dimensional manifold has a neighborhood that is homeomorphic to the Euclidean space of dimension n. In this more precise terminology, a manifold is referred to as an n-manifold.

Hervé Le Tellier is a French writer and linguist, and a member of the international literary group Oulipo. He is its fourth president. Other notable members have included Raymond Queneau, Georges Perec, Italo Calvino, Jacques Roubaud, Jean Lescure and Harry Mathews.

Béla Kerékjártó was a Hungarian mathematician who wrote numerous articles on topology.
Gerald Messadié was a French scientific journalist, essayist and novelist. His work comprised historical novels, biographies, essays on the history of religions, and some science fiction work where esoterism takes a large place.
In mathematics, the Appell–Humbert theorem describes the line bundles on a complex torus or complex abelian variety. It was proved for 2-dimensional tori by Appell (1891) and Humbert (1893), and in general by Lefschetz (1921)

The canton of Lattes is an administrative division of the Hérault department, southern France. Its borders were modified at the French canton reorganisation which came into effect in March 2015. Its seat is in Lattes.

In mathematics, a finite subdivision rule is a recursive way of dividing a polygon or other two-dimensional shape into smaller and smaller pieces. Subdivision rules in a sense are generalizations of regular geometric fractals. Instead of repeating exactly the same design over and over, they have slight variations in each stage, allowing a richer structure while maintaining the elegant style of fractals. Subdivision rules have been used in architecture, biology, and computer science, as well as in the study of hyperbolic manifolds. Substitution tilings are a well-studied type of subdivision rule.
The Prix Maison de la Presse is an annual French literary prize, established in 1970 by the Syndicat national des dépositaires de presse (SNDP) and Gabriel Cantin. Until 2005 it was known as Prix des Maisons de la Presse and given out in the two categories Novel (Roman) and Non-Fiction (Document), after which the name was changed and the categories merged into one.
Maurice Denuzière is a French journalist and writer.
Samuel Lattès was a French mathematician.
References
- Lattès, S (1918), "Sur l'itération des substitutions rationnelles et les fonctions de Poincaré", Les Comptes rendus de l'Académie des sciences , 166: 26–28
- Milnor, John Willard (2006), "On Lattès maps", Dynamics on the Riemann sphere, Eur. Math. Soc., pp. 9–43, MR 2348953