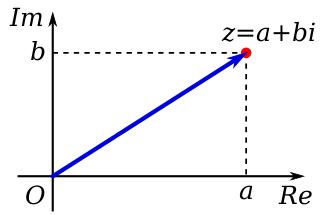
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted i, called the imaginary unit and satisfying the equation ; every complex number can be expressed in the form , where a and b are real numbers. Because no real number satisfies the above equation, i was called an imaginary number by René Descartes. For the complex number , a is called the real part, and b is called the imaginary part. The set of complex numbers is denoted by either of the symbols or C. Despite the historical nomenclature "imaginary", complex numbers are regarded in the mathematical sciences as just as "real" as the real numbers and are fundamental in many aspects of the scientific description of the natural world.

The exponential function is a mathematical function denoted by or . Unless otherwise specified, the term generally refers to the positive-valued function of a real variable, although it can be extended to the complex numbers or generalized to other mathematical objects like matrices or Lie algebras. The exponential function originated from the notion of exponentiation, but modern definitions allow it to be rigorously extended to all real arguments, including irrational numbers. Its ubiquitous occurrence in pure and applied mathematics led mathematician Walter Rudin to opine that the exponential function is "the most important function in mathematics".

In mathematics, the gamma function is one commonly used extension of the factorial function to complex numbers. The gamma function is defined for all complex numbers except the non-positive integers. For every positive integer n,

An integer is the number zero (0), a positive natural number or a negative integer with a minus sign. The negative numbers are the additive inverses of the corresponding positive numbers. In the language of mathematics, the set of integers is often denoted by the boldface Z or blackboard bold .

In mathematics, the logarithm is the inverse function to exponentiation. That means the logarithm of a number x to the base b is the exponent to which b must be raised, to produce x. For example, since 1000 = 103, the logarithm base 10 of 1000 is 3, or log10 (1000) = 3. The logarithm of x to base b is denoted as logb (x), or without parentheses, logb x, or even without the explicit base, log x, when no confusion is possible, or when the base does not matter such as in big O notation.

In mathematics, modular arithmetic is a system of arithmetic for integers, where numbers "wrap around" when reaching a certain value, called the modulus. The modern approach to modular arithmetic was developed by Carl Friedrich Gauss in his book Disquisitiones Arithmeticae, published in 1801.

In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is
The number π is a mathematical constant that is the ratio of a circle's circumference to its diameter, approximately equal to 3.14159. The number π appears in many formulas across mathematics and physics. It is an irrational number, meaning that it cannot be expressed exactly as a ratio of two integers, although fractions such as are commonly used to approximate it. Consequently, its decimal representation never ends, nor enters a permanently repeating pattern. It is a transcendental number, meaning that it cannot be a solution of an equation involving only sums, products, powers, and integers. The transcendence of π implies that it is impossible to solve the ancient challenge of squaring the circle with a compass and straightedge. The decimal digits of π appear to be randomly distributed, but no proof of this conjecture has been found.

Z is the 26th and last letter of the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its usual names in English are zed and zee, with an occasional archaic variant izzard.
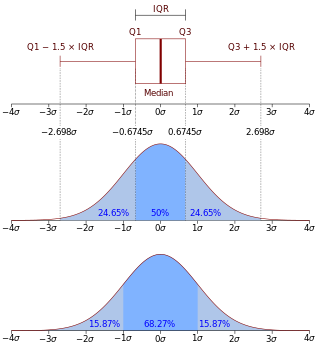
In probability theory, a probability density function (PDF), or density of a continuous random variable, is a function whose value at any given sample in the sample space can be interpreted as providing a relative likelihood that the value of the random variable would be equal to that sample. Probability density is the probability per unit length, in other words, while the absolute likelihood for a continuous random variable to take on any particular value is 0, the value of the PDF at two different samples can be used to infer, in any particular draw of the random variable, how much more likely it is that the random variable would be close to one sample compared to the other sample.
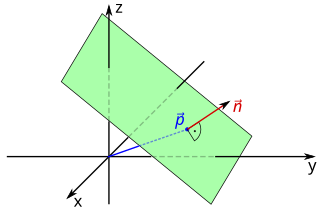
In mathematics, a plane is a Euclidean (flat), two-dimensional surface that extends indefinitely. A plane is the two-dimensional analogue of a point, a line and three-dimensional space. Planes can arise as subspaces of some higher-dimensional space, as with one of a room's walls, infinitely extended, or they may enjoy an independent existence in their own right, as in the setting of two-dimensional Euclidean geometry. Sometimes the word plane is used more generally to describe a two-dimensional surface, for example the hyperbolic plane and elliptic plane.

Shawn Corey Carter, known professionally as Jay-Z, is an American rapper, record producer, entrepreneur, and founder of Manhattan-based conglomerate talent and entertainment agency Roc Nation. He is regarded as one of the greatest rappers of all time. He was the CEO of Def Jam Recordings and he has been central to the creative and commercial success of artists including Kanye West, Rihanna, and J. Cole.

In statistics, the standard score is the number of standard deviations by which the value of a raw score is above or below the mean value of what is being observed or measured. Raw scores above the mean have positive standard scores, while those below the mean have negative standard scores.
In linear algebra, a rotation matrix is a transformation matrix that is used to perform a rotation in Euclidean space. For example, using the convention below, the matrix
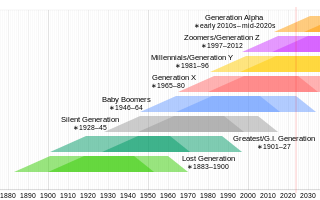
Generation Z, colloquially known as zoomers, is the demographic cohort succeeding Millennials and preceding Generation Alpha. Researchers and popular media use the mid-to-late 1990s as starting birth years and the early 2010s as ending birth years. Most members of Generation Z are children of Generation X.

The Kawasaki Z1 is a four-cylinder, air-cooled, double-overhead camshaft, carbureted, chain-drive motorcycle introduced in 1972 by Kawasaki. Following the introduction of Honda's CB750 in 1968, the Z1 helped popularize the in-line, across-the-frame four-cylinder, a format that became known as the Universal Japanese Motorcycle or UJM.
Dragon Ball is a Japanese media franchise created by Akira Toriyama in 1984. The initial manga, written and illustrated by Toriyama, was serialized in Weekly Shōnen Jump from 1984 to 1995, with the 519 individual chapters collected into 42 tankōbon volumes by its publisher Shueisha. Dragon Ball was originally inspired by the classical 16th-century Chinese novel Journey to the West, combined with elements of Hong Kong martial arts films. The series follows the adventures of protagonist Son Goku from his childhood through adulthood as he trains in martial arts. He spends his childhood far from civilization until he meets a teen girl named Bulma, who encourages him to join her quest in exploring the world in search of the seven orbs known as the Dragon Balls, which summon a wish-granting dragon when gathered. Along his journey, Goku makes several other friends, becomes a family man, discovers his alien heritage, and battles a wide variety of villains, many of whom also seek the Dragon Balls.

World War Z is a 2013 American apocalyptic action horror film directed by Marc Forster, with a screenplay by Matthew Michael Carnahan, Drew Goddard, and Damon Lindelof, from a story by Carnahan and J. Michael Straczynski, based on the title of the 2006 novel of the same name by Max Brooks. It stars Brad Pitt as Gerry Lane, a former United Nations investigator who travels the world seeking a solution for a sudden zombie apocalypse. The ensemble supporting cast includes Mireille Enos, Daniella Kertesz, James Badge Dale, Ludi Boeken, Matthew Fox, Fana Mokoena, David Morse, Elyes Gabel, Peter Capaldi, Pierfrancesco Favino, Ruth Negga, and Moritz Bleibtreu.

Dragon Ball Z is a Japanese anime television series produced by Toei Animation. Part of the Dragon Ball media franchise, it is the sequel to the 1986 Dragon Ball anime series and adapts the latter 325 chapters of the original Dragon Ball manga series created by Akira Toriyama. The series aired in Japan on Fuji TV from April 1989 to January 1996 and was later dubbed for broadcast in at least 81 countries worldwide.

The Latin-script letter Z is one of several symbols painted on military vehicles of the Russian Armed Forces involved in the 2022 Russian invasion of Ukraine. It is speculated that the Z helps task forces distinguish themselves from other forces.
















