Related Research Articles

A structure is an arrangement and organization of interrelated elements in a material object or system, or the object or system so organized. Material structures include man-made objects such as buildings and machines and natural objects such as biological organisms, minerals and chemicals. Abstract structures include data structures in computer science and musical form. Types of structure include a hierarchy, a network featuring many-to-many links, or a lattice featuring connections between components that are neighbors in space.

The western blot, or western blotting, is a widely used analytical technique in molecular biology and immunogenetics to detect specific proteins in a sample of tissue homogenate or extract. Besides detecting the proteins, this technique is also utilized to visualize, distinguish, and quantify the different proteins in a complicated protein combination.

In continuum mechanics, stress is a physical quantity that describes forces present during deformation. For example, an object being pulled apart, such as a stretched elastic band, is subject to tensile stress and may undergo elongation. An object being pushed together, such as a crumpled sponge, is subject to compressive stress and may undergo shortening. The greater the force and the smaller the cross-sectional area of the body on which it acts, the greater the stress. Stress has dimension of force per area, with SI units of newtons per square meter (N/m2) or pascal (Pa).
Structural analysis is a branch of solid mechanics which uses simplified models for solids like bars, beams and shells for engineering decision making. Its main objective is to determine the effect of loads on physical structures and their components. In contrast to theory of elasticity, the models used in structural analysis are often differential equations in one spatial variable. Structures subject to this type of analysis include all that must withstand loads, such as buildings, bridges, aircraft and ships. Structural analysis uses ideas from applied mechanics, materials science and applied mathematics to compute a structure's deformations, internal forces, stresses, support reactions, velocity, accelerations, and stability. The results of the analysis are used to verify a structure's fitness for use, often precluding physical tests. Structural analysis is thus a key part of the engineering design of structures.
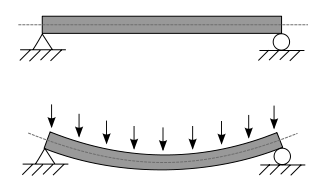
A beam is a structural element that primarily resists loads applied laterally across the beam's axis. Its mode of deflection is primarily by bending, as loads produce reaction forces at the beam's support points and internal bending moments, shear, stresses, strains, and deflections. Beams are characterized by their manner of support, profile, equilibrium conditions, length, and material.

In structural engineering, buckling is the sudden change in shape (deformation) of a structural component under load, such as the bowing of a column under compression or the wrinkling of a plate under shear. If a structure is subjected to a gradually increasing load, when the load reaches a critical level, a member may suddenly change shape and the structure and component is said to have buckled. Euler's critical load and Johnson's parabolic formula are used to determine the buckling stress of a column.
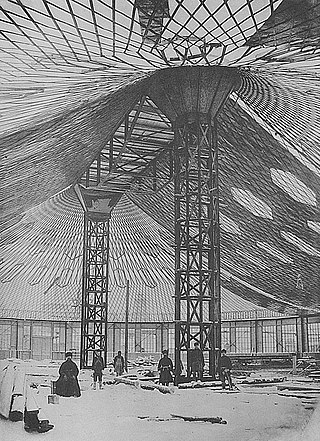
In structural engineering, a tensile structure is a construction of elements carrying only tension and no compression or bending. The term tensile should not be confused with tensegrity, which is a structural form with both tension and compression elements. Tensile structures are the most common type of thin-shell structures.

In applied mechanics, bending characterizes the behavior of a slender structural element subjected to an external load applied perpendicularly to a longitudinal axis of the element.

In materials science, photoelasticity describes changes in the optical properties of a material under mechanical deformation. It is a property of all dielectric media and is often used to experimentally determine the stress distribution in a material.
The strength of ships is a topic of key interest to naval architects and shipbuilders. Ships which are built too strong are heavy, slow, and cost extra money to build and operate since they weigh more, whilst ships which are built too weakly suffer from minor hull damage and in some extreme cases catastrophic failure and sinking.
The finite element method (FEM) is a powerful technique originally developed for numerical solution of complex problems in structural mechanics, and it remains the method of choice for complex systems. In the FEM, the structural system is modeled by a set of appropriate finite elements interconnected at discrete points called nodes. Elements may have physical properties such as thickness, coefficient of thermal expansion, density, Young's modulus, shear modulus and Poisson's ratio.

In materials science, a sandwich-structured composite is a special class of composite materials that is fabricated by attaching two thin-but-stiff skins to a lightweight-but-thick core. The core material is normally of low strength, but its greater thickness provides the sandwich composite with high bending stiffness with overall low density.

Structural mechanics or mechanics of structures is the computation of deformations, deflections, and internal forces or stresses within structures, either for design or for performance evaluation of existing structures. It is one subset of structural analysis. Structural mechanics analysis needs input data such as structural loads, the structure's geometric representation and support conditions, and the materials' properties. Output quantities may include support reactions, stresses and displacements. Advanced structural mechanics may include the effects of stability and non-linear behaviors.
Lipid bilayer mechanics is the study of the physical material properties of lipid bilayers, classifying bilayer behavior with stress and strain rather than biochemical interactions. Local point deformations such as membrane protein interactions are typically modelled with the complex theory of biological liquid crystals but the mechanical properties of a homogeneous bilayer are often characterized in terms of only three mechanical elastic moduli: the area expansion modulus Ka, a bending modulus Kb and an edge energy . For fluid bilayers the shear modulus is by definition zero, as the free rearrangement of molecules within plane means that the structure will not support shear stresses. These mechanical properties affect several membrane-mediated biological processes. In particular, the values of Ka and Kb affect the ability of proteins and small molecules to insert into the bilayer. Bilayer mechanical properties have also been shown to alter the function of mechanically activated ion channels.
A plate is a structural element which is characterized by a three-dimensional solid whose thickness is very small when compared with other dimensions.

A shell is a three-dimensional solid structural element whose thickness is very small compared to its other dimensions. It is characterized in structural terms by mid-plane stress which is both coplanar and normal to the surface. A shell can be derived from a plate in two steps: by initially forming the middle surface as a singly or doubly curved surface, then by applying loads which are coplanar to the plate's plane thus generating significant stresses. Materials range from concrete to fabric.
Stress resultants are simplified representations of the stress state in structural elements such as beams, plates, or shells. The geometry of typical structural elements allows the internal stress state to be simplified because of the existence of a "thickness'" direction in which the size of the element is much smaller than in other directions. As a consequence the three traction components that vary from point to point in a cross-section can be replaced with a set of resultant forces and resultant moments. These are the stress resultants that may be used to determine the detailed stress state in the structural element. A three-dimensional problem can then be reduced to a one-dimensional problem or a two-dimensional problem.
This glossary of structural engineering terms pertains specifically to structural engineering and its sub-disciplines. Please see Glossary of engineering for a broad overview of the major concepts of engineering.
Variational Asymptotic Method (VAM) is a powerful mathematical approach to simplify the process of finding stationary points for a described functional by taking advantage of small parameters. VAM is the synergy of variational principles and asymptotic approaches. Variational principles are applied to the defined functional as well as the asymptotes are applied to the same functional instead of applying on differential equations which is more prone error. This methodology is applicable for a whole range of physics problems, where the problem has to be defined in a variational form and should be able to identify the small parameters within the problem definition. In other words, VAM can be applicable where the functional is so complex in determining the stationary points either by analytical or by computationally expensive numerical analysis with an advantage of small parameters. Thus, approximate stationary points in the functional can be utilized to obtain the original functional.
Mecway is a finite-element analysis (FEA) application for Windows. which focuses on mechanical and thermal simulation. For thermal simulation, it allows convection, radiation boundary conditions, and temperature dependent thermal properties. Structural (mechanical) analysis capabilities are linear static, non-linear static, multi-body contact, dynamic, composite materials, modal analysis and buckling. There is also acoustic modal analysis.
References
- ↑ Wilhelm Flügge, Stresses in Shells (1973) 2nd edition, Preface, p. iv. ISBN 978-3-642-88291-3. Originally published in German as Statik und Dynamik der Schalen (1937).