Lambda calculus is a formal system in mathematical logic for expressing computation based on function abstraction and application using variable binding and substitution. Untyped lambda calculus, the topic of this article, is a universal model of computation that can be used to simulate any Turing machine. It was introduced by the mathematician Alonzo Church in the 1930s as part of his research into the foundations of mathematics. In 1936, Church found a formulation which was logically consistent, and documented it in 1940.
In mathematical logic and computer science, a general recursive function, partial recursive function, or μ-recursive function is a partial function from natural numbers to natural numbers that is "computable" in an intuitive sense – as well as in a formal one. If the function is total, it is also called a total recursive function. In computability theory, it is shown that the μ-recursive functions are precisely the functions that can be computed by Turing machines. The μ-recursive functions are closely related to primitive recursive functions, and their inductive definition (below) builds upon that of the primitive recursive functions. However, not every total recursive function is a primitive recursive function—the most famous example is the Ackermann function.

In probability theory and statistics, the exponential distribution or negative exponential distribution is the probability distribution of the distance between events in a Poisson point process, i.e., a process in which events occur continuously and independently at a constant average rate; the distance parameter could be any meaningful mono-dimensional measure of the process, such as time between production errors, or length along a roll of fabric in the weaving manufacturing process. It is a particular case of the gamma distribution. It is the continuous analogue of the geometric distribution, and it has the key property of being memoryless. In addition to being used for the analysis of Poisson point processes it is found in various other contexts.
In combinatory logic for computer science, a fixed-point combinator, is a higher-order function that returns some fixed point of its argument function, if one exists.
In mathematics, a self-adjoint operator on a complex vector space V with inner product is a linear map A that is its own adjoint. If V is finite-dimensional with a given orthonormal basis, this is equivalent to the condition that the matrix of A is a Hermitian matrix, i.e., equal to its conjugate transpose A∗. By the finite-dimensional spectral theorem, V has an orthonormal basis such that the matrix of A relative to this basis is a diagonal matrix with entries in the real numbers. This article deals with applying generalizations of this concept to operators on Hilbert spaces of arbitrary dimension.
In mathematical logic and computer science, the calculus of constructions (CoC) is a type theory created by Thierry Coquand. It can serve as both a typed programming language and as constructive foundation for mathematics. For this second reason, the CoC and its variants have been the basis for Coq and other proof assistants.
System F is a typed lambda calculus that introduces, to simply typed lambda calculus, a mechanism of universal quantification over types. System F formalizes parametric polymorphism in programming languages, thus forming a theoretical basis for languages such as Haskell and ML. It was discovered independently by logician Jean-Yves Girard (1972) and computer scientist John C. Reynolds.
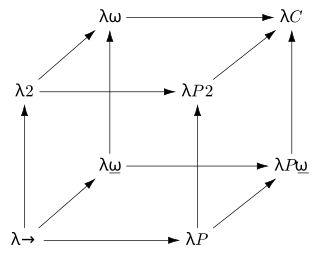
In mathematical logic and type theory, the λ-cube is a framework introduced by Henk Barendregt to investigate the different dimensions in which the calculus of constructions is a generalization of the simply typed λ-calculus. Each dimension of the cube corresponds to a new kind of dependency between terms and types. Here, "dependency" refers to the capacity of a term or type to bind a term or type. The respective dimensions of the λ-cube correspond to:
In probability theory, a compound Poisson distribution is the probability distribution of the sum of a number of independent identically-distributed random variables, where the number of terms to be added is itself a Poisson-distributed variable. The result can be either a continuous or a discrete distribution.
Lambda lifting is a meta-process that restructures a computer program so that functions are defined independently of each other in a global scope. An individual "lift" transforms a local function into a global function. It is a two step process, consisting of;
In mathematics, Church encoding is a means of representing data and operators in the lambda calculus. The Church numerals are a representation of the natural numbers using lambda notation. The method is named for Alonzo Church, who first encoded data in the lambda calculus this way.
In statistics, the bias of an estimator is the difference between this estimator's expected value and the true value of the parameter being estimated. An estimator or decision rule with zero bias is called unbiased. In statistics, "bias" is an objective property of an estimator. Bias is a distinct concept from consistency: consistent estimators converge in probability to the true value of the parameter, but may be biased or unbiased; see bias versus consistency for more.

In probability theory and statistics, the Conway–Maxwell–Poisson distribution is a discrete probability distribution named after Richard W. Conway, William L. Maxwell, and Siméon Denis Poisson that generalizes the Poisson distribution by adding a parameter to model overdispersion and underdispersion. It is a member of the exponential family, has the Poisson distribution and geometric distribution as special cases and the Bernoulli distribution as a limiting case.
In statistics, principal component regression (PCR) is a regression analysis technique that is based on principal component analysis (PCA). More specifically, PCR is used for estimating the unknown regression coefficients in a standard linear regression model.
In probability theory, concentration inequalities provide mathematical bounds on the probability of a random variable deviating from some value.
A Hindley–Milner (HM) type system is a classical type system for the lambda calculus with parametric polymorphism. It is also known as Damas–Milner or Damas–Hindley–Milner. It was first described by J. Roger Hindley and later rediscovered by Robin Milner. Luis Damas contributed a close formal analysis and proof of the method in his PhD thesis.
Computable topology is a discipline in mathematics that studies the topological and algebraic structure of computation. Computable topology is not to be confused with algorithmic or computational topology, which studies the application of computation to topology.
In computer science, a "let" expression associates a function definition with a restricted scope.
Lambda calculus is a formal mathematical system based on lambda abstraction and function application. Two definitions of the language are given here: a standard definition, and a definition using mathematical formulas.
A Boltzmann sampler is an algorithm intended for random sampling of combinatorial structures. If the object size is viewed as its energy, and the argument of the corresponding generating function is interpreted in terms of the temperature of the physical system, then a Boltzmann sampler returns an object from a classical Boltzmann distribution.




















