Preference ranking organization method for enrichment evaluation

Last updated March 20, 2024

The Preference Ranking Organization METHod for Enrichment of Evaluations and its descriptive complement geometrical analysis for interactive aid are better known as the Promethee and Gaia^[1] methods.

Rather than pointing out a "right" decision, the Promethee and Gaia method helps decision makers find the alternative that best suits their goal and their understanding of the problem. It provides a comprehensive and rational framework for structuring a decision problem, identifying and quantifying its conflicts and synergies, clusters of actions, and highlight the main alternatives and the structured reasoning behind.

History

The basic elements of the Promethee method have been first introduced by Professor Jean-Pierre Brans (CSOO, VUB Vrije Universiteit Brussel) in 1982.^[2] It was later developed and implemented by Professor Jean-Pierre Brans and Professor Bertrand Mareschal (Solvay Brussels School of Economics and Management, ULB Université Libre de Bruxelles), including extensions such as GAIA.

The descriptive approach, named Gaia,^[3] allows the decision maker to visualize the main features of a decision problem: he/she is able to easily identify conflicts or synergies between criteria, to identify clusters of actions and to highlight remarkable performances.

The prescriptive approach, named Promethee,^[4] provides the decision maker with both complete and partial rankings of the actions.

Promethee has successfully been used in many decision making contexts worldwide. A non-exhaustive list of scientific publications about extensions, applications and discussions related to the Promethee methods^[5] was published in 2010.

Uses and applications

While it can be used by individuals working on straightforward decisions, the Promethee & Gaia is most useful where groups of people are working on complex problems, especially those with several criteria, involving a lot of human perceptions and judgments, whose decisions have long-term impact. It has unique advantages when important elements of the decision are difficult to quantify or compare, or where collaboration among departments or team members are constrained by their different specializations or perspectives.

Decision situations to which the Promethee and Gaia can be applied include:

Choice – The selection of one alternative from a given set of alternatives, usually where there are multiple decision criteria involved.
Prioritization – Determining the relative merit of members of a set of alternatives, as opposed to selecting a single one or merely ranking them.
Resource allocation – Allocating resources among a set of alternatives
Ranking – Putting a set of alternatives in order from most to least preferred
Conflict resolution – Settling disputes between parties with apparently incompatible objectives

The applications of Promethee and Gaia to complex multi-criteria decision scenarios have numbered in the thousands, and have produced extensive results in problems involving planning, resource allocation, priority setting, and selection among alternatives. Other areas have included forecasting, talent selection, and tender analysis.

Some uses of Promethee and Gaia have become case-studies. Recently these have included:

Deciding which resources are the best with the available budget to meet SPS quality standards (STDF – WTO) [See more in External Links]
Selecting new route for train performance (Italferr)[See more in External Links]

The mathematical model

Assumptions

Let $A=\{a_{1},..,a_{n}\}$ be a set of n actions and let $F=\{f_{1},..,f_{q}\}$ be a consistent family of q criteria. Without loss of generality, we will assume that these criteria have to be maximized.

The basic data related to such a problem can be written in a table containing $n\times q$ evaluations. Each line corresponds to an action and each column corresponds to a criterion.

{\begin{array}{|c|c|c|c|c|c|c|}\hline &f_{1}(\cdot )&f_{2}(\cdot )&\cdots &f_{j}(\cdot )&\cdots &f_{q}(\cdot )\\\hline a_{1}&f_{1}(a_{1})&f_{2}(a_{1})&\cdots &f_{j}(a_{1})&\cdots &f_{q}(a_{1})\\\hline a_{2}&f_{1}(a_{2})&f_{2}(a_{2})&\cdots &f_{j}(a_{2})&\cdots &f_{q}(a_{2})\\\hline \cdots &\cdots &\cdots &\cdots &\cdots &\cdots &.\cdots \\\hline a_{i}&f_{1}(a_{i})&f_{2}(a_{i})&\cdots &f_{j}(a_{i})&\cdots &f_{q}(a_{i})\\\hline \cdots &\cdots &\cdots &\cdots &\cdots &\cdots &\cdots \\\hline a_{n}&f_{1}(a_{n})&f_{2}(a_{n})&\cdots &f_{j}(a_{n})&\cdots &f_{q}(a_{n})\\\hline \end{array}}

Pairwise comparisons

At first, pairwise comparisons will be made between all the actions for each criterion:

d_{k}(a_{i},a_{j})=f_{k}(a_{i})-f_{k}(a_{j})

$d_{k}(a_{i},a_{j})$ is the difference between the evaluations of two actions for criterion $f_{k}$ . Of course, these differences depend on the measurement scales used and are not always easy to compare for the decision maker.

Preference degree

As a consequence the notion of preference function is introduced to translate the difference into a unicriterion preference degree as follows:

\pi _{k}(a_{i},a_{j})=P_{k}[d_{k}(a_{i},a_{j})]

where $P_{k}:\mathbb {R} \rightarrow [0,1]$ is a positive non-decreasing preference function such that $P_{k}(0)=0$ . Six different types of preference function are proposed in the original Promethee definition. Among them, the linear unicriterion preference function is often used in practice for quantitative criteria:

P_{k}(x){\begin{cases}0,&{\text{if }}x\leq q_{k}\\{\frac {x-q_{k}}{p_{k}-q_{k}}},&{\text{if }}q_{k}<x\leq p_{k}\\1,&{\text{if }}x>p_{k}\end{cases}}

where $q_{j}$ and $p_{j}$ are respectively the indifference and preference thresholds. The meaning of these parameters is the following: when the difference is smaller than the indifference threshold it is considered as negligible by the decision maker. Therefore, the corresponding unicriterion preference degree is equal to zero. If the difference exceeds the preference threshold it is considered to be significant. Therefore, the unicriterion preference degree is equal to one (the maximum value). When the difference is between the two thresholds, an intermediate value is computed for the preference degree using a linear interpolation.

Multicriteria preference degree

When a preference function has been associated to each criterion by the decision maker, all comparisons between all pairs of actions can be done for all the criteria. A multicriteria preference degree is then computed to globally compare every couple of actions:

\pi (a,b)=\displaystyle \sum _{k=1}^{q}P_{k}(a,b)\cdot w_{k}

Where $w_{k}$ represents the weight of criterion $f_{k}$ . It is assumed that $w_{k}\geq 0$ and $\sum _{k=1}^{q}w_{k}=1$ . As a direct consequence, we have:

\pi (a_{i},a_{j})\geq 0

\pi (a_{i},a_{j})+\pi (a_{j},a_{i})\leq 1

Multicriteria preference flows

In order to position every action with respect to all the other actions, two scores are computed:

\phi ^{+}(a)={\frac {1}{n-1}}\displaystyle \sum _{x\in A}\pi (a,x)

\phi ^{-}(a)={\frac {1}{n-1}}\displaystyle \sum _{x\in A}\pi (x,a)

The positive preference flow $\phi ^{+}(a_{i})$ quantifies how a given action $a_{i}$ is globally preferred to all the other actions while the negative preference flow $\phi ^{-}(a_{i})$ quantifies how a given action $a_{i}$ is being globally preferred by all the other actions. An ideal action would have a positive preference flow equal to 1 and a negative preference flow equal to 0. The two preference flows induce two generally different complete rankings on the set of actions. The first one is obtained by ranking the actions according to the decreasing values of their positive flow scores. The second one is obtained by ranking the actions according to the increasing values of their negative flow scores. The Promethee I partial ranking is defined as the intersection of these two rankings. As a consequence, an action $a_{i}$ will be as good as another action $a_{j}$ if $\phi ^{+}(a_{i})\geq \phi ^{+}(a_{j})$ and $\phi ^{-}(a_{i})\leq \phi ^{-}(a_{j})$

The positive and negative preference flows are aggregated into the net preference flow:

\phi (a)=\phi ^{+}(a)-\phi ^{-}(a)

Direct consequences of the previous formula are:

\phi (a_{i})\in [-1;1]

\sum _{a_{i}\in A}\phi (a_{i})=0

The Promethee II complete ranking is obtained by ordering the actions according to the decreasing values of the net flow scores.

Unicriterion net flows

According to the definition of the multicriteria preference degree, the multicriteria net flow can be disaggregated as follows:

\phi (a_{i})=\displaystyle \sum _{k=1}^{q}\phi _{k}(a_{i}).w_{k}

Where:

\phi _{k}(a_{i})={\frac {1}{n-1}}\displaystyle \sum _{a_{j}\in A}\{P_{k}(a_{i},a_{j})-P_{k}(a_{j},a_{i})\}

.

The unicriterion net flow, denoted $\phi _{k}(a_{i})\in [-1;1]$ , has the same interpretation as the multicriteria net flow $\phi (a_{i})$ but is limited to one single criterion. Any action $a_{i}$ can be characterized by a vector ${\vec {\phi }}(a_{i})=[\phi _{1}(a_{i}),\ldots ,\phi _{k}(a_{i}),\phi _{q}(a_{i})]$ in a $q$ dimensional space. The GAIA plane is the principal plane obtained by applying a principal components analysis to the set of actions in this space.

Promethee preference functions

Usual

P_{j}(d_{j})={\begin{cases}0&{\text{if }}d_{j}\leq 0\\[4pt]1&{\text{if }}d_{j}>0\end{cases}}

U-shape

{\begin{array}{cc}P_{j}(d_{j})=\left\{{\begin{array}{lll}0&{\text{if}}&|d_{j}|\leq q_{j}\\\\1&{\text{if}}&|d_{j}|>q_{j}\\\end{array}}\right.\end{array}}

V-shape

{\begin{array}{cc}P_{j}(d_{j})=\left\{{\begin{array}{lll}{\frac {|d_{j}|}{p_{j}}}&{\text{if}}&|d_{j}|\leq p_{j}\\\\1&{\text{if}}&|d_{j}|>p_{j}\\\end{array}}\right.\end{array}}

Level

{\begin{array}{cc}P_{j}(d_{j})=\left\{{\begin{array}{lll}0&{\text{if}}&|d_{j}|\leq q_{j}\\\\{\frac {1}{2}}&{\text{if}}&q_{j}<|d_{j}|\leq p_{j}\\\\1&{\text{if}}&|d_{j}|>p_{j}\\\end{array}}\right.\end{array}}

Linear

{\begin{array}{cc}P_{j}(d_{j})=\left\{{\begin{array}{lll}0&{\text{if}}&|d_{j}|\leq q_{j}\\\\{\frac {|d_{j}|-q_{j}}{p_{j}-q_{j}}}&{\text{if}}&q_{j}<|d_{j}|\leq p_{j}\\\\1&{\text{if}}&|d_{j}|>p_{j}\\\end{array}}\right.\end{array}}

Gaussian

P_{j}(d_{j})=1-e^{-{\frac {d_{j}^{2}}{2s_{j}^{2}}}}

Promethee rankings

Promethee I

Promethee I is a partial ranking of the actions. It is based on the positive and negative flows. It includes preferences, indifferences and incomparabilities (partial preorder).

Promethee II

Promethee II is a complete ranking of the actions. It is based on the multicriteria net flow. It includes preferences and indifferences (preorder).

Related Research Articles

In number theory, an arithmetic, arithmetical, or number-theoretic function is generally any function f(n) whose domain is the positive integers and whose range is a subset of the complex numbers. Hardy & Wright include in their definition the requirement that an arithmetical function "expresses some arithmetical property of n". There is a larger class of number-theoretic functions that do not fit this definition, for example, the prime-counting functions. This article provides links to functions of both classes.

Distributions, also known as Schwartz distributions or generalized functions, are objects that generalize the classical notion of functions in mathematical analysis. Distributions make it possible to differentiate functions whose derivatives do not exist in the classical sense. In particular, any locally integrable function has a distributional derivative.

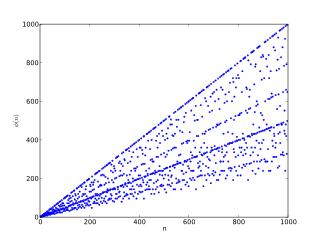
<span class="mw-page-title-main">Euler's totient function</span> Number of integers coprime to and not exceeding n

In number theory, Euler's totient function counts the positive integers up to a given integer $n$ that are relatively prime to $n$ . It is written using the Greek letter phi as $or, and may also be called Euler's phi function . In other words, it is the number of integers k in the range 1 \leq k \leq n for which the greatest common divisor gcd(n, k) is equal to 1. The integers k of this form are sometimes referred to as totatives of n .$

In vector calculus, the divergence theorem, also known as Gauss's theorem or Ostrogradsky's theorem, is a theorem relating the flux of a vector field through a closed surface to the divergence of the field in the volume enclosed.

In analytic number theory and related branches of mathematics, a complex-valued arithmetic function $:\mathbb {Z} \rightarrow \mathbb {C} }$ is a Dirichlet character of modulus if for all integers $and :$

$that is, is completely multiplicative.$
$; that is, is periodic with period .$

In mathematical analysis, the Minkowski inequality establishes that the L^p spaces are normed vector spaces. Let $be a measure space, let and let and be elements of Then is in and we have the triangle inequality$

Stellar dynamics is the branch of astrophysics which describes in a statistical way the collective motions of stars subject to their mutual gravity. The essential difference from celestial mechanics is that the number of body

In mathematics, specifically the algebraic theory of fields, a normal basis is a special kind of basis for Galois extensions of finite degree, characterised as forming a single orbit for the Galois group. The normal basis theorem states that any finite Galois extension of fields has a normal basis. In algebraic number theory, the study of the more refined question of the existence of a normal integral basis is part of Galois module theory.

In abstract algebra, Hilbert's Theorem 90 (or Satz 90) is an important result on cyclic extensions of fields (or to one of its generalizations) that leads to Kummer theory. In its most basic form, it states that if L/K is an extension of fields with cyclic Galois group G = Gal(L/K) generated by an element $and if is an element of L of relative norm 1, that is$

In quantum field theory, the Wightman distributions can be analytically continued to analytic functions in Euclidean space with the domain restricted to the ordered set of points in Euclidean space with no coinciding points. These functions are called the Schwinger functions and they are real-analytic, symmetric under the permutation of arguments, Euclidean covariant and satisfy a property known as reflection positivity. Properties of Schwinger functions are known as Osterwalder–Schrader axioms. Schwinger functions are also referred to as Euclidean correlation functions.

In mathematics, the Iverson bracket, named after Kenneth E. Iverson, is a notation that generalises the Kronecker delta, which is the Iverson bracket of the statement $x = y$ . It maps any statement to a function of the free variables in that statement. This function is defined to take the value 1 for the values of the variables for which the statement is true, and takes the value 0 otherwise. It is generally denoted by putting the statement inside square brackets:

In mathematics and mathematical physics, raising and lowering indices are operations on tensors which change their type. Raising and lowering indices are a form of index manipulation in tensor expressions.

In mechanical engineering, the Denavit–Hartenberg parameters are the four parameters associated with a particular convention for attaching reference frames to the links of a spatial kinematic chain, or robot manipulator.

In mathematics, the spectral theory of ordinary differential equations is the part of spectral theory concerned with the determination of the spectrum and eigenfunction expansion associated with a linear ordinary differential equation. In his dissertation, Hermann Weyl generalized the classical Sturm–Liouville theory on a finite closed interval to second order differential operators with singularities at the endpoints of the interval, possibly semi-infinite or infinite. Unlike the classical case, the spectrum may no longer consist of just a countable set of eigenvalues, but may also contain a continuous part. In this case the eigenfunction expansion involves an integral over the continuous part with respect to a spectral measure, given by the Titchmarsh–Kodaira formula. The theory was put in its final simplified form for singular differential equations of even degree by Kodaira and others, using von Neumann's spectral theorem. It has had important applications in quantum mechanics, operator theory and harmonic analysis on semisimple Lie groups.

Uncertainty theory is a branch of mathematics based on normality, monotonicity, self-duality, countable subadditivity, and product measure axioms.

In mathematics, the ring of polynomial functions on a vector space V over a field k gives a coordinate-free analog of a polynomial ring. It is denoted by k[V]. If V is finite dimensional and is viewed as an algebraic variety, then k[V] is precisely the coordinate ring of V.

In the mathematical field of group theory, an Artin transfer is a certain homomorphism from an arbitrary finite or infinite group to the commutator quotient group of a subgroup of finite index. Originally, such mappings arose as group theoretic counterparts of class extension homomorphisms of abelian extensions of algebraic number fields by applying Artin's reciprocity maps to ideal class groups and analyzing the resulting homomorphisms between quotients of Galois groups. However, independently of number theoretic applications, a partial order on the kernels and targets of Artin transfers has recently turned out to be compatible with parent-descendant relations between finite p-groups, which can be visualized in descendant trees. Therefore, Artin transfers provide a valuable tool for the classification of finite p-groups and for searching and identifying particular groups in descendant trees by looking for patterns defined by the kernels and targets of Artin transfers. These strategies of pattern recognition are useful in purely group theoretic context, as well as for applications in algebraic number theory concerning Galois groups of higher p-class fields and Hilbert p-class field towers.

In mathematical physics, Clebsch–Gordan coefficients are the expansion coefficients of total angular momentum eigenstates in an uncoupled tensor product basis. Mathematically, they specify the decomposition of the tensor product of two irreducible representations into a direct sum of irreducible representations, where the type and the multiplicities of these irreducible representations are known abstractly. The name derives from the German mathematicians Alfred Clebsch (1833–1872) and Paul Gordan (1837–1912), who encountered an equivalent problem in invariant theory.

In variational Bayesian methods, the evidence lower bound is a useful lower bound on the log-likelihood of some observed data.

This article summarizes several identities in exterior calculus.

References

↑ J. Figueira; S. Greco & M. Ehrgott (2005). Multiple Criteria Decision Analysis: State of the Art Surveys. Springer Verlag.
↑ J.P. Brans (1982). "L'ingénierie de la décision: élaboration d'instruments d'aide à la décision. La méthode PROMETHEE". Presses de l’Université Laval.
↑ B. Mareschal; J.P. Brans (1988). "Geometrical representations for MCDA. the GAIA module". European Journal of Operational Research.
↑ J.P. Brans & P. Vincke (1985). "A preference ranking organisation method: The PROMETHEE method for MCDM". Management Science.
↑ M. Behzadian; R.B. Kazemzadeh; A. Albadvi; M. Aghdasi (2010). "PROMETHEE: A comprehensive literature review on methodologies and applications". European Journal of Operational Research.

External links

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[Figueria-1] J. Figueira; S. Greco & M. Ehrgott (2005). Multiple Criteria Decision Analysis: State of the Art Surveys. Springer Verlag.

[Brans-2] J.P. Brans (1982). "L'ingénierie de la décision: élaboration d'instruments d'aide à la décision. La méthode PROMETHEE". Presses de l’Université Laval.

[Gaia-3] B. Mareschal; J.P. Brans (1988). "Geometrical representations for MCDA. the GAIA module". European Journal of Operational Research.

[Promethee-4] J.P. Brans & P. Vincke (1985). "A preference ranking organisation method: The PROMETHEE method for MCDM". Management Science.

[applications-5] M. Behzadian; R.B. Kazemzadeh; A. Albadvi; M. Aghdasi (2010). "PROMETHEE: A comprehensive literature review on methodologies and applications". European Journal of Operational Research.

[1]

[2]

[3]

[4]

[5]