
In geometry, a set of points is convex if it contains every line segment between two points in the set. Equivalently, a convex set or a convex region is a set that intersects every line in a line segment, single point, or the empty set. For example, a solid cube is a convex set, but anything that is hollow or has an indent, for example, a crescent shape, is not convex.
In commutative algebra, the prime spectrum of a commutative ring is the set of all prime ideals of , and is usually denoted by ; in algebraic geometry it is simultaneously a topological space equipped with the sheaf of rings .

In mathematics, topological groups are the combination of groups and topological spaces, i.e. they are groups and topological spaces at the same time, such that the continuity condition for the group operations connects these two structures together and consequently they are not independent from each other.
In mathematics, a topological vector space is one of the basic structures investigated in functional analysis. A topological vector space is a vector space that is also a topological space with the property that the vector space operations are also continuous functions. Such a topology is called a vector topology and every topological vector space has a uniform topological structure, allowing a notion of uniform convergence and completeness. Some authors also require that the space is a Hausdorff space. One of the most widely studied categories of TVSs are locally convex topological vector spaces. This article focuses on TVSs that are not necessarily locally convex. Other well-known examples of TVSs include Banach spaces, Hilbert spaces and Sobolev spaces.
In mathematics, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra. Complementarily, noncommutative algebra is the study of ring properties that are not specific to commutative rings. This distinction results from the high number of fundamental properties of commutative rings that do not extend to noncommutative rings.
In mathematics, Hilbert's Nullstellensatz is a theorem that establishes a fundamental relationship between geometry and algebra. This relationship is the basis of algebraic geometry. It relates algebraic sets to ideals in polynomial rings over algebraically closed fields. This relationship was discovered by David Hilbert, who proved the Nullstellensatz in his second major paper on invariant theory in 1893.
In combinatorics, a branch of mathematics, a matroid is a structure that abstracts and generalizes the notion of linear independence in vector spaces. There are many equivalent ways to define a matroid axiomatically, the most significant being in terms of: independent sets; bases or circuits; rank functions; closure operators; and closed sets or flats. In the language of partially ordered sets, a finite simple matroid is equivalent to a geometric lattice.
In mathematics, a transcendental extension is a field extension such that there exists an element in the field that is transcendental over the field ; that is, an element that is not a root of any univariate polynomial with coefficients in . In other words, a transcendental extension is a field extension that is not algebraic. For example, and are both transcendental extensions of

In mathematics, an affine space is a geometric structure that generalizes some of the properties of Euclidean spaces in such a way that these are independent of the concepts of distance and measure of angles, keeping only the properties related to parallelism and ratio of lengths for parallel line segments. Affine space is the setting for affine geometry.
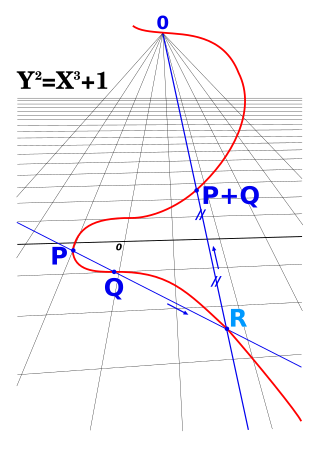
In algebraic geometry, a projective variety is an algebraic variety that is a closed subvariety of a projective space. That is, it is the zero-locus in of some finite family of homogeneous polynomials that generate a prime ideal, the defining ideal of the variety.
In mathematics, a closure operator on a set S is a function :{\mathcal {P}}(S)\rightarrow {\mathcal {P}}(S)} from the power set of S to itself that satisfies the following conditions for all sets
In mathematics, a duality translates concepts, theorems or mathematical structures into other concepts, theorems or structures in a one-to-one fashion, often by means of an involution operation: if the dual of A is B, then the dual of B is A. In other cases the dual of the dual – the double dual or bidual – is not necessarily identical to the original. Such involutions sometimes have fixed points, so that the dual of A is A itself. For example, Desargues' theorem is self-dual in this sense under the standard duality in projective geometry.
In the mathematical fields of linear algebra and functional analysis, the orthogonal complement of a subspace of a vector space equipped with a bilinear form is the set of all vectors in that are orthogonal to every vector in . Informally, it is called the perp, short for perpendicular complement. It is a subspace of .
In functional and convex analysis, and related disciplines of mathematics, the polar set is a special convex set associated to any subset of a vector space lying in the dual space The bipolar of a subset is the polar of but lies in .
In algebraic geometry, Proj is a construction analogous to the spectrum-of-a-ring construction of affine schemes, which produces objects with the typical properties of projective spaces and projective varieties. The construction, while not functorial, is a fundamental tool in scheme theory.
A Dynkin system, named after Eugene Dynkin, is a collection of subsets of another universal set satisfying a set of axioms weaker than those of 𝜎-algebra. Dynkin systems are sometimes referred to as 𝜆-systems or d-system. These set families have applications in measure theory and probability.
In mathematics, the Noether normalization lemma is a result of commutative algebra, introduced by Emmy Noether in 1926. It states that for any field k, and any finitely generated commutative k-algebraA, there exist elements y1, y2, ..., yd in A that are algebraically independent over k and such that A is a finitely generated module over the polynomial ring S = k [y1, y2, ..., yd]. The integer d is equal to the Krull dimension of the ring A; and if A is an integral domain, d is also the transcendence degree of the field of fractions of A over k.
In algebraic geometry, a morphism between algebraic varieties is a function between the varieties that is given locally by polynomials. It is also called a regular map. A morphism from an algebraic variety to the affine line is also called a regular function. A regular map whose inverse is also regular is called biregular, and the biregular maps are the isomorphisms of algebraic varieties. Because regular and biregular are very restrictive conditions – there are no non-constant regular functions on projective varieties – the concepts of rational and birational maps are widely used as well; they are partial functions that are defined locally by rational fractions instead of polynomials.
This is a glossary of algebraic geometry.
In mathematics, a derivation of a commutative ring is called a locally nilpotent derivation (LND) if every element of is annihilated by some power of .









































































