S.I.N. Theory

Last updated
S.I.N. Theory
Directed byRichie Mitchell
Written byRichie Mitchell
Produced byRichie Mitchell
Jeremy Larter
Casper Lundbak
StarringJeremy Larter
Allison Dawn Doiron
Farid Yazdani
Richard Guppy
Kevin Stonefield
Stephen Jacob Hogan
Ed Lewis
Edited byLachey Wall, Luke Higginson
Music byPeter Rankin
Transits Of Mercury
Distributed byMCTV/Continuum Motion Pictures
Release date
  • November 4, 2012 (2012-11-04)(Moving Image Film Festival)
Running time
70 minutes
CountryCanada
LanguageEnglish

S.I.N. Theory (abbreviation for social insurance number theory) [1] is a 2012 Canadian science fiction drama film about a mathematics professor creating an algorithm capable of predicting an individual's future. The film was written and directed by Richie Mitchell, and stars Jeremy Larter and Allison Dawn Doiron. S.I.N. Theory was produced on a shoestring budget and makes notable use of existing mathematical theories to affirm the concept's plausibility. [2] The film has been screened at science fiction film festivals in Canada and the US, whereas it has been picked up for distribution by MCTV with an anticipated release Fall 2013. [3]

Contents

Plot

Michael, a mathematics professor having dedicated his career to creating the ultimate game theory, is finally let go for being a long time black mark of the faculty. Despite becoming a laughing stock, Michael continues his work from home and is now free from legal parameters the faculty has enforced. Determined to prove all naysayers wrong, and to reclaim if not heighten his name, Michael gives the go-ahead to his anonymous colleague, a hacker, to obtain access to the populace’s full credit and health report information. This illegal database proves to be the last piece of the puzzle, and with it, Michael is able to accurately calculate and therefore predict the outcome to nearly any situation. As it was initially thought to be of use for stock markets and political predicaments, Michael however curiously formulates the life span of his favorite student, a young woman he loves, and finds out she only has days to live. The further he unravels the possibilities and dangers the algorithm beholds, his morality, its proper use, and reporting of it comes into question. Each time he uses the algorithm, he taps into the credit report database and his illegal presence becomes vulnerable, and ultimately discovered by a competing-dirty corporation, hell bent on the same goal for monetary purposes. Michael must choose what to do with this illegal and very powerful equation; either to publish it for his own glorious demise, or save the woman he loves and risk annihilation by the threatening competitor.

Cast

Production

In writing the script, a contained sci-fi written explicitly for the accommodation of the budget, Mitchell wished to incorporate existing mathematical theories to showcase the plausibility of such an equation, and how to a certain extent, already exists today. In a viral video interview posted on the film's website, [1] Mitchell talks of drawing comparisons to observing human behavior to the study of fluid behavior, as depicted by the Navier–Stokes equations, an attribution to Mitchell's engineering education.

Having undergone development hell on a couple other of his projects, Mitchell decided to finance the film along with support of close friends and family. [2] It was shot in Little Italy, Toronto, the residing neighborhood of Mitchell, Larter and Doiron. Often the trio would simply lug gear to site, film their scenes quickly and without a permit, and move on, accumulating to a 2-week production schedule plus a summer of weekend pick-ups. [4] Since all locations were filmed at no cost, the location of the Dean's office proved to be the most difficult. It wasn't until that Larter and Mitchell coincidentally vacationed on Prince Edward Island at the same time were they able to swoop in at an elementary school and film the scene with Kevin Stonefield, a retired school principal and a long time friend of Mitchell's who's been in several of Mitchell's short films.

An earlier version of the film was screened at the 2013 Boston Science Fiction Film Festival. After a year since post production began, Mitchell hired a new editor Luke Higgenson to perform a final edit to strengthen pacing and also to add a fresh voice to the project. [4] Upon completion of the new and final edit, the film was picked up for distribution by MCTV (Continuum Motion Pictures) and is intended to be released on VOD, Dish Channel and various platforms Fall 2013. [5]

Release

FestivalCityYear
Boston Science Fiction Film FestivalBoston, USA,2013
Philip K. Dyck Science Fiction Film FestivalNew York City, USA,2012
Moving Image Film FestivalToronto, Canada,2012

Reception

Rogue cinema called S.I.N. Theory "one of the most compelling indie flicks of the year" [6] while others such as Filmmaking Review have applauded the director's work given the budgetary restraints. [7]

Related Research Articles

<span class="mw-page-title-main">Chaos theory</span> Field of mathematics and science based on non-linear systems and initial conditions

Chaos theory is an interdisciplinary area of scientific study and branch of mathematics. It focuses on underlying patterns and deterministic laws of dynamical systems that are highly sensitive to initial conditions. These were once thought to have completely random states of disorder and irregularities. Chaos theory states that within the apparent randomness of chaotic complex systems, there are underlying patterns, interconnection, constant feedback loops, repetition, self-similarity, fractals and self-organization. The butterfly effect, an underlying principle of chaos, describes how a small change in one state of a deterministic nonlinear system can result in large differences in a later state. A metaphor for this behavior is that a butterfly flapping its wings in Brazil can cause a tornado in Texas.

<span class="mw-page-title-main">Discrete mathematics</span> Study of discrete mathematical structures

Discrete mathematics is the study of mathematical structures that can be considered "discrete" rather than "continuous". Objects studied in discrete mathematics include integers, graphs, and statements in logic. By contrast, discrete mathematics excludes topics in "continuous mathematics" such as real numbers, calculus or Euclidean geometry. Discrete objects can often be enumerated by integers; more formally, discrete mathematics has been characterized as the branch of mathematics dealing with countable sets. However, there is no exact definition of the term "discrete mathematics".

A mathematical model is an abstract description of a concrete system using mathematical concepts and language. The process of developing a mathematical model is termed mathematical modeling. Mathematical models are used in applied mathematics and in the natural sciences and engineering disciplines, as well as in non-physical systems such as the social sciences. It can also be taught as a subject in its own right.

<span class="mw-page-title-main">Number theory</span> Mathematics of integer properties

Number theory is a branch of pure mathematics devoted primarily to the study of the integers and arithmetic functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Mathematics is the queen of the sciences—and number theory is the queen of mathematics." Number theorists study prime numbers as well as the properties of mathematical objects constructed from integers, or defined as generalizations of the integers.

The following outline is provided as an overview of and topical guide to statistics:

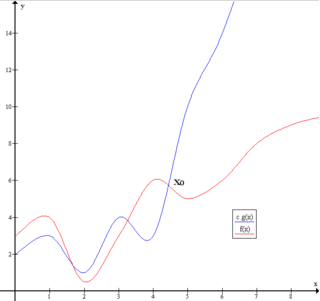
Big <i>O</i> notation Describes limiting behavior of a function

Big O notation is a mathematical notation that describes the limiting behavior of a function when the argument tends towards a particular value or infinity. Big O is a member of a family of notations invented by German mathematicians Paul Bachmann, Edmund Landau, and others, collectively called Bachmann–Landau notation or asymptotic notation. The letter O was chosen by Bachmann to stand for Ordnung, meaning the order of approximation.

<span class="mw-page-title-main">Gabriel Lamé</span> French mathematician (1795–1870)

Gabriel Lamé was a French mathematician who contributed to the theory of partial differential equations by the use of curvilinear coordinates, and the mathematical theory of elasticity.

<span class="mw-page-title-main">Andrey Kolmogorov</span> Soviet mathematician (1903–1987)

Andrey Nikolaevich Kolmogorov was a Soviet mathematician who contributed to the mathematics of probability theory, topology, intuitionistic logic, turbulence, classical mechanics, algorithmic information theory and computational complexity.

In mathematics and science, a nonlinear system is a system in which the change of the output is not proportional to the change of the input. Nonlinear problems are of interest to engineers, biologists, physicists, mathematicians, and many other scientists since most systems are inherently nonlinear in nature. Nonlinear dynamical systems, describing changes in variables over time, may appear chaotic, unpredictable, or counterintuitive, contrasting with much simpler linear systems.

"The Unreasonable Effectiveness of Mathematics in the Natural Sciences" is a 1960 article written by the physicist Eugene Wigner, published in Communication in Pure and Applied Mathematics. In it, Wigner observes that a theoretical physics's mathematical structure often points the way to further advances in that theory and to empirical predictions. Mathematical theories often have predictive power in describing nature.

Theoretical computer science is a subfield of computer science and mathematics that focuses on the abstract and mathematical foundations of computation, such as the theory of computation, formal language theory, the lambda calculus and type theory.

Lists of mathematics topics cover a variety of topics related to mathematics. Some of these lists link to hundreds of articles; some link only to a few. The template to the right includes links to alphabetical lists of all mathematical articles. This article brings together the same content organized in a manner better suited for browsing. Lists cover aspects of basic and advanced mathematics, methodology, mathematical statements, integrals, general concepts, mathematical objects, and reference tables. They also cover equations named after people, societies, mathematicians, journals, and meta-lists.

In mathematics, a Dirichlet problem asks for a function which solves a specified partial differential equation (PDE) in the interior of a given region that takes prescribed values on the boundary of the region.

<span class="mw-page-title-main">Qin Jiushao</span> Chinese mathematician and inventor

Qin Jiushao, courtesy name Daogu (道古), was a Chinese mathematician, meteorologist, inventor, politician, and writer. He is credited for discovering Horner's method as well as inventing Tianchi basins, a type of rain gauge instrument used to gather meteorological data.

<span class="mw-page-title-main">Terence Tao</span> Australian-American mathematician (born 1975)

Terence Chi-Shen Tao is an Australian mathematician who is a professor of mathematics at the University of California, Los Angeles (UCLA), where he holds the James and Carol Collins Chair in the College of Letters and Sciences. His research includes topics in harmonic analysis, partial differential equations, algebraic combinatorics, arithmetic combinatorics, geometric combinatorics, probability theory, compressed sensing and analytic number theory.

<span class="mw-page-title-main">Pierre-Louis Lions</span> French mathematician (born 1956)

Pierre-Louis Lions is a French mathematician. He is known for a number of contributions to the fields of partial differential equations and the calculus of variations. He was a recipient of the 1994 Fields Medal and the 1991 Prize of the Philip Morris tobacco and cigarette company.

This is a timeline of pure and applied mathematics history. It is divided here into three stages, corresponding to stages in the development of mathematical notation: a "rhetorical" stage in which calculations are described purely by words, a "syncopated" stage in which quantities and common algebraic operations are beginning to be represented by symbolic abbreviations, and finally a "symbolic" stage, in which comprehensive notational systems for formulas are the norm.

Eureqa was a proprietary modeling engine created in Cornell's Artificial Intelligence Lab and later commercialized by Nutonian, Inc. The software used genetic algorithms to determine mathematical equations that describe sets of data in their simplest form, a technique referred to as symbolic regression.

<span class="mw-page-title-main">Gennady Makanin</span> Russian mathematician (1938–2017)

GennadySemenovich Makanin (1938–2017) was a Russian mathematician, awarded the 2010 I. M. Vinogradov Prize for a series of papers on the problem of algorithmically recognizing the solvability of arbitrary equations in free groups and semigroups.

References

  1. 1 2 "S.I.N. Theory Press" . Retrieved 2013-08-16.
  2. 1 2 "S.I.N. Theory Bios" . Retrieved 2013-08-16.
  3. ↑ "S.I.N. Theory A Canadian Science Fiction Picked Up for Distribution" . Retrieved 2013-05-10.
  4. 1 2 "An Interview With Richie Mitchell" . Retrieved 2013-06-04.
  5. ↑ "S.I.N. Theory Release" . Retrieved 2013-08-16.
  6. ↑ "S.I.N. Theory (2013) - By Philip Smolen" . Retrieved 2013-05-03.
  7. ↑ "S.I.N. Theory Review" . Retrieved 2013-05-25.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.