
Half-life is the time required for a quantity to reduce to half of its initial value. The term is commonly used in nuclear physics to describe how quickly unstable atoms undergo radioactive decay or how long stable atoms survive. The term is also used more generally to characterize any type of exponential decay. For example, the medical sciences refer to the biological half-life of drugs and other chemicals in the human body. The converse of half-life is doubling time.
A nuclide is a class of atoms characterized by their number of protons, Z, their number of neutrons, N, and their nuclear energy state.
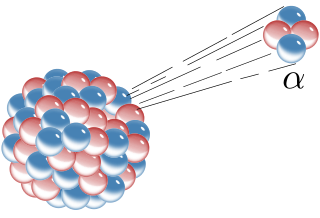
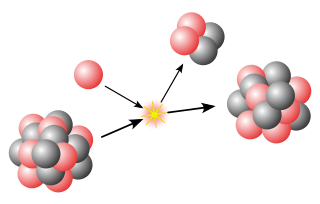
Radioactive decay is the process by which an unstable atomic nucleus loses energy by radiation. A material containing unstable nuclei is considered radioactive. Three of the most common types of decay are alpha, beta, and gamma decay. The weak force is the mechanism that is responsible for beta decay, while the other two are governed by the electromagnetism and nuclear force.

A quantity is subject to exponential decay if it decreases at a rate proportional to its current value. Symbolically, this process can be expressed by the following differential equation, where N is the quantity and λ (lambda) is a positive rate called the exponential decay constant, disintegration constant, rate constant, or transformation constant:
Population dynamics is the type of mathematics used to model and study the size and age composition of populations as dynamical systems.

In the context of radioactivity, activity or total activity (symbol A) is a physical quantity defined as the number of radioactive transformations per second that occur in a particular radionuclide. The unit of activity is the becquerel (symbol Bq), which is defined equivalent to reciprocal seconds (symbol s-1). The older, non-SI unit of activity is the curie (Ci), which is 3.7×1010 radioactive decay per second. Another unit of activity is the rutherford, which is defined as 1×106 radioactive decay per second.
In particle physics and nuclear physics, the branching fraction for a decay is the fraction of particles which decay by an individual decay mode or with respect to the total number of particles which decay. It applies to either the radioactive decay of atoms or the decay of elementary particles. It is equal to the ratio of the partial decay constant to the overall decay constant. Sometimes a partial half-life is given, but this term is misleading; due to competing modes, it is not true that half of the particles will decay through a particular decay mode after its partial half-life. The partial half-life is merely an alternate way to specify the partial decay constant λ, the two being related through:
The equilibrium constant of a chemical reaction is the value of its reaction quotient at chemical equilibrium, a state approached by a dynamic chemical system after sufficient time has elapsed at which its composition has no measurable tendency towards further change. For a given set of reaction conditions, the equilibrium constant is independent of the initial analytical concentrations of the reactant and product species in the mixture. Thus, given the initial composition of a system, known equilibrium constant values can be used to determine the composition of the system at equilibrium. However, reaction parameters like temperature, solvent, and ionic strength may all influence the value of the equilibrium constant.

Isochron dating is a common technique of radiometric dating and is applied to date certain events, such as crystallization, metamorphism, shock events, and differentiation of precursor melts, in the history of rocks. Isochron dating can be further separated into mineral isochron dating and whole rock isochron dating; both techniques are applied frequently to date terrestrial and also extraterrestrial rocks (meteorites). The advantage of isochron dating as compared to simple radiometric dating techniques is that no assumptions are needed about the initial amount of the daughter nuclide in the radioactive decay sequence. Indeed, the initial amount of the daughter product can be determined using isochron dating. This technique can be applied if the daughter element has at least one stable isotope other than the daughter isotope into which the parent nuclide decays.
In pharmacokinetics, the effective half-life is the rate of accumulation or elimination of a biochemical or pharmacological substance in an organism; it is the analogue of biological half-life when the kinetics are governed by multiple independent mechanisms. This is seen when there are multiple mechanisms of elimination, or when a drug occupies multiple pharmacological compartments. It reflects the cumulative effect of the individual half-lives, as observed by the changes in the actual serum concentration of a drug under a given dosing regimen. The complexity of biological systems means that most pharmacological substances do not have a single mechanism of elimination, and hence the observed or effective half-life does not reflect that of a single process, but rather the summation of multiple independent processes.
In physical systems, damping is the loss of energy of an oscillating system by dissipation. Damping is an influence within or upon an oscillatory system that has the effect of reducing or preventing its oscillation. Examples of damping include viscous damping in a fluid, surface friction, radiation, resistance in electronic oscillators, and absorption and scattering of light in optical oscillators. Damping not based on energy loss can be important in other oscillating systems such as those that occur in biological systems and bikes. Damping is not to be confused with friction, which is a type of dissipative force acting on a system. Friction can cause or be a factor of damping.
Decay correction is a method of estimating the amount of radioactive decay at some set time before it was actually measured.

In nuclear physics, the concept of a neutron cross section is used to express the likelihood of interaction between an incident neutron and a target nucleus. The neutron cross section σ can be defined as the area in cm2 for which the number of neutron-nuclei reactions taking place is equal to the product of the number of incident neutrons that would pass through the area and the number of target nuclei. In conjunction with the neutron flux, it enables the calculation of the reaction rate, for example to derive the thermal power of a nuclear power plant. The standard unit for measuring the cross section is the barn, which is equal to 10−28 m2 or 10−24 cm2. The larger the neutron cross section, the more likely a neutron will react with the nucleus.
The principle of detailed balance can be used in kinetic systems which are decomposed into elementary processes. It states that at equilibrium, each elementary process is in equilibrium with its reverse process.

The Tafel equation is an equation in electrochemical kinetics relating the rate of an electrochemical reaction to the overpotential. The Tafel equation was first deduced experimentally and was later shown to have a theoretical justification. The equation is named after Swiss chemist Julius Tafel.
" It describes how the electrical current through an electrode depends on the voltage difference between the electrode and the bulk electrolyte for a simple, unimolecular redox reaction ".
In nuclear physics, transient equilibrium is a situation in which equilibrium is reached by a parent-daughter radioactive isotope pair where the half-life of the daughter is shorter than the half-life of the parent. Contrary to secular equilibrium, the half-life of the daughter is not negligible compared to parent's half-life. An example of this is a molybdenum-99 generator producing technetium-99 for nuclear medicine diagnostic procedures. Such a generator is sometimes called a cow because the daughter product, in this case technetium-99, is milked at regular intervals. Transient equilibrium occurs after four half-lives, on average.
In physics and engineering, the time constant, usually denoted by the Greek letter τ (tau), is the parameter characterizing the response to a step input of a first-order, linear time-invariant (LTI) system. The time constant is the main characteristic unit of a first-order LTI system.
Equilibrium chemistry is concerned with systems in chemical equilibrium. The unifying principle is that the free energy of a system at equilibrium is the minimum possible, so that the slope of the free energy with respect to the reaction coordinate is zero. This principle, applied to mixtures at equilibrium provides a definition of an equilibrium constant. Applications include acid–base, host–guest, metal–complex, solubility, partition, chromatography and redox equilibria.

In nuclear physics, the Bateman equation is a mathematical model describing abundances and activities in a decay chain as a function of time, based on the decay rates and initial abundances. The model was formulated by Ernest Rutherford in 1905 and the analytical solution was provided by Harry Bateman in 1910.
Potassium–calcium dating, abbreviated K–Ca dating, is a radiometric dating method used in geochronology. It is based upon measuring the ratio of a parent isotope of potassium to a daughter isotope of calcium. This form of radioactive decay is accomplished through beta decay.














