Shape resonance

Last updated

In quantum mechanics, a shape resonance is a metastable state in which an electron is trapped due to the shape of a potential barrier. [1] Altunata [2] describes a state as being a shape resonance if, "the internal state of the system remains unchanged upon disintegration of the quasi-bound level." A more general discussion of resonances and their taxonomies in molecular system can be found in the review article by Schulz; [3] [4] for the discovery of the Fano resonance line-shape [5] and for the Majorana pioneering work in this field [6] by Antonio Bianconi; and for a mathematical review by Combes et al. [7]

Contents

Quantum mechanics

In quantum mechanics, a shape resonance, in contrast to a Feshbach resonance, is a resonance which is not turned into a bound state if the coupling between some degrees of freedom and the degrees of freedom associated to the fragmentation (reaction coordinates) are set to zero. More simply, the shape resonance total energy is more than the separated fragment energy. [8] Practical implications of this difference for lifetimes and spectral widths are mentioned in works such as Zobel. [9]

Related terms include a special kind of shape resonance, the core-excited shape resonance, and trap-induced shape resonance. [10]

Of course in one-dimensional systems, resonances are shape resonances. In a system with more than one degree of freedom, this definition makes sense only if the separable model, which supposes the two groups of degrees of freedom uncoupled, is a meaningful approximation. When the coupling becomes large, the situation is much less clear.

In the case of atomic and molecular electronic structure problems, it is well known that the self-consistent field (SCF) approximation is relevant at least as a starting point of more elaborate methods. The Slater determinants built from SCF orbitals (atomic or molecular orbitals) are shape resonances if only one electronic transition is required to emit one electron.

Today, there is some debate about the definition and even existence of the shape resonance in some systems observed with molecular spectroscopy. [11] It has been experimentally observed in the anionic yields from photofragmentation of small molecules to provide details of internal structure. [12]

In nuclear physics the concept of "Shape Resonance" is described by Amos de-Shalit and Herman Feshbach in their book. [13]

"It is well known that the scattering from a potential shows characteristics peaks, as a function of energy, for such values of E that make the integral number of wave lengths sit within the potential. The resulting shape resonances are rather broad, their width being of the order of ...."

The shape resonances were observed around the years 1949–54 in nuclear scattering experiments. They indicate broad asymmetric peaks in the scattering cross section of neutrons or protons scattered by nuclei. The name "shape resonance" has been introduced to describe the fact that the resonance in the potential scattering for the particle of energy E is controlled by the shape of the nucleus. In fact the shape resonance occurs where the integral number of wavelengths of the particle sit within the potential of the nucleus of radius R. Therefore, the measure of the energies of the shape resonances in the neutron-nucleus scattering have been used in the years from 1947 to 1954 to measure the radii R of the nuclei with the precision of ±1×10−13 cm as it can be seen in the chapter "Elastic Cross Sections" of A Textbook in Nuclear Physics by R. D. Evans. [14]

The "shape resonances" are discussed in general introductory academic courses of quantum mechanics in the frame of potential scattering phenomena. [15]

The shape resonances arise from the quantum interference between closed and an open scattering channels. At the resonance energy a quasi bound state is degenerate with a continuum. This quantum interference in many body system has been described using quantum mechanics by Gregor Wentzel, for the interpretation of the Auger effect, by Ettore Majorana for the dissociation processes and quasi bound states, by Ugo Fano for the atomic auto-ionization states in the continuum of helium atomic spectrum and by Victor Frederick Weisskopf. J. M. Blatt and Herman Feshbach for nuclear scattering experiments. [16]

The shape resonances are related with the existence of nearly stable bound states (that is, resonances) of two objects that dramatically influences how those two objects interact when their total energy is near that of the bound state. When the total energy of the objects is close to the energy of the resonance they interact strongly, and their scattering cross-section becomes very large.

A particular type of "shape resonance" occurs in multiband or two-band superconducting heterostructures at atomic limit called superstripes due to quantum interference of a first pairing channel in a first wide band and a second pairing channel in a second band where the chemical potential is tuned near a Lifshitz transition at the band edge or at the topological electronic transitions of the Fermi surface type "neck-collapsing" or "neck-disrupting" [17]

See also

Related Research Articles

<span class="mw-page-title-main">BCS theory</span> Microscopic theory of superconductivity

In physics, theBardeen–Cooper–Schrieffer (BCS) theory is the first microscopic theory of superconductivity since Heike Kamerlingh Onnes's 1911 discovery. The theory describes superconductivity as a microscopic effect caused by a condensation of Cooper pairs. The theory is also used in nuclear physics to describe the pairing interaction between nucleons in an atomic nucleus.

<span class="mw-page-title-main">Condensed matter physics</span> Branch of physics

Condensed matter physics is the field of physics that deals with the macroscopic and microscopic physical properties of matter, especially the solid and liquid phases, that arise from electromagnetic forces between atoms and electrons. More generally, the subject deals with condensed phases of matter: systems of many constituents with strong interactions among them. More exotic condensed phases include the superconducting phase exhibited by certain materials at extremely low cryogenic temperatures, the ferromagnetic and antiferromagnetic phases of spins on crystal lattices of atoms, the Bose–Einstein condensates found in ultracold atomic systems, and liquid crystals. Condensed matter physicists seek to understand the behavior of these phases by experiments to measure various material properties, and by applying the physical laws of quantum mechanics, electromagnetism, statistical mechanics, and other physics theories to develop mathematical models and predict the properties of extremely large groups of atoms.

<span class="mw-page-title-main">Ionization</span> Process by which atoms or molecules acquire charge by gaining or losing electrons

Ionization is the process by which an atom or a molecule acquires a negative or positive charge by gaining or losing electrons, often in conjunction with other chemical changes. The resulting electrically charged atom or molecule is called an ion. Ionization can result from the loss of an electron after collisions with subatomic particles, collisions with other atoms, molecules, electrons, positrons, protons, antiprotons and ions, or through the interaction with electromagnetic radiation. Heterolytic bond cleavage and heterolytic substitution reactions can result in the formation of ion pairs. Ionization can occur through radioactive decay by the internal conversion process, in which an excited nucleus transfers its energy to one of the inner-shell electrons causing it to be ejected.

In condensed matter physics, a Cooper pair or BCS pair is a pair of electrons bound together at low temperatures in a certain manner first described in 1956 by American physicist Leon Cooper.

In physics, quantum tunnelling, barrier penetration, or simply tunnelling is a quantum mechanical phenomenon in which an object such as an electron or atom passes through a potential energy barrier that, according to classical mechanics, should not be passable due to the object not having sufficient energy to pass or surmount the barrier.

<span class="mw-page-title-main">Molecular physics</span> Study of the physical and chemical properties of molecules

Molecular physics is the study of the physical properties of molecules and molecular dynamics. The field overlaps significantly with physical chemistry, chemical physics, and quantum chemistry. It is often considered as a sub-field of atomic, molecular, and optical physics. Research groups studying molecular physics are typically designated as one of these other fields. Molecular physics addresses phenomena due to both molecular structure and individual atomic processes within molecules. Like atomic physics, it relies on a combination of classical and quantum mechanics to describe interactions between electromagnetic radiation and matter. Experiments in the field often rely heavily on techniques borrowed from atomic physics, such as spectroscopy and scattering.

<span class="mw-page-title-main">X-ray absorption fine structure</span> Specific structure observed in X-ray absorption spectroscopy

X-ray absorption fine structure (XAFS) is a specific structure observed in X-ray absorption spectroscopy (XAS). By analyzing the XAFS, information can be acquired on the local structure and on the unoccupied local electronic states.

In physics, a Feshbach resonance can occur upon collision of two slow atoms, when they temporarily stick together forming an unstable compound with short lifetime. It is a feature of many-body systems in which a bound state is achieved if the coupling(s) between at least one internal degree of freedom and the reaction coordinates, which lead to dissociation, vanish. The opposite situation, when a bound state is not formed, is a shape resonance. It is named after Herman Feshbach, a physicist at MIT.

<span class="mw-page-title-main">Fano resonance</span> Type of scattering resonance

In physics, a Fano resonance is a type of resonant scattering phenomenon that gives rise to an asymmetric line-shape. Interference between a background and a resonant scattering process produces the asymmetric line-shape. It is named after Italian-American physicist Ugo Fano, who in 1961 gave a theoretical explanation for the scattering line-shape of inelastic scattering of electrons from helium; however, Ettore Majorana was the first to discover this phenomenon. Fano resonance is a weak coupling effect meaning that the decay rate is so high, that no hybridization occurs. The coupling modifies the resonance properties such as spectral position and width and its line-shape takes on the distinctive asymmetric Fano profile. Because it is a general wave phenomenon, examples can be found across many areas of physics and engineering.

<span class="mw-page-title-main">Majorana fermion</span> Fermion that is its own antiparticle

A Majorana fermion, also referred to as a Majorana particle, is a fermion that is its own antiparticle. They were hypothesised by Ettore Majorana in 1937. The term is sometimes used in opposition to a Dirac fermion, which describes fermions that are not their own antiparticles.

The term R-matrix has several meanings, depending on the field of study.

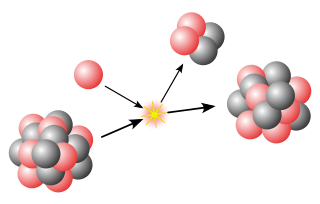
<span class="mw-page-title-main">Nuclear structure</span> Structure of the atomic nucleus

Understanding the structure of the atomic nucleus is one of the central challenges in nuclear physics.

Delbrück scattering, the deflection of high-energy photons in the Coulomb field of nuclei as a consequence of vacuum polarization, was observed in 1975. The related process of the scattering of light by light, also a consequence of vacuum polarization, was not observed until 1998. In both cases, it is a process described by quantum electrodynamics.

The timeline of quantum mechanics is a list of key events in the history of quantum mechanics, quantum field theories and quantum chemistry.

Superstripes is a generic name for a phase with spatial broken symmetry that favors the onset of superconducting or superfluid quantum order. This scenario emerged in the 1990s when non-homogeneous metallic heterostructures at the atomic limit with a broken spatial symmetry have been found to favor superconductivity. Before a broken spatial symmetry was expected to compete and suppress the superconducting order. The driving mechanism for the amplification of the superconductivity critical temperature in superstripes matter has been proposed to be the shape resonance in the energy gap parameters ∆n that is a type of Fano resonance for coexisting condensates.

<span class="mw-page-title-main">Ratko Janev</span> Macedonian atomic physicist

Ratko Janev was a Yugoslav and Serbian atomic physicist and Macedonian academician.

<span class="mw-page-title-main">Discovery of the neutron</span> Scientific background leading to the discovery of subatomic particles

The discovery of the neutron and its properties was central to the extraordinary developments in atomic physics in the first half of the 20th century. Early in the century, Ernest Rutherford developed a crude model of the atom, based on the gold foil experiment of Hans Geiger and Ernest Marsden. In this model, atoms had their mass and positive electric charge concentrated in a very small nucleus. By 1920, isotopes of chemical elements had been discovered, the atomic masses had been determined to be (approximately) integer multiples of the mass of the hydrogen atom, and the atomic number had been identified as the charge on the nucleus. Throughout the 1920s, the nucleus was viewed as composed of combinations of protons and electrons, the two elementary particles known at the time, but that model presented several experimental and theoretical contradictions.

The nucleon magnetic moments are the intrinsic magnetic dipole moments of the proton and neutron, symbols μp and μn. The nucleus of an atom comprises protons and neutrons, both nucleons that behave as small magnets. Their magnetic strengths are measured by their magnetic moments. The nucleons interact with normal matter through either the nuclear force or their magnetic moments, with the charged proton also interacting by the Coulomb force.

<span class="mw-page-title-main">Shape of the atomic nucleus</span>

The shape of the atomic nucleus depends on the variety of factors related to the size and shape of its nucleon constituents and the nuclear force holding them together. The origins of nuclear shape begin with the spacial extent of almost nearly all stable and a great many unstable nuclei has been determined mainly by electron and muon scattering experiments as well as spectroscopic experiments. An important factor in the internal structure of the nucleus is the nucleon-nucleon potential, which ultimately governs the distance between individual nucleons, while a dip in the charge density of some light nuclide structures a lesser density of nucleonic matter. A surprising non-spherical expectation for the shape of the nucleus originated in 1939 in the spectroscopic analysis of the quadrapole moments while the prolate spheroid shape of the nucleon arises from analysis of the intrinsic quadruple moment. The simple spherical approximation of nuclear size and shape provides at best a textbook introduction to nuclear size and shape. The unusual cosmic abundance of alpha nuclides has inspired geometric arrangements of alpha particles as a solution to nuclear shapes, although the atomic nucleus generally assumes a prolate spheroid shape. Nuclides can also be discus-shaped, triaxial or pear-shaped.

Leo Radzihovsky is a Russian American condensed matter physicist and academic serving as a professor of Distinction in Physics at the University of Colorado Boulder.

References

  1. ↑ Atomic, Molecular, and Optical Physics Panel on Atomic, Molecular, and Optical Physics Physics Survey Committee, Board on Physics and Astronomy, National Research Council, National Academic Press ISBN   978-0-309-07371-4
  2. ↑ cite A Generalized Quantum Defect Methods in Chemistry Altunata, PhD Thesis, MIT 2006 full text Archived 2011-06-05 at the Wayback Machine
  3. ↑ Schulz, George J. (1973-07-01). "Resonances in Electron Impact on Atoms" (PDF). Reviews of Modern Physics. 45 (3). American Physical Society (APS): 378–422. Bibcode:1973RvMP...45..378S. doi:10.1103/revmodphys.45.378. ISSN   0034-6861. Archived from the original (PDF) on 2008-09-24.
  4. ↑ Schulz, George J. (1973-07-01). "Resonances in Electron Impact on Diatomic Molecules" (PDF). Reviews of Modern Physics. 45 (3). American Physical Society (APS): 423–486. Bibcode:1973RvMP...45..423S. doi:10.1103/revmodphys.45.423. ISSN   0034-6861. Archived from the original (PDF) on 2008-09-24.
  5. ↑ Bianconi, Antonio (2003). Ugo Fano and shape resonances. X-ray and Inner Shell Processes (19th Int. Conference Roma June 24–28, 2002). Unsolved Problems of Noise and Fluctuations. Vol. 652. AIP. p. 13. arXiv: cond-mat/0211452 . doi:10.1063/1.1536357. ISSN   0094-243X.
  6. ↑ Vittorini-Orgeas, Alessandra; Bianconi, Antonio (2009-01-07). "From Majorana Theory of Atomic Autoionization to Feshbach Resonances in High Temperature Superconductors". Journal of Superconductivity and Novel Magnetism. 22 (3): 215–221. arXiv: 0812.1551 . doi:10.1007/s10948-008-0433-x. ISSN   1557-1939. S2CID   118439516.
  7. ↑ Combes, J. M.; Duclos, P.; Klein, M.; Seiler, R. (1987). "The shape resonance". Communications in Mathematical Physics. 110 (2). Springer Science and Business Media LLC: 215–236. Bibcode:1987CMaPh.110..215C. doi:10.1007/bf01207364. ISSN   0010-3616. S2CID   119536657.
  8. ↑ "Roles Played by Metastable States in Chemistry" Jack Simons in Resonances in Electron-Molecule Scattering Van Der Waals Complexes, and Reactive Chemical Dynamics Donald G. Truhlar, Ed, American Chemical Society Symposium Series, ACS No. 263 (1984)
  9. ↑ Zobel, J; Mayer, U; Jung, K; Ehrhardt, H; Pritchard, H; Winstead, C; McKoy, V (1996-02-28). "Absolute differential cross sections for electron-impact excitation of CO near threshold: II. The Rydberg states of CO" (PDF). Journal of Physics B: Atomic, Molecular and Optical Physics. 29 (4). IOP Publishing: 839–856. Bibcode:1996JPhB...29..839Z. doi:10.1088/0953-4075/29/4/022. ISSN   0953-4075. S2CID   250751335.
  10. ↑ Stock, René; Deutsch, Ivan H.; Bolda, Eric L. (2003-10-31). "Quantum State Control via Trap-Induced Shape Resonance in Ultracold Atomic Collisions". Physical Review Letters. 91 (18): 183201. arXiv: quant-ph/0304093 . Bibcode:2003PhRvL..91r3201S. doi:10.1103/physrevlett.91.183201. ISSN   0031-9007. PMID   14611281. S2CID   33876413.
  11. ↑ LBL Mol Spec Discussion
  12. ↑ Stolte, W. C.; Hansen, D. L.; Piancastelli, M. N.; Dominguez Lopez, I.; Rizvi, A.; et al. (2001-05-14). "Anionic Photofragmentation of CO: A Selective Probe of Core-Level Resonances". Physical Review Letters. 86 (20). American Physical Society (APS): 4504–4507. Bibcode:2001PhRvL..86.4504S. doi:10.1103/physrevlett.86.4504. ISSN   0031-9007. PMID   11384269.
  13. ↑ Nuclear Physics: Nuclear structure Amos de Shalit and Herman Feshbach John Wiley & Sons Inc, New York, page 87 (1974)
  14. ↑ Robley D. Evans The Atomic Nucleus McGraw-Hill Books, p. 448-450 and p. 455-456 (1955)
  15. ↑ J. J. Sakurai, Modern Quantum Mechanics Addison-Wesley Longman (2005) pag. 418-421 ISBN   7-5062-7314-4
  16. ↑ J. M. Blatt and V. F. Weisskopf Theoretical Nuclear Physics John Wiley & Sons, Inc., New York (1952)
  17. ↑ Innocenti, Davide; Poccia, Nicola; Ricci, Alessandro; Valletta, Antonio; Caprara, Sergio; et al. (2010-11-19). "Resonant and crossover phenomena in a multiband superconductor: Tuning the chemical potential near a band edge". Physical Review B. 82 (18). American Physical Society (APS): 184528. arXiv: 1007.0510 . Bibcode:2010PhRvB..82r4528I. doi:10.1103/physrevb.82.184528. ISSN   1098-0121. S2CID   119232655.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.