In mathematical finance, the stochastic volatility jump (SVJ) model is suggested by Bates. [1] This model fits the observed implied volatility surface well. The model is a Heston process for stochastic volatility with an added Merton log-normal jump. It assumes the following correlated processes:
where S is the price of security, μ is the constant drift (i.e. expected return), t represents time, Z1 is a standard Brownian Motion, q is a Poisson counter with density λ.
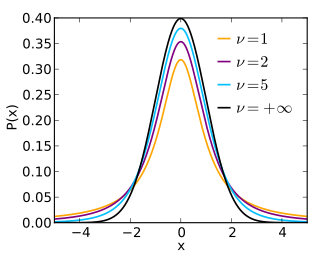
In probability and statistics, Student's t-distribution is any member of a family of continuous probability distributions that arise when estimating the mean of a normally distributed population in situations where the sample size is small and the population's standard deviation is unknown. It was developed by English statistician William Sealy Gosset under the pseudonym "Student".
A geometric Brownian motion (GBM) is a continuous-time stochastic process in which the logarithm of the randomly varying quantity follows a Brownian motion with drift. It is an important example of stochastic processes satisfying a stochastic differential equation (SDE); in particular, it is used in mathematical finance to model stock prices in the Black–Scholes model.
The Einstein–Hilbert action in general relativity is the action that yields the Einstein field equations through the principle of least action. With the (− + + +) metric signature, the gravitational part of the action is given as
A compound Poisson process is a continuous-time (random) stochastic process with jumps. The jumps arrive randomly according to a Poisson process and the size of the jumps is also random, with a specified probability distribution. A compound Poisson process, parameterised by a rate and jump size distribution G, is a process given by
Variational Bayesian methods are a family of techniques for approximating intractable integrals arising in Bayesian inference and machine learning. They are typically used in complex statistical models consisting of observed variables as well as unknown parameters and latent variables, with various sorts of relationships among the three types of random variables, as might be described by a graphical model. As typical in Bayesian inference, the parameters and latent variables are grouped together as "unobserved variables". Variational Bayesian methods are primarily used for two purposes:
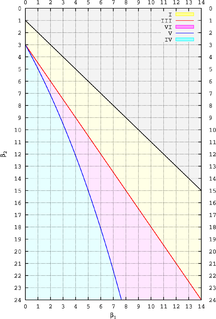
The Pearson distribution is a family of continuous probability distributions. It was first published by Karl Pearson in 1895 and subsequently extended by him in 1901 and 1916 in a series of articles on biostatistics.
In general relativity, a geodesic generalizes the notion of a "straight line" to curved spacetime. Importantly, the world line of a particle free from all external, non-gravitational forces is a particular type of geodesic. In other words, a freely moving or falling particle always moves along a geodesic.
In probability theory and statistics, the generalized inverse Gaussian distribution (GIG) is a three-parameter family of continuous probability distributions with probability density function
A gamma process in mathematics and probability theory, is a random process with independent gamma distributed increments. Often written as , it is a pure-jump increasing Lévy process with intensity measure for positive . Thus jumps whose size lies in the interval occur as a Poisson process with intensity The parameter controls the rate of jump arrivals and the scaling parameter inversely controls the jump size. It is assumed that the process starts from a value 0 at t = 0.
In physics, Maxwell's equations in curved spacetime govern the dynamics of the electromagnetic field in curved spacetime or where one uses an arbitrary coordinate system. These equations can be viewed as a generalization of the vacuum Maxwell's equations which are normally formulated in the local coordinates of flat spacetime. But because general relativity dictates that the presence of electromagnetic fields induce curvature in spacetime, Maxwell's equations in flat spacetime should be viewed as a convenient approximation.
Ellipsoidal coordinates are a three-dimensional orthogonal coordinate system that generalizes the two-dimensional elliptic coordinate system. Unlike most three-dimensional orthogonal coordinate systems that feature quadratic coordinate surfaces, the ellipsoidal coordinate system is based on confocal quadrics.
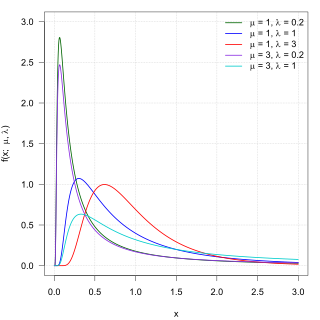
In probability theory, the inverse Gaussian distribution is a two-parameter family of continuous probability distributions with support on (0,∞).
In statistics, stochastic volatility models are those in which the variance of a stochastic process is itself randomly distributed. They are used in the field of mathematical finance to evaluate derivative securities, such as options. The name derives from the models' treatment of the underlying security's volatility as a random process, governed by state variables such as the price level of the underlying security, the tendency of volatility to revert to some long-run mean value, and the variance of the volatility process itself, among others.
In finance, a volatility swap is a forward contract on the future realised volatility of a given underlying asset. Volatility swaps allow investors to trade the volatility of an asset directly, much as they would trade a price index. Its payoff at expiration is equal to
In probability theory and statistics, the Conway–Maxwell–Poisson distribution is a discrete probability distribution named after Richard W. Conway, William L. Maxwell, and Siméon Denis Poisson that generalizes the Poisson distribution by adding a parameter to model overdispersion and underdispersion. It is a member of the exponential family, has the Poisson distribution and geometric distribution as special cases and the Bernoulli distribution as a limiting case.
Financial models with long-tailed distributions and volatility clustering have been introduced to overcome problems with the realism of classical financial models. These classical models of financial time series typically assume homoskedasticity and normality cannot explain stylized phenomena such as skewness, heavy tails, and volatility clustering of the empirical asset returns in finance. In 1963, Benoit Mandelbrot first used the stable distribution to model the empirical distributions which have the skewness and heavy-tail property. Since -stable distributions have infinite -th moments for all , the tempered stable processes have been proposed for overcoming this limitation of the stable distribution.
In probability theory and statistics, the generalized multivariate log-gamma (G-MVLG) distribution is a multivariate distribution introduced by Demirhan and Hamurkaroglu in 2011. The G-MVLG is a flexible distribution. Skewness and kurtosis are well controlled by the parameters of the distribution. This enables one to control dispersion of the distribution. Because of this property, the distribution is effectively used as a joint prior distribution in Bayesian analysis, especially when the likelihood is not from the location-scale family of distributions such as normal distribution.
In probability theory, the stable count distribution is the conjugate prior of a one-sided stable distribution. This distribution was discovered by Stephen Lihn in his 2017 study of daily distributions of the S&P 500 and the VIX. The stable distribution family is also sometimes referred to as the Lévy alpha-stable distribution, after Paul Lévy, the first mathematician to have studied it.
In finance, an option on realized variance is a type of variance derivatives which is the derivative securities on which the payoff depends on the annualized realized variance of the return of a specified underlying asset, e.g. stock index, bond, exchange rate, etc. Another liquidated security of the same type is variance swap, which is, in other words, the futures contract on realized variance.
In finance, option on realized volatility is a subclass of derivatives securities that the payoff function embedded with the notion of annualized realized volatility of a specified underlying asset, which could be stock index, bond, foreign exchange rate, etc. Another product of volatility derivative that is widely traded refers to the volatility swap, which is in another word the forward contract on future realized volatility.
 | This Econometrics-related article is a stub. You can help Wikipedia by expanding it. |
