Strong measure zero set

Last updated

In mathematical analysis, a strong measure zero set [1] is a subset A of the real line with the following property:

for every sequence (εn) of positive reals there exists a sequence (In) of intervals such that |In| < εn for all n and A is contained in the union of the In.

(Here |In| denotes the length of the interval In.)

Every countable set is a strong measure zero set, and so is every union of countably many strong measure zero sets. Every strong measure zero set has Lebesgue measure 0. The Cantor set is an example of an uncountable set of Lebesgue measure 0 which is not of strong measure zero. [2]

Borel's conjecture [1] states that every strong measure zero set is countable. It is now known that this statement is independent of ZFC (the Zermelo–Fraenkel axioms of set theory, which is the standard axiom system assumed in mathematics). This means that Borel's conjecture can neither be proven nor disproven in ZFC (assuming ZFC is consistent). Sierpiński proved in 1928 that the continuum hypothesis (which is now also known to be independent of ZFC) implies the existence of uncountable strong measure zero sets. [3] In 1976 Laver used a method of forcing to construct a model of ZFC in which Borel's conjecture holds. [4] These two results together establish the independence of Borel's conjecture.

The following characterization of strong measure zero sets was proved in 1973:

A set A ⊆ R has strong measure zero if and only if A + M ≠ R for every meagre set M ⊆ R. [5]

This result establishes a connection to the notion of strongly meagre set, defined as follows:

A set M ⊆ R is strongly meagre if and only if A + M ≠ R for every set A ⊆ R of Lebesgue measure zero.

The dual Borel conjecture states that every strongly meagre set is countable. This statement is also independent of ZFC. [6]

Related Research Articles

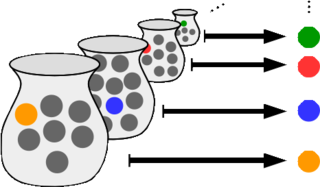
Axiom of choice axiom of set theory

In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that a Cartesian product of a collection of non-empty sets is non-empty. Informally put, the axiom of choice says that given any collection of bins, each containing at least one object, it is possible to make a selection of exactly one object from each bin, even if the collection is infinite. Formally, it states that for every indexed family of nonempty sets there exists an indexed family of elements such that for every . The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem.

In measure theory, a branch of mathematics, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of n-dimensional Euclidean space. For n = 1, 2, or 3, it coincides with the standard measure of length, area, or volume. In general, it is also called n-dimensional volume, n-volume, or simply volume. It is used throughout real analysis, in particular to define Lebesgue integration. Sets that can be assigned a Lebesgue measure are called Lebesgue-measurable; the measure of the Lebesgue-measurable set A is here denoted by λ(A).

In mathematical analysis, a null set is a measurable set that has measure zero. This can be characterized as a set that can be covered by a countable union of intervals of arbitrarily small total length.

In mathematics, a topological space is called separable if it contains a countable, dense subset; that is, there exists a sequence of elements of the space such that every nonempty open subset of the space contains at least one element of the sequence.

In mathematics, a well-order on a set S is a total order on S with the property that every non-empty subset of S has a least element in this ordering. The set S together with the well-order relation is then called a well-ordered set. In some academic articles and textbooks these terms are instead written as wellorder, wellordered, and wellordering or well order, well ordered, and well ordering.

In mathematics, a Borel set is any set in a topological space that can be formed from open sets through the operations of countable union, countable intersection, and relative complement. Borel sets are named after Émile Borel.

In mathematics, Suslin's problem is a question about totally ordered sets posed by Mikhail Yakovlevich Suslin (1920) and published posthumously. It has been shown to be independent of the standard axiomatic system of set theory known as ZFC: Solovay & Tennenbaum (1971) showed that the statement can neither be proven nor disproven from those axioms, assuming ZF is consistent.

Aleph number Infinite cardinal number

In mathematics, particularly in set theory, the aleph numbers are a sequence of numbers used to represent the cardinality of infinite sets that can be well-ordered. They were introduced by the mathematician Georg Cantor and are named after the symbol he used to denote them, the Hebrew letter aleph.

In the mathematical discipline of general topology, a Polish space is a separable completely metrizable topological space; that is, a space homeomorphic to a complete metric space that has a countable dense subset. Polish spaces are so named because they were first extensively studied by Polish topologists and logicians—Sierpiński, Kuratowski, Tarski and others. However, Polish spaces are mostly studied today because they are the primary setting for descriptive set theory, including the study of Borel equivalence relations. Polish spaces are also a convenient setting for more advanced measure theory, in particular in probability theory.

In mathematics, a non-measurable set is a set which cannot be assigned a meaningful "volume". The mathematical existence of such sets is construed to provide information about the notions of length, area and volume in formal set theory. In Zermelo–Fraenkel set theory, the axiom of choice entails that non-measurable subsets of exist.

<i>Counterexamples in Topology</i> Book by Lynn Steen

Counterexamples in Topology is a book on mathematics by topologists Lynn Steen and J. Arthur Seebach, Jr.

In the mathematical field of set theory, Martin's axiom, introduced by Donald A. Martin and Robert M. Solovay, is a statement that is independent of the usual axioms of ZFC set theory. It is implied by the continuum hypothesis, but it is consistent with ZFC and the negation of the continuum hypothesis. Informally, it says that all cardinals less than the cardinality of the continuum, , behave roughly like . The intuition behind this can be understood by studying the proof of the Rasiowa–Sikorski lemma. It is a principle that is used to control certain forcing arguments.

In mathematics, set-theoretic topology is a subject that combines set theory and general topology. It focuses on topological questions that are independent of Zermelo–Fraenkel set theory (ZFC).

Mikhail Yakovlevich Suslin was a Russian mathematician who made major contributions to the fields of general topology and descriptive set theory.

In mathematics, specifically geometric topology, the Borel conjecture asserts that an aspherical closed manifold is determined by its fundamental group, up to homeomorphism. It is a rigidity conjecture, asserting that a weak, algebraic notion of equivalence should imply a stronger, topological notion.

In mathematics, a Luzin space (or Lusin space), named for N. N. Luzin, is an uncountable topological T1 space without isolated points in which every nowhere-dense subset is countable. There are many minor variations of this definition in use: the T1 condition can be replaced by T2 or T3, and some authors allow a countable or even arbitrary number of isolated points.

In the mathematical field of set theory, the Solovay model is a model constructed by Robert M. Solovay (1970) in which all of the axioms of Zermelo–Fraenkel set theory (ZF) hold, exclusive of the axiom of choice, but in which all sets of real numbers are Lebesgue measurable. The construction relies on the existence of an inaccessible cardinal.

This is a glossary of set theory.

In mathematics, a Sierpiński set is an uncountable subset of a real vector space whose intersection with every measure-zero set is countable. The existence of Sierpiński sets is independent of the axioms of ZFC. Sierpiński (1924) showed that they exist if the continuum hypothesis is true. On the other hand, they do not exist if Martin's axiom for ℵ1 is true. Sierpiński sets are weakly Luzin sets but are not Luzin sets.

References

  1. 1 2 Borel, Émile (1919). "Sur la classification des ensembles de mesure nulle" (PDF). Bull. Soc. Math. France. 47: 97–125. doi: 10.24033/bsmf.996 .
  2. ↑ Jech, Thomas (2003). Set Theory: The Third Millennium Edition, Revised and Expanded. Springer Monographs in Mathematics (3rd ed.). Springer. p. 539. ISBN   978-3540440857.
  3. ↑ Sierpiński, W. (1928). "Sur un ensemble non denombrable, dont toute image continue est de mesure nulle" (PDF). Fundamenta Mathematicae (in French). 11 (1): 302–4. doi: 10.4064/fm-11-1-302-303 .
  4. ↑ Laver, Richard (1976). "On the consistency of Borel's conjecture". Acta Math. 137 (1): 151–169. doi: 10.1007/BF02392416 .
  5. ↑ Galvin, F.; Mycielski, J.; Solovay, R.M. (1973). "Strong measure zero sets". Notices of the American Mathematical Society. 26.
  6. ↑ Carlson, Timothy J. (1993). "Strong measure zero and strongly meager sets". Proc. Amer. Math. Soc. 118 (2): 577–586. doi: 10.1090/s0002-9939-1993-1139474-6 . JSTOR   2160341.
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.