Related Research Articles

John von Neumann was a Hungarian-American mathematician, physicist, computer scientist, engineer and polymath. He was regarded as having perhaps the widest coverage of any mathematician of his time and was said to have been "the last representative of the great mathematicians who were equally at home in both pure and applied mathematics". He integrated pure and applied sciences.

Linear algebra is the branch of mathematics concerning linear equations such as:
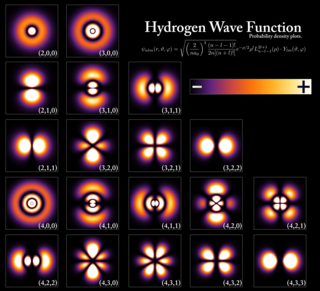
Quantum mechanics is a fundamental theory in physics that provides a description of the physical properties of nature at the scale of atoms and subatomic particles. It is the foundation of all quantum physics including quantum chemistry, quantum field theory, quantum technology, and quantum information science.

Mathematical physics refers to the development of mathematical methods for application to problems in physics. The Journal of Mathematical Physics defines the field as "the application of mathematics to problems in physics and the development of mathematical methods suitable for such applications and for the formulation of physical theories". An alternative definition would also include those mathematics that are inspired by physics.
In physics, an observable is a physical quantity that can be measured. Examples include position and momentum. In systems governed by classical mechanics, it is a real-valued "function" on the set of all possible system states. In quantum physics, it is an operator, or gauge, where the property of the quantum state can be determined by some sequence of operations. For example, these operations might involve submitting the system to various electromagnetic fields and eventually reading a value.
Noncommutative geometry (NCG) is a branch of mathematics concerned with a geometric approach to noncommutative algebras, and with the construction of spaces that are locally presented by noncommutative algebras of functions. A noncommutative algebra is an associative algebra in which the multiplication is not commutative, that is, for which does not always equal ; or more generally an algebraic structure in which one of the principal binary operations is not commutative; one also allows additional structures, e.g. topology or norm, to be possibly carried by the noncommutative algebra of functions.

Paul Richard Halmos was a Hungarian-born American mathematician and statistician who made fundamental advances in the areas of mathematical logic, probability theory, statistics, operator theory, ergodic theory, and functional analysis. He was also recognized as a great mathematical expositor. He has been described as one of The Martians.

In functional analysis, a branch of mathematics, an operator algebra is an algebra of continuous linear operators on a topological vector space, with the multiplication given by the composition of mappings.
In mathematics, spectral theory is an inclusive term for theories extending the eigenvector and eigenvalue theory of a single square matrix to a much broader theory of the structure of operators in a variety of mathematical spaces. It is a result of studies of linear algebra and the solutions of systems of linear equations and their generalizations. The theory is connected to that of analytic functions because the spectral properties of an operator are related to analytic functions of the spectral parameter.
In quantum physics, a measurement is the testing or manipulation of a physical system to yield a numerical result. The predictions that quantum physics makes are in general probabilistic. The mathematical tools for making predictions about what measurement outcomes may occur were developed during the 20th century and make use of linear algebra and functional analysis.
In mathematical physics, Gleason's theorem shows that the rule one uses to calculate probabilities in quantum physics, the Born rule, can be derived from the usual mathematical representation of measurements in quantum physics together with the assumption of non-contextuality. Andrew M. Gleason first proved the theorem in 1957, answering a question posed by George W. Mackey, an accomplishment that was historically significant for the role it played in showing that wide classes of hidden-variable theories are inconsistent with quantum physics. Multiple variations have been proven in the years since. Gleason's theorem is of particular importance for the field of quantum logic and its attempt to find a minimal set of mathematical axioms for quantum theory.

Ronald George Douglas was an American mathematician, best known for his work on operator theory and operator algebras.
Hilbert C*-modules are mathematical objects that generalise the notion of a Hilbert space, in that they endow a linear space with an "inner product" that takes values in a C*-algebra. Hilbert C*-modules were first introduced in the work of Irving Kaplansky in 1953, which developed the theory for commutative, unital algebras. In the 1970s the theory was extended to non-commutative C*-algebras independently by William Lindall Paschke and Marc Rieffel, the latter in a paper that used Hilbert C*-modules to construct a theory of induced representations of C*-algebras. Hilbert C*-modules are crucial to Kasparov's formulation of KK-theory, and provide the right framework to extend the notion of Morita equivalence to C*-algebras. They can be viewed as the generalization of vector bundles to noncommutative C*-algebras and as such play an important role in noncommutative geometry, notably in C*-algebraic quantum group theory, and groupoid C*-algebras.
In mathematics, especially (higher) category theory, higher-dimensional algebra is the study of categorified structures. It has applications in nonabelian algebraic topology, and generalizes abstract algebra.

In mathematics, Hilbert spaces allow generalizing the methods of linear algebra and calculus from (finite-dimensional) Euclidean vector spaces to spaces that may be infinite-dimensional. Hilbert spaces arise naturally and frequently in mathematics and physics, typically as function spaces. Formally, a Hilbert space is a vector space equipped with an inner product that defines a distance function for which the space is a complete metric space.
In functional analysis, a discipline within mathematics, an operator space is a normed vector space "given together with an isometric embedding into the space B(H) of all bounded operators on a Hilbert space H.". The appropriate morphisms between operator spaces are completely bounded maps.
In physics, quantum dynamics is the quantum version of classical dynamics. Quantum dynamics deals with the motions, and energy and momentum exchanges of systems whose behavior is governed by the laws of quantum mechanics. Quantum dynamics is relevant for burgeoning fields, such as quantum computing and atomic optics.

Gilles I. Pisier is a professor of mathematics at the Pierre and Marie Curie University and a distinguished professor and A.G. and M.E. Owen Chair of Mathematics at the Texas A&M University. He is known for his contributions to several fields of mathematics, including functional analysis, probability theory, harmonic analysis, and operator theory. He has also made fundamental contributions to the theory of C*-algebras. Gilles is the younger brother of French actress Marie-France Pisier.

Kalyan Bidhan Sinha is an Indian mathematician. He is a professor at the Jawaharlal Nehru Centre for Advanced Scientific Research, and Professor Emeritus for life of the Indian Statistical Institute.

Mohammad Sal Moslehian. is an Iranian mathematician and a professor of mathematics at Ferdowsi University of Mashhad, Iran. He is the President of the Iranian Mathematical Society for the period of 2021-2024 and an invited member of the Iranian Academy of Sciences. His Erdős number is 3. He is known for his contribution to the operator and norm inequality. He has developed the orthogonality in Hilbert C*-modules and has significant contributions to operator means. He established noncommutative versions of martingale and maximum inequalities that play an essential role in noncommutative probability spaces. In addition, he has written several expository papers discussing research and education, as well as promoting mathematics.
References
- ↑ "Vern Paulsen". uh.edu. Retrieved May 10, 2017.
- ↑ "Vern Paulsen". uwaterloo.ca. 2 July 2015. Retrieved May 10, 2017.
- ↑ "Vern Paulsen - IQC People". Institute for Quantum Computing. Retrieved 2019-03-15.