Related Research Articles

The Fahrenheit scale is a temperature scale based on one proposed in 1724 by the European physicist Daniel Gabriel Fahrenheit (1686–1736). It uses the degree Fahrenheit as the unit. Several accounts of how he originally defined his scale exist, but the original paper suggests the lower defining point, 0 °F, was established as the freezing temperature of a solution of brine made from a mixture of water, ice, and ammonium chloride. The other limit established was his best estimate of the average human body temperature, originally set at 90 °F, then 96 °F.

The North Pole, also known as the Geographic North Pole, Terrestrial North Pole or 90th Parallel North, is the point in the Northern Hemisphere where the Earth's axis of rotation meets its surface. It is called the True North Pole to distinguish from the Magnetic North Pole.
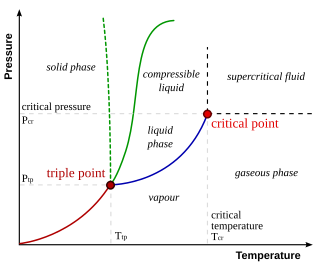
In thermodynamics, the triple point of a substance is the temperature and pressure at which the three phases of that substance coexist in thermodynamic equilibrium. It is that temperature and pressure at which the sublimation, fusion, and vaporisation curves meet. For example, the triple point of mercury occurs at a temperature of −38.8 °C (−37.8 °F) and a pressure of 0.165 mPa.

A fathom is a unit of length in the imperial and the U.S. customary systems equal to 6 feet (1.8288 m), used especially for measuring the depth of water. The fathom is neither an international standard (SI) unit, nor an internationally accepted non-SI unit. Historically it was the maritime measure of depth in the English-speaking world but, apart from within the US, charts now use metres.

In mathematics, a ball is the solid figure bounded by a sphere; it is also called a solid sphere. It may be a closed ball or an open ball.

The Rockwell scale is a hardness scale based on indentation hardness of a material. The Rockwell test measures the depth of penetration of an indenter under a large load compared to the penetration made by a preload. There are different scales, denoted by a single letter, that use different loads or indenters. The result is a dimensionless number noted as HRA, HRB, HRC, etc., where the last letter is the respective Rockwell scale. Larger numbers correspond to harder materials.
In mathematics, a fractal dimension is a term invoked in the science of geometry to provide a rational statistical index of complexity detail in a pattern. A fractal pattern changes with the scale at which it is measured. It is also a measure of the space-filling capacity of a pattern, and it tells how a fractal scales differently, in a fractal (non-integer) dimension.

The Cavendish experiment, performed in 1797–1798 by English scientist Henry Cavendish, was the first experiment to measure the force of gravity between masses in the laboratory and the first to yield accurate values for the gravitational constant. Because of the unit conventions then in use, the gravitational constant does not appear explicitly in Cavendish's work. Instead, the result was originally expressed as the specific gravity of Earth, or equivalently the mass of Earth. His experiment gave the first accurate values for these geophysical constants.

In fractal geometry, the Minkowski–Bouligand dimension, also known as Minkowski dimension or box-counting dimension, is a way of determining the fractal dimension of a set in a Euclidean space , or more generally in a metric space . It is named after the Polish mathematician Hermann Minkowski and the French mathematician Georges Bouligand.

A mill is a device, often a structure, machine or kitchen appliance, that breaks solid materials into smaller pieces by grinding, crushing, or cutting. Such comminution is an important unit operation in many processes. There are many different types of mills and many types of materials processed in them. Historically mills were powered by hand or by animals, working animal, wind (windmill) or water (watermill). In modern era, they are usually powered by electricity.

Pool is a classification of cue sports played on a table with six pockets along the rails, into which balls are shot. Each specific pool game has its own name; some of the better-known include eight-ball, blackball, nine-ball, ten-ball, seven-ball, straight pool, one-pocket, and bank pool. Eight-ball is the most frequently played discipline of pool, and is often thought of as synonymous with "pool".

The gauge of a firearm is a unit of measurement used to express the inner diameter of the barrel.

Seed balls, also known as earth balls or nendo dango, consist of seeds rolled within a ball of clay and other matter to assist germination. They are then thrown into vacant lots and over fences as a form of 'guerilla gardening'. Matter such as humus and compost are often placed around the seeds to provide microbial inoculants. Cotton-fibres or liquefied paper are sometimes added to further protect the clay ball in particularly harsh habitats. An ancient technique, it was re-discovered by Japanese natural farming pioneer Masanobu Fukuoka.

Fish balls are balls made from fish paste which are then boiled or deep-fried. Similar in composition to fishcake, fish balls are often made from fish mince or surimi, salt, and a culinary binder such as tapioca flour, corn, or potato starch.
Chicago is a "money ball" pool gambling game.

The surface-area-to-volume ratio or surface-to-volume ratio is the ratio between surface area and volume of an object or collection of objects.
Temperature is a physical quantity that quantitatively expresses the attribute of hotness or coldness. Temperature is measured with a thermometer. It reflects the kinetic energy of the vibrating and colliding atoms making up a substance.
The angstrom or ångström is a metric unit of length equal to 10−10 m; that is, one ten-billionth (US) of a metre, a hundred-millionth of a centimetre, 0.1 nanometre, or 100 picometres. Its symbol is Å, a letter of the Swedish alphabet. The unit is named after the Swedish physicist Anders Jonas Ångström (1814–1874).
In mathematics, a unit sphere is a sphere of unit radius: the set of points at Euclidean distance 1 from some center point in three-dimensional space. More generally, the unit -sphere is an -sphere of unit radius in -dimensional Euclidean space; the unit circle is a special case, the unit -sphere in the plane. An (open) unit ball is the region inside of a unit sphere, the set of points of distance less than 1 from the center.
References
- ↑ Joseph, Christopher (2022-04-12). How to Measure Anything. London: Ivy Press. p. 40. ISBN 978-0-7112-6803-6.
- 1 2 Jerrard, H. G. (2013-03-09). A Dictionary of Scientific Units. Springer Science & Business Media. p. 165. ISBN 978-94-017-0571-4.
- ↑ Fairhall, David (1971). Russian Sea Power. Boston: Boston : Gambit. p. 37. ISBN 978-0-87645-040-6.
- 1 2 Gyllenbok, Jan (2018-04-11). Encyclopaedia of Historical Metrology, Weights, and Measures. Cham: Birkhäuser. p. 237. ISBN 978-3-319-57598-8.