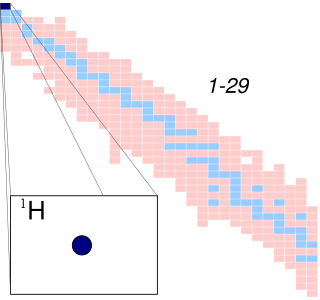
A hydrogen atom is an atom of the chemical element hydrogen. The electrically neutral atom contains a single positively charged proton and a single negatively charged electron bound to the nucleus by the Coulomb force. Atomic hydrogen constitutes about 75% of the baryonic mass of the universe.
The Schrödinger equation is a linear partial differential equation that governs the wave function of a quantum-mechanical system. It is a key result in quantum mechanics, and its discovery was a significant landmark in the development of the subject. The equation is named after Erwin Schrödinger, who postulated the equation in 1925, and published it in 1926, forming the basis for the work that resulted in his Nobel Prize in Physics in 1933.
In quantum chemistry and molecular physics, the Born–Oppenheimer (BO) approximation is the best-known mathematical approximation in molecular dynamics. Specifically, it is the assumption that the wave functions of atomic nuclei and electrons in a molecule can be treated separately, based on the fact that the nuclei are much heavier than the electrons. Due to the larger relative mass of a nucleus compared to an electron, the coordinates of the nuclei in a system are approximated as fixed, while the coordinates of the electrons are dynamic. The approach is named after Max Born and J. Robert Oppenheimer who proposed it in 1927, in the early period of quantum mechanics.

An instanton is a notion appearing in theoretical and mathematical physics. An instanton is a classical solution to equations of motion with a finite, non-zero action, either in quantum mechanics or in quantum field theory. More precisely, it is a solution to the equations of motion of the classical field theory on a Euclidean spacetime.
In quantum mechanics, a particle in a spherically symmetric potential, is a quantum system with a potential that depends only on the distance between the particle and a defined center point. One example of a spherically symmetric potential is the electron within a hydrogen atom. The electron's potential only depends on its distance from the proton in the atom's nucleus. This potential can be derived from Coulomb's law.
In particle, atomic and condensed matter physics, a Yukawa potential is a potential named after the Japanese physicist Hideki Yukawa. The potential is of the form:
In mathematical physics, the WKB approximation or WKB method is a method for finding approximate solutions to linear differential equations with spatially varying coefficients. It is typically used for a semiclassical calculation in quantum mechanics in which the wavefunction is recast as an exponential function, semiclassically expanded, and then either the amplitude or the phase is taken to be changing slowly.
In quantum mechanics, Bargmann's limit, named for Valentine Bargmann, provides an upper bound on the number of bound states with azimuthal quantum number in a system with central potential . It takes the form
In quantum physics, Regge theory is the study of the analytic properties of scattering as a function of angular momentum, where the angular momentum is not restricted to be an integer multiple of ħ but is allowed to take any complex value. The nonrelativistic theory was developed by Tullio Regge in 1959.
The Schrödinger–Newton equation, sometimes referred to as the Newton–Schrödinger or Schrödinger–Poisson equation, is a nonlinear modification of the Schrödinger equation with a Newtonian gravitational potential, where the gravitational potential emerges from the treatment of the wave function as a mass density, including a term that represents interaction of a particle with its own gravitational field. The inclusion of a self-interaction term represents a fundamental alteration of quantum mechanics. It can be written either as a single integro-differential equation or as a coupled system of a Schrödinger and a Poisson equation. In the latter case it is also referred to in the plural form.
In atomic, molecular, and optical physics and quantum chemistry, the molecular Hamiltonian is the Hamiltonian operator representing the energy of the electrons and nuclei in a molecule. This operator and the associated Schrödinger equation play a central role in computational chemistry and physics for computing properties of molecules and aggregates of molecules, such as thermal conductivity, specific heat, electrical conductivity, optical, and magnetic properties, and reactivity.
The Gross–Pitaevskii equation describes the ground state of a quantum system of identical bosons using the Hartree–Fock approximation and the pseudopotential interaction model.
The quantum potential or quantum potentiality is a central concept of the de Broglie–Bohm formulation of quantum mechanics, introduced by David Bohm in 1952.
The Einstein–Brillouin–Keller method (EBK) is a semiclassical method used to compute eigenvalues in quantum-mechanical systems. EBK quantization is an improvement from Bohr-Sommerfeld quantization which did not consider the caustic phase jumps at classical turning points. This procedure is able to reproduce exactly the spectrum of the 3D harmonic oscillator, particle in a box, and even the relativistic fine structure of the hydrogen atom.
A hydrogen-like atom (or hydrogenic atom) is any atom or ion with a single valence electron. These atoms are isoelectronic with hydrogen. Examples of hydrogen-like atoms include, but are not limited to, hydrogen itself, all alkali metals such as Rb and Cs, singly ionized alkaline earth metals such as Ca+ and Sr+ and other ions such as He+, Li2+, and Be3+ and isotopes of any of the above. A hydrogen-like atom includes a positively charged core consisting of the atomic nucleus and any core electrons as well as a single valence electron. Because helium is common in the universe, the spectroscopy of singly ionized helium is important in EUV astronomy, for example, of DO white dwarf stars.
In theoretical physics, the eikonal approximation is an approximative method useful in wave scattering equations which occur in optics, seismology, quantum mechanics, quantum electrodynamics, and partial wave expansion.
In solid-state physics, the k·p perturbation theory is an approximated semi-empirical approach for calculating the band structure and optical properties of crystalline solids. It is pronounced "k dot p", and is also called the "k·p method". This theory has been applied specifically in the framework of the Luttinger–Kohn model, and of the Kane model.
The Monte Carlo method for electron transport is a semiclassical Monte Carlo (MC) approach of modeling semiconductor transport. Assuming the carrier motion consists of free flights interrupted by scattering mechanisms, a computer is utilized to simulate the trajectories of particles as they move across the device under the influence of an electric field using classical mechanics. The scattering events and the duration of particle flight is determined through the use of random numbers.
The so-called double-well potential is one of a number of quartic potentials of considerable interest in quantum mechanics, in quantum field theory and elsewhere for the exploration of various physical phenomena or mathematical properties since it permits in many cases explicit calculation without over-simplification.