Leonid Bunimovich

Last updated
Leonid Bunimovich
Leonid Bunimovic.jpg
Leonid Bunimovich in 1981
(photo from MFO)
Alma mater Moscow State University (1967)

Leonid Abramowich Bunimovich (born August 1, 1947) is a Soviet and American mathematician, who made fundamental contributions to the theory of Dynamical Systems, Statistical Physics and various applications. Bunimovich received his bachelor's degree in 1967, master's degree in 1969 and PhD in 1973 from the University of Moscow. His masters and PhD thesis advisor was Yakov G. Sinai. In 1986 (after Perestroika started) he finally received Doctor of Sciences degree in "Theoretical and Mathematical Physics". Bunimovich is a Regents' Professor of Mathematics at the Georgia Institute of Technology. Bunimovich is a Fellow of the Institute of Physics and was awarded Humboldt Prize in Physics.

Contents

Biography

His Master's proved that some classes of quadratic maps of an interval have an absolutely continuous invariant measure and strong stochastic properties. [1]

Bunimovich is mostly known for discovery of a fundamental mechanism of chaos in dynamical systems called the mechanism of defocusing. [2] This discovery came as a striking surprise not only to mathematics but to physics community as well. Physicists could not believe that such (physical!) phenomenon is possible (even though a rigorous mathematical proof was provided) until they conducted massive numerical experiments. The most famous class of chaotic dynamical systems of this type, dynamical billiards are focusing chaotic billiards such as the Bunimovich stadium ("Bunimovich flowers", elliptic flowers, etc.). [3] Later Bunimovich proved that his mechanism of defocusing works in all dimensions despite of the phenomenon of astigmatism. [4] Bunimovich introduced absolutely focusing mirrors, which is a new notion in geometric optics, and proved that only such mirrors could be focusing parts of chaotic billiards. [5] He also constructed so called Bunimovich mushrooms, which are visual examples of billiards with mixed regular and chaotic dynamics. [6] [7] Physical realizations of Bunimovich stadia have been constructed for both classical and quantum investigations..

Although the discovery of defocusing mechanism was just a part of Bunimovich's PhD thesis, he could not find any job after finishing graduate school thanks to antisemitic policies in the Soviet Union. Bunimovich was unable to ever find work as a mathematician in the Soviet Union. Moreover, while finally finding a work place he was not allowed to publish mathematical papers because in the places, where he worked, authorities refused to confirm that his mathematical papers contain no state secrets. Likewise for a long time he could not attend mathematical conferences even in the Soviet Union. However, although being trained as a pure mathematician, Bunimovich turned out to be able to work in applications to biomedical studies and in oceanology.

Notably, Bunimovich introduced and investigated hierarchical models of human populations, [8] which allowed to clarify laws of distribution of hereditary diseases and explain data on migration in industrial parts of developed countries. He realized and demonstrated that the lengths of remissions in schizophrenia form a Markov process, i.e. that a length of remission depends only upon the length of the previous remission. Besides he demonstrated that among the types of attacks in schizophrenia there are those which more (or less) likely to occur after the other types of attacks. [9] Before it was just known that the same type of attack will basically always happen.

Bunimovich discovered traps for internal waves in non-homogeneous stratified fluids and analyzed their dynamics in such traps which in particular allowed to explain some surprising observations on internal waves in the oceans. [10]

One of the most fundamental problems in Statistical Physics is to derive Deterministic time-irreversible macro-dynamics from deterministic time-reversible Newtonian micro-dynamics. This problem, which was considered before to be mathematically non-tractable was settled in the paper [11] by Bunimovich with Ya.G. Sinai for diffusion of mass in periodic Lorentz gas. In their previous paper was constructed the first infinite Markov partition for chaotic systems with singularities which allowed to transform this deterministic problem into probabilistic one. Then in Bunimovich's paper with H.Spohn [12] diffusion of shear and bulk viscosities in deterministic periodic fluid was rigorously derived.

The paper by Bunimovich with Ya.G. Sinai [13] pioneered rigorous studies of the space-time chaos. There were even no exact definition of this widely observed in experiments phenomenon. In this paper such definition was given and it was proved that space-time chaos gets realized in weakly interacting time-chaotic systems.

Together with Ben Webb Bunimovich introduced and developed the theory of Isospectral transformations for analysis of multi-dimensional systems and networks. [14] This approach allows to obtain various types of visualization of networks as well to uncover their hierarchical structure and hidden symmetries.

Bunimovich pioneered a rigorous theory of Finite-time dynamics and finite-time predictions for strongly chaotic and random systems. [15] [16]

Together with Skums and Khudyakov Bunimovich discovered phenomenon of local immunodeficiency which demonstrates how viruses can cooperate to overcome an immune response of a human organism. [17] It allowed to clarify numerous unexplained phenomena in evolution of the Hepatitis C and serves as new tool to study any disease with cross-immunoreactivity.

Related Research Articles

Butterfly effect Idea that small causes can have large effects

In chaos theory, the butterfly effect is the sensitive dependence on initial conditions in which a small change in one state of a deterministic nonlinear system can result in large differences in a later state.

Chaos theory Field of mathematics

Chaos theory is an interdisciplinary scientific theory and branch of mathematics focused on underlying patterns and deterministic laws, of dynamical systems, that are highly sensitive to initial conditions, that were once thought to have completely random states of disorder and irregularities. Chaos theory states that within the apparent randomness of chaotic complex systems, there are underlying patterns, interconnection, constant feedback loops, repetition, self-similarity, fractals, and self-organization. The butterfly effect, an underlying principle of chaos, describes how a small change in one state of a deterministic nonlinear system can result in large differences in a later state. A metaphor for this behavior is that a butterfly flapping its wings in Brazil can cause a tornado in Texas.

Dynamical system Mathematical model of the time dependence of a point in space

In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it.

James A. Yorke

James A. Yorke is a Distinguished University Research Professor of Mathematics and Physics and former chair of the Mathematics Department at the University of Maryland, College Park.

Dynamical systems theory Area of mathematics used to describe the behavior of complex dynamical systems

Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. From a physical point of view, continuous dynamical systems is a generalization of classical mechanics, a generalization where the equations of motion are postulated directly and are not constrained to be Euler–Lagrange equations of a least action principle. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a Cantor set, one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations.

Yakov Sinai Russian-American mathematician

Yakov Grigorevich Sinai is a Russian-American mathematician known for his work on dynamical systems. He contributed to the modern metric theory of dynamical systems and connected the world of deterministic (dynamical) systems with the world of probabilistic (stochastic) systems. He has also worked on mathematical physics and probability theory. His efforts have provided the groundwork for advances in the physical sciences.

Dynamical billiards Dynamical system abstract an ideal game of billiards, with elastic collisions off boundaries

A dynamical billiard is a dynamical system in which a particle alternates between free motion and specular reflections from a boundary. When the particle hits the boundary it reflects from it without loss of speed. Billiards are Hamiltonian idealizations of the game of billiards, but where the region contained by the boundary can have shapes other than rectangular and even be multidimensional. Dynamical billiards may also be studied on non-Euclidean geometries; indeed, the first studies of billiards established their ergodic motion on surfaces of constant negative curvature. The study of billiards which are kept out of a region, rather than being kept in a region, is known as outer billiard theory.

Outer billiards is a dynamical system based on a convex shape in the plane. Classically, this system is defined for the Euclidean plane but one can also consider the system in the hyperbolic plane or in other spaces that suitably generalize the plane. Outer billiards differs from a usual dynamical billiard in that it deals with a discrete sequence of moves outside the shape rather than inside of it.

David Ruelle Belgian-French mathematical physicist

David Pierre Ruelle is a Belgian mathematical physicist, naturalized French. He has worked on statistical physics and dynamical systems. With Floris Takens, Ruelle coined the term strange attractor, and developed a new theory of turbulence.

Intermittency Irregular alternation different types of dynamics

In dynamical systems, intermittency is the irregular alternation of phases of apparently periodic and chaotic dynamics, or different forms of chaotic dynamics.

In physics and mathematics, the Hadamard dynamical system is a chaotic dynamical system, a type of dynamical billiards. Introduced by Jacques Hadamard in 1898, and studied by Martin Gutzwiller in the 1980s, it is the first dynamical system to be proven chaotic.

Lorenz system System of ordinary differential equations with chaotic solutions

The Lorenz system is a system of ordinary differential equations first studied by mathematician and meteorologist Edward Lorenz. It is notable for having chaotic solutions for certain parameter values and initial conditions. In particular, the Lorenz attractor is a set of chaotic solutions of the Lorenz system. In popular media the "butterfly effect" stems from the real-world implications of the Lorenz attractor, namely that in a chaotic physical system, in the absence of perfect knowledge of the initial conditions, our ability to predict its future course will always fail. This underscores that physical systems can be completely deterministic and yet still be inherently unpredictable. The shape of the Lorenz attractor itself, when plotted in phase space, may also be seen to resemble a butterfly.

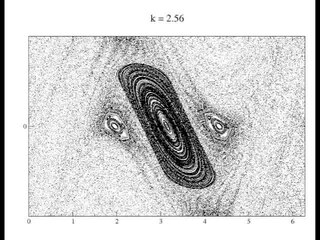
Standard map Area-preserving chaotic map from a square with side 2π onto itself

The standard map is an area-preserving chaotic map from a square with side onto itself. It is constructed by a Poincaré's surface of section of the kicked rotator, and is defined by:

Synchronization of chaos is a phenomenon that may occur when two or more dissipative chaotic systems are coupled.

Boris Chirikov 20th-century Russian physicist

Boris Valerianovich Chirikov was a Soviet and Russian physicist. He was the founder of the physical theory of Hamiltonian chaos and made pioneering contributions to the theory of quantum chaos. In 1959, he invented the Chirikov criterion which gives an analytical estimate for the overlap of resonances and provides the conditions for transition from integrability to global chaos in Hamiltonian dynamical systems.

A coupled map lattice (CML) is a dynamical system that models the behavior of non-linear systems. They are predominantly used to qualitatively study the chaotic dynamics of spatially extended systems. This includes the dynamics of spatiotemporal chaos where the number of effective degrees of freedom diverges as the size of the system increases.

George Zaslavsky Soviet physicist (1935–2008)

George M. Zaslavsky was a Soviet mathematical physicist and one of the founders of the physics of dynamical chaos.

Valentin Afraimovich was a Soviet, Russian and Mexican mathematician. He made contributions to dynamical systems theory, qualitative theory of ordinary differential equations, bifurcation theory, concept of attractor, strange attractors, space-time chaos, mathematical models of nonequilibrium media and biological systems, traveling waves in lattices, complexity of orbits and dimension-like characteristics in dynamical systems.

Yakov Pesin

Yakov Borisovich Pesin was born in Moscow, Russia on December 12, 1946. Pesin is currently a Distinguished Professor in the Department of Mathematics and the Director of the Anatole Katok Center for Dynamical Systems and Geometry at the Pennsylvania State University (PSU). His primary areas of research are the theory of dynamical systems with an emphasis on smooth ergodic theory, dimension theory in dynamical systems, and Riemannian geometry, as well as mathematical and statistical physics.

Supersymmetric theory of stochastic dynamics or stochastics (STS) is an exact theory of stochastic (partial) differential equations (SDEs), the class of mathematical models with the widest applicability covering, in particular, all continuous time dynamical systems, with and without noise. The main utility of the theory from the physical point of view is a rigorous theoretical explanation of the ubiquitous spontaneous long-range dynamical behavior that manifests itself across disciplines via such phenomena as 1/f, flicker, and crackling noises and the power-law statistics, or Zipf's law, of instantonic processes like earthquakes and neuroavalanches. From the mathematical point of view, STS is interesting because it bridges the two major parts of mathematical physics – the dynamical systems theory and topological field theories. Besides these and related disciplines such as algebraic topology and supersymmetric field theories, STS is also connected with the traditional theory of stochastic differential equations and the theory of pseudo-Hermitian operators.

References

  1. ↑ L.Bunimovich, On one transformation of the circle, Mathematical Notes v.5 (1970) 205-216
  2. ↑ L.Bunimovich, On Billiards Close to Dispersing, Mathematical Sbornik, v.94 (1974), 45-67
  3. ↑ L.Bunimovich, On Ergodic Properties of Some Billiards, Func-l Anal-s & Appl-s v.8 (1974) 254-255
  4. ↑ L.Bunimovich, J.Rehacek, How High-Dimensional Stadia Look Like, Comm. Math. Phys.v.197 (1998) 277–301
  5. ↑ L.Bunimovich, Many-dimensional nowhere dispersing chaotic billiards, Physica D v.33 (1988) 58-64
  6. ↑ L.Bunimovich, Mushrooms and Other Billiards with Divided Phase Space, Chaos v.11 (2001) 1-7
  7. ↑ Bunimovich, Leonid (2008). "Chaotic and nonchaotic mushrooms". Discrete and Continuous Dynamical Systems. 22: 63–74. doi: 10.3934/dcds.2008.22.63 .
  8. ↑ L. Bunimovich, Hierarchial Model of Human Populations, Genetika v.11 (1975) 134-143
  9. ↑ L.Shmaonova, Yu. Liberman. L.Bunimovich, Clinical and Statistical laws of the Dynamics of Schizophrenia J. of Neurology and Psychiatry v.48 (1981) 34-42
  10. ↑ L.Bunimovich, On Properties of Internal Waves in Horizontally Varying Vaisala-Brendt Frequency Field in the Ocean, Physics of Atmosphere and Ocean v.16 (1980) 517-525
  11. ↑ L.Bunimovich, Ya.G.Sinai, Statistical properties of the Lorentz Gas with Periodic Configurations of Scatterers, Comm. Math. Phys. v.78 (1981) 479-497
  12. ↑ L.Bunimovich, H.Spohn, Viscosity for Periodic Two-Disk Fluid, Comm. Math. Phys. v.76 (1996) 661-680
  13. ↑ L.Bunimovich, Ya.G.Sinai, Space-Time Chaos in Coupled Map Lattices, Nonlinearity v.1 (1988) 491-516
  14. ↑ L.Bunimovich, B.Webb, Isospectral Transformations: a New Approach to Analysis of Multi-Dimensional Dynamical Systems and Networks, Springer, 2014, XVI+175p
  15. ↑ L.Bunimovich, A.Yurchenko, Where to Place a Hole to Achieve Maximal Escape Rate, Israel. J. Math. v.122 (2011) 229-252
  16. ↑ M.Bolding, L.Bunimovich, Where and When Orbits of Strongly Chaotic Systems Prefer to Go, Nonlinearity v.32 (2019) 1731-1771
  17. ↑ P.Skums, L.Bunimovich, Yu.Khudyakov, Antigenic cooperation among intrahost HCV variants organized into a complex network of cross-immunoreactivity, Proc. Nat-l Ac. Sci. v. 112 (21) (2015) 6653-6658
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.