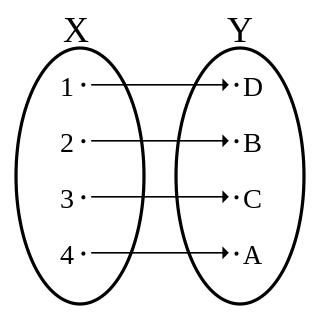
In mathematics, a bijection, bijective function, one-to-one correspondence, or invertible function, is a function between the elements of two sets, where each element of one set is paired with exactly one element of the other set, and each element of the other set is paired with exactly one element of the first set. There are no unpaired elements. In mathematical terms, a bijective function f: X → Y is a one-to-one (injective) and onto (surjective) mapping of a set X to a set Y. The term one-to-one correspondence must not be confused with one-to-one function.

In abstract algebra, a branch of mathematics, a monoid is a set equipped with an associative binary operation and an identity element.
In mathematics, a partial functionf from a set X to a set Y is a function from a subset S of X to Y. The subset S, that is, the domain of f viewed as a function, is called the domain of definition of f. If S equals X, that is, if f is defined on every element in X, then f is said to be total.

In mathematics, a semigroup is an algebraic structure consisting of a set together with an associative binary operation.
In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. In particular, the finite symmetric group defined over a finite set of symbols consists of the permutations that can be performed on the symbols. Since there are such permutation operations, the order of the symmetric group is .
In abstract algebra, the idea of an inverse element generalises the concepts of negation and reciprocation. The intuition is of an element that can 'undo' the effect of combination with another given element. While the precise definition of an inverse element varies depending on the algebraic structure involved, these definitions coincide in a group.
In abstract algebra, a generating set of a group is a subset of the group set such that every element of the group can be expressed as a combination of finitely many elements of the subset and their inverses.

In group theory, the wreath product is a special combination of two groups based on the semidirect product. It is formed by the action of one group on many copies of another group, somewhat analogous to exponentiation. Wreath products are used in the classification of permutation groups and also provide a way of constructing interesting examples of groups.
In mathematics, function composition is an operation that takes two functions f and g and produces a function h such that h(x) = g(f ). In this operation, the function g is applied to the result of applying the function f to x. That is, the functions f : X → Y and g : Y → Z are composed to yield a function that maps x in X to g(f ) in Z.

In mathematics, the symmetric difference of two sets, also known as the disjunctive union, is the set of elements which are in either of the sets, but not in their intersection. For example, the symmetric difference of the sets and is .
In mathematics, a join-semilattice is a partially ordered set that has a join for any nonempty finite subset. Dually, a meet-semilattice is a partially ordered set which has a meet for any nonempty finite subset. Every join-semilattice is a meet-semilattice in the inverse order and vice versa.
In mathematics, Green's relations are five equivalence relations that characterise the elements of a semigroup in terms of the principal ideals they generate. The relations are named for James Alexander Green, who introduced them in a paper of 1951. John Mackintosh Howie, a prominent semigroup theorist, described this work as "so all-pervading that, on encountering a new semigroup, almost the first question one asks is 'What are the Green relations like?'". The relations are useful for understanding the nature of divisibility in a semigroup; they are also valid for groups, but in this case tell us nothing useful, because groups always have divisibility.
In group theory, an inverse semigroupS is a semigroup in which every element x in S has a unique inversey in S in the sense that x = xyx and y = yxy, i.e. a regular semigroup in which every element has a unique inverse. Inverse semigroups appear in a range of contexts; for example, they can be employed in the study of partial symmetries.

In mathematics, a transformation is a function f that maps a set X to itself, i.e. f : X → X. In other areas of mathematics, a transformation may simply refer to any function, regardless of domain and codomain. For this wider sense of the term, see function (mathematics).
In abstract algebra, a semiheap is an algebraic structure consisting of a non-empty set H with a ternary operation denoted that satisfies a modified associativity property:
In mathematics, a regular semigroup is a semigroup S in which every element is regular, i.e., for each element a in S there exists an element x in S such that axa = a. Regular semigroups are one of the most-studied classes of semigroups, and their structure is particularly amenable to study via Green's relations.
Viktor Vladimirovich Wagner, also Vagner was a Russian mathematician, best known for his work in differential geometry and on semigroups.
In algebra, a transformation semigroup is a collection of transformations that is closed under function composition. If it includes the identity function, it is a monoid, called a transformationmonoid. This is the semigroup analogue of a permutation group.

In mathematics, a matrix is a rectangular array or table of numbers, symbols, or expressions, arranged in rows and columns, which is used to represent a mathematical object or a property of such an object.
In mathematics, particularly in abstract algebra, a semigroup with involution or a *-semigroup is a semigroup equipped with an involutive anti-automorphism, which—roughly speaking—brings it closer to a group because this involution, considered as unary operator, exhibits certain fundamental properties of the operation of taking the inverse in a group: uniqueness, double application "cancelling itself out", and the same interaction law with the binary operation as in the case of the group inverse. It is thus not a surprise that any group is a semigroup with involution. However, there are significant natural examples of semigroups with involution that are not groups.










