Tree rotation

Last updated
Generic tree rotations. BinaryTreeRotations.svg
Generic tree rotations.

In discrete mathematics, tree rotation is an operation on a binary tree that changes the structure without interfering with the order of the elements. A tree rotation moves one node up in the tree and one node down. It is used to change the shape of the tree, and in particular to decrease its height by moving smaller subtrees down and larger subtrees up, resulting in improved performance of many tree operations.

Contents

There exists an inconsistency in different descriptions as to the definition of the direction of rotations. Some say that the direction of rotation reflects the direction that a node is moving upon rotation (a left child rotating into its parent's location is a right rotation) while others say that the direction of rotation reflects which subtree is rotating (a left subtree rotating into its parent's location is a left rotation, the opposite of the former). This article takes the approach of the directional movement of the rotating node.

Illustration

Tree rotation.png
Animation of tree rotations taking place. Tree rotation animation 250x250.gif
Animation of tree rotations taking place.

The right rotation operation as shown in the adjacent image is performed with Q as the root and hence is a right rotation on, or rooted at, Q. This operation results in a rotation of the tree in the clockwise direction. The inverse operation is the left rotation, which results in a movement in a counter-clockwise direction (the left rotation shown above is rooted at P). The key to understanding how a rotation functions is to understand its constraints. In particular the order of the leaves of the tree (when read left to right for example) cannot change (another way to think of it is that the order that the leaves would be visited in an in-order traversal must be the same after the operation as before). Another constraint is the main property of a binary search tree, namely that all nodes in the right subtree are greater than the parent and all nodes in the left subtree are less than the parent. Notice that the right child of a left child of the root of a sub-tree (for example node B in the diagram for the tree rooted at Q) can become the left child of the root, that itself becomes the right child of the "new" root in the rotated sub-tree, without violating either of those constraints. As seen in the diagram, the order of the leaves doesn't change. The opposite operation also preserves the order and is the second kind of rotation.

Assuming this is a binary search tree, as stated above, the elements must be interpreted as variables that can be compared to each other. The alphabetic characters to the left are used as placeholders for these variables. In the animation to the right, capital alphabetic characters are used as variable placeholders while lowercase Greek letters are placeholders for an entire set of variables. The circles represent individual nodes and the triangles represent subtrees. Each subtree could be empty, consist of a single node, or consist of any number of nodes.

Detailed illustration

Pictorial description of how rotations are made. Tree Rotations.gif
Pictorial description of how rotations are made.

When a subtree is rotated, the subtree side upon which it is rotated increases its height by one node while the other subtree decreases its height. This makes tree rotations useful for rebalancing a tree.

Consider the terminology of Root for the parent node of the subtrees to rotate, Pivot for the node which will become the new parent node, RS for the side of rotation and OS for the opposite side of rotation. For the root Q in the diagram above, RS is C and OS is P. Using these terms, the pseudo code for the rotation is:

Pivot = Root.OS Root.OS = Pivot.RS Pivot.RS = Root Root = Pivot

This is a constant time operation.

The programmer must also make sure that the root's parent points to the pivot after the rotation. Also, the programmer should note that this operation may result in a new root for the entire tree and take care to update pointers accordingly.

Inorder invariance

The tree rotation renders the inorder traversal of the binary tree invariant. This implies the order of the elements is not affected when a rotation is performed in any part of the tree. Here are the inorder traversals of the trees shown above:

Left tree: ((A, P, B), Q, C)        Right tree: (A, P, (B, Q, C))

Computing one from the other is very simple. The following is example Python code that performs that computation:

defright_rotation(treenode):"""Rotate the specified tree to the right."""left,Q,C=treenodeA,P,B=leftreturn(A,P,(B,Q,C))

Another way of looking at it is:

Right rotation of node Q:

Let P be Q's left child. Set Q's left child to be P's right child. [Set P's right-child's parent to Q] Set P's right child to be Q. [Set Q's parent to P]

Left rotation of node P:

Let Q be P's right child. Set P's right child to be Q's left child. [Set Q's left-child's parent to P] Set Q's left child to be P. [Set P's parent to Q]

All other connections are left as-is.

There are also double rotations, which are combinations of left and right rotations. A double left rotation at X can be defined to be a right rotation at the right child of X followed by a left rotation at X; similarly, a double right rotation at X can be defined to be a left rotation at the left child of X followed by a right rotation at X.

Tree rotations are used in a number of tree data structures such as AVL trees, red–black trees, WAVL trees, splay trees, and treaps. They require only constant time because they are local transformations: they only operate on 5 nodes, and need not examine the rest of the tree.

Rotations for rebalancing

Pictorial description of how rotations cause rebalancing in an AVL tree. Tree Rebalancing.gif
Pictorial description of how rotations cause rebalancing in an AVL tree.

A tree can be rebalanced using rotations. After a rotation, the side of the rotation increases its height by 1 whilst the side opposite the rotation decreases its height similarly. Therefore, one can strategically apply rotations to nodes whose left child and right child differ in height by more than 1. Self-balancing binary search trees apply this operation automatically. A type of tree which uses this rebalancing technique is the AVL tree.

Rotation distance

Unsolved problem in computer science:

Can the rotation distance between two binary trees be computed in polynomial time?

(more unsolved problems in computer science)

The rotation distance between any two binary trees with the same number of nodes is the minimum number of rotations needed to transform one into the other. With this distance, the set of n-node binary trees becomes a metric space: the distance is symmetric, positive when given two different trees, and satisfies the triangle inequality.

It is an open problem whether there exists a polynomial time algorithm for calculating rotation distance, though several variants of the rotation distance problem admit polynomial time algorithms. [1] [2] [3]

Daniel Sleator, Robert Tarjan and William Thurston showed that the rotation distance between any two n-node trees (for n ≥ 11) is at most 2n − 6, and that some pairs of trees are this far apart as soon as n is sufficiently large. [4] Lionel Pournin showed that, in fact, such pairs exist whenever n ≥ 11. [5]

See also

Related Research Articles

<span class="mw-page-title-main">AVL tree</span> Self-balancing binary search tree

In computer science, an AVL tree is a self-balancing binary search tree. In an AVL tree, the heights of the two child subtrees of any node differ by at most one; if at any time they differ by more than one, rebalancing is done to restore this property. Lookup, insertion, and deletion all take O(log n) time in both the average and worst cases, where is the number of nodes in the tree prior to the operation. Insertions and deletions may require the tree to be rebalanced by one or more tree rotations.

<span class="mw-page-title-main">Binary search tree</span> Rooted binary tree data structure

In computer science, a binary search tree (BST), also called an ordered or sorted binary tree, is a rooted binary tree data structure with the key of each internal node being greater than all the keys in the respective node's left subtree and less than the ones in its right subtree. The time complexity of operations on the binary search tree is linear with respect to the height of the tree.

<span class="mw-page-title-main">Binary tree</span> Limited form of tree data structure

In computer science, a binary tree is a tree data structure in which each node has at most two children, referred to as the left child and the right child. That is, it is a k-ary tree with k = 2. A recursive definition using set theory is that a binary tree is a tuple (L, S, R), where L and R are binary trees or the empty set and S is a singleton set containing the root.

In computer science, a B-tree is a self-balancing tree data structure that maintains sorted data and allows searches, sequential access, insertions, and deletions in logarithmic time. The B-tree generalizes the binary search tree, allowing for nodes with more than two children. Unlike other self-balancing binary search trees, the B-tree is well suited for storage systems that read and write relatively large blocks of data, such as databases and file systems.

In computer science, a red–black tree is a specialised binary search tree data structure noted for fast storage and retrieval of ordered information, and a guarantee that operations will complete within a known time. Compared to other self-balancing binary search trees, the nodes in a red-black tree hold an extra bit called "color" representing "red" and "black" which is used when re-organising the tree to ensure that it is always approximately balanced.

A splay tree is a binary search tree with the additional property that recently accessed elements are quick to access again. Like self-balancing binary search trees, a splay tree performs basic operations such as insertion, look-up and removal in O(log n) amortized time. For random access patterns drawn from a non-uniform random distribution, their amortized time can be faster than logarithmic, proportional to the entropy of the access pattern. For many patterns of non-random operations, also, splay trees can take better than logarithmic time, without requiring advance knowledge of the pattern. According to the unproven dynamic optimality conjecture, their performance on all access patterns is within a constant factor of the best possible performance that could be achieved by any other self-adjusting binary search tree, even one selected to fit that pattern. The splay tree was invented by Daniel Sleator and Robert Tarjan in 1985.

<span class="mw-page-title-main">Tree (data structure)</span> Linked node hierarchical data structure

In computer science, a tree is a widely used abstract data type that represents a hierarchical tree structure with a set of connected nodes. Each node in the tree can be connected to many children, but must be connected to exactly one parent, except for the root node, which has no parent. These constraints mean there are no cycles or "loops", and also that each child can be treated like the root node of its own subtree, making recursion a useful technique for tree traversal. In contrast to linear data structures, many trees cannot be represented by relationships between neighboring nodes in a single straight line.

<span class="mw-page-title-main">Treap</span>

In computer science, the treap and the randomized binary search tree are two closely related forms of binary search tree data structures that maintain a dynamic set of ordered keys and allow binary searches among the keys. After any sequence of insertions and deletions of keys, the shape of the tree is a random variable with the same probability distribution as a random binary tree; in particular, with high probability its height is proportional to the logarithm of the number of keys, so that each search, insertion, or deletion operation takes logarithmic time to perform.

In computer science, tree traversal is a form of graph traversal and refers to the process of visiting each node in a tree data structure, exactly once. Such traversals are classified by the order in which the nodes are visited. The following algorithms are described for a binary tree, but they may be generalized to other trees as well.

In computer science, a 2–3 tree is a tree data structure, where every node with children has either two children (2-node) and one data element or three children (3-node) and two data elements. A 2–3 tree is a B-tree of order 3. Nodes on the outside of the tree have no children and one or two data elements. 2–3 trees were invented by John Hopcroft in 1970.

<span class="mw-page-title-main">T-tree</span>

In computer science a T-tree is a type of binary tree data structure that is used by main-memory databases, such as Datablitz, eXtremeDB, MySQL Cluster, Oracle TimesTen and MobileLite.

In computer science, a scapegoat tree is a self-balancing binary search tree, invented by Arne Andersson in 1989 and again by Igal Galperin and Ronald L. Rivest in 1993. It provides worst-case lookup time and amortized insertion and deletion time.

In computer science, an interval tree is a tree data structure to hold intervals. Specifically, it allows one to efficiently find all intervals that overlap with any given interval or point. It is often used for windowing queries, for instance, to find all roads on a computerized map inside a rectangular viewport, or to find all visible elements inside a three-dimensional scene. A similar data structure is the segment tree.

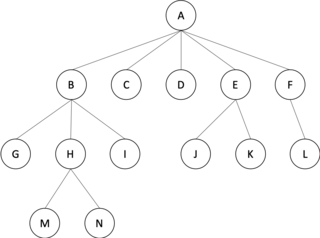
<i>m</i>-ary tree Tree data structure in which each node has at most m children.

In graph theory, an m-ary tree is an arborescence in which each node has no more than m children. A binary tree is the special case where m = 2, and a ternary tree is another case with m = 3 that limits its children to three.

Left rotation refers to the following

In computer science, weight-balanced binary trees (WBTs) are a type of self-balancing binary search trees that can be used to implement dynamic sets, dictionaries (maps) and sequences. These trees were introduced by Nievergelt and Reingold in the 1970s as trees of bounded balance, or BB[α] trees. Their more common name is due to Knuth.

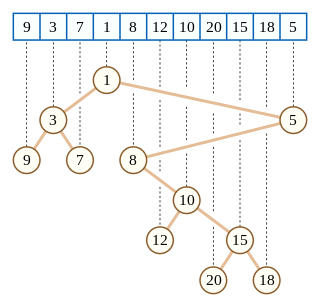
<span class="mw-page-title-main">Cartesian tree</span> Binary tree derived from a sequence of numbers

In computer science, a Cartesian tree is a binary tree derived from a sequence of distinct numbers. To construct the Cartesian tree, set its root to be the minimum number in the sequence, and recursively construct its left and right subtrees from the subsequences before and after this number. It is uniquely defined as a min-heap whose symmetric (in-order) traversal returns the original sequence.

In computer science, a WAVL tree or weak AVL tree is a self-balancing binary search tree. WAVL trees are named after AVL trees, another type of balanced search tree, and are closely related both to AVL trees and red–black trees, which all fall into a common framework of rank balanced trees. Like other balanced binary search trees, WAVL trees can handle insertion, deletion, and search operations in time O(log n) per operation.

In computer science, join-based tree algorithms are a class of algorithms for self-balancing binary search trees. This framework aims at designing highly-parallelized algorithms for various balanced binary search trees. The algorithmic framework is based on a single operation join. Under this framework, the join operation captures all balancing criteria of different balancing schemes, and all other functions join have generic implementation across different balancing schemes. The join-based algorithms can be applied to at least four balancing schemes: AVL trees, red–black trees, weight-balanced trees and treaps.

In discrete mathematics and theoretical computer science, the rotation distance between two binary trees with the same number of nodes is the minimum number of tree rotations needed to reconfigure one tree into another. Because of a combinatorial equivalence between binary trees and triangulations of convex polygons, rotation distance is equivalent to the flip distance for triangulations of convex polygons.

References

  1. ↑ Fordham, Blake (2003), "Minimal Length Elements of Thompson's Group F", Geometriae Dedicata, Springer Science and Business Media LLC, 99 (1): 179–220, doi:10.1023/a:1024971818319, ISSN   0046-5755
  2. ↑ Bonnin, André; Pallo, Jean-Marcel (1992), "A shortest path metric on unlabeled binary trees", Pattern Recognition Letters, Elsevier BV, 13 (6): 411–415, doi:10.1016/0167-8655(92)90047-4, ISSN   0167-8655
  3. ↑ Pallo, Jean Marcel (2003), "Right-arm rotation distance between binary trees", Information Processing Letters, Elsevier BV, 87 (4): 173–177, doi:10.1016/s0020-0190(03)00283-7, ISSN   0020-0190
  4. ↑ Sleator, Daniel D.; Tarjan, Robert E.; Thurston, William P. (1988), "Rotation distance, triangulations, and hyperbolic geometry", Journal of the American Mathematical Society , 1 (3): 647–681, doi: 10.2307/1990951 , JSTOR   1990951, MR   0928904 .
  5. ↑ Pournin, Lionel (2014), "The diameter of associahedra", Advances in Mathematics , 259: 13–42, arXiv: 1207.6296 , doi: 10.1016/j.aim.2014.02.035 , MR   3197650 .
This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.