
An abacus, also called a counting frame, is a hand-operated calculating tool which was used from ancient times in the ancient Near East, Europe, China, and Russia, until the adoption of the Hindu–Arabic numeral system. An abacus consists of a two-dimensional array of slidable beads. In their earliest designs, the beads could be loose on a flat surface or sliding in grooves. Later the beads were made to slide on rods and built into a frame, allowing faster manipulation.

Arithmetic is an elementary branch of mathematics that studies numerical operations like addition, subtraction, multiplication, and division. In a wider sense, it also includes exponentiation, extraction of roots, and taking logarithms.

In mathematics, a multiplication table is a mathematical table used to define a multiplication operation for an algebraic system.

Addition is one of the four basic operations of arithmetic, the other three being subtraction, multiplication and division. The addition of two whole numbers results in the total amount or sum of those values combined. The example in the adjacent image shows two columns of three apples and two apples each, totaling at five apples. This observation is equivalent to the mathematical expression "3 + 2 = 5".

Subtraction is one of the four arithmetic operations along with addition, multiplication and division. Subtraction is an operation that represents removal of objects from a collection. For example, in the adjacent picture, there are 5 − 2 peaches—meaning 5 peaches with 2 taken away, resulting in a total of 3 peaches. Therefore, the difference of 5 and 2 is 3; that is, 5 − 2 = 3. While primarily associated with natural numbers in arithmetic, subtraction can also represent removing or decreasing physical and abstract quantities using different kinds of objects including negative numbers, fractions, irrational numbers, vectors, decimals, functions, and matrices.

New Mathematics or New Math was a dramatic but temporary change in the way mathematics was taught in American grade schools, and to a lesser extent in European countries and elsewhere, during the 1950s–1970s.
In computer science, arbitrary-precision arithmetic, also called bignum arithmetic, multiple-precision arithmetic, or sometimes infinite-precision arithmetic, indicates that calculations are performed on numbers whose digits of precision are potentially limited only by the available memory of the host system. This contrasts with the faster fixed-precision arithmetic found in most arithmetic logic unit (ALU) hardware, which typically offers between 8 and 64 bits of precision.

Cuisenaire rods are mathematics learning aids for pupils that provide an interactive, hands-on way to explore mathematics and learn mathematical concepts, such as the four basic arithmetical operations, working with fractions and finding divisors. In the early 1950s, Caleb Gattegno popularised this set of coloured number rods created by Georges Cuisenaire (1891–1975), a Belgian primary school teacher, who called the rods réglettes.
Principles and Standards for School Mathematics (PSSM) are guidelines produced by the National Council of Teachers of Mathematics (NCTM) in 2000, setting forth recommendations for mathematics educators. They form a national vision for preschool through twelfth grade mathematics education in the US and Canada. It is the primary model for standards-based mathematics.

Elementary arithmetic is a branch of mathematics involving addition, subtraction, multiplication, and division. Due to its low level of abstraction, broad range of application, and position as the foundation of all mathematics, elementary arithmetic is generally the first branch of mathematics taught in schools.
In elementary arithmetic, a standard algorithm or method is a specific method of computation which is conventionally taught for solving particular mathematical problems. These methods vary somewhat by nation and time, but generally include exchanging, regrouping, long division, and long multiplication using a standard notation, and standard formulas for average, area, and volume. Similar methods also exist for procedures such as square root and even more sophisticated functions, but have fallen out of the general mathematics curriculum in favor of calculators. As to standard algorithms in elementary mathematics, Fischer et al. (2019) state that advanced students use standard algorithms more effectively than peers who use these algorithms unreasoningly. That said, standard algorithms, such as addition, subtraction, as well as those mentioned above, represent central components of elementary math.
In psychology, number sense is the term used for the hypothesis that some animals, particularly humans, have a biologically determined ability that allows them to represent and manipulate large numerical quantities. The term was popularized by Stanislas Dehaene in his 1997 book "The Number Sense," but originally named by the mathematician Tobias Dantzig in his 1930 text Number: The Language of Science.
Arithmetic is an elementary branch of mathematics that is widely used for tasks ranging from simple day-to-day counting to advanced science and business calculations.

In mathematics education, a manipulative is an object which is designed so that a learner can perceive some mathematical concept by manipulating it, hence its name. The use of manipulatives provides a way for children to learn concepts through developmentally appropriate hands-on experience.
Traditional mathematics was the predominant method of mathematics education in the United States in the early-to-mid 20th century. This contrasts with non-traditional approaches to math education. Traditional mathematics education has been challenged by several reform movements over the last several decades, notably new math, a now largely abandoned and discredited set of alternative methods, and most recently reform or standards-based mathematics based on NCTM standards, which is federally supported and has been widely adopted, but subject to ongoing criticism.
Investigations in Numbers, Data, and Space is a K–5 mathematics curriculum, developed at TERC in Cambridge, Massachusetts, United States. The curriculum is often referred to as Investigations or simply TERC. Patterned after the NCTM standards for mathematics, it is among the most widely used of the new reform mathematics curricula. As opposed to referring to textbooks and having teachers impose methods for solving arithmetic problems, the TERC program uses a constructivist approach that encourages students to develop their own understanding of mathematics. The curriculum underwent a major revision in 2005–2007.
Singapore math is a teaching method based on the national mathematics curriculum used for first through sixth grade in Singaporean schools. The term was coined in the United States to describe an approach originally developed in Singapore to teach students to learn and master fewer mathematical concepts at greater detail as well as having them learn these concepts using a three-step learning process: concrete, pictorial, and abstract. In the concrete step, students engage in hands-on learning experiences using physical objects which can be everyday items such as paper clips, toy blocks or math manipulates such as counting bears, link cubes and fraction discs. This is followed by drawing pictorial representations of mathematical concepts. Students then solve mathematical problems in an abstract way by using numbers and symbols.

Virtual manipulatives for mathematics are digital representations of physical mathematics manipulatives used in classrooms. The goal of this technology is to allow learners to investigate, explore, and derive mathematical concepts using concrete models.
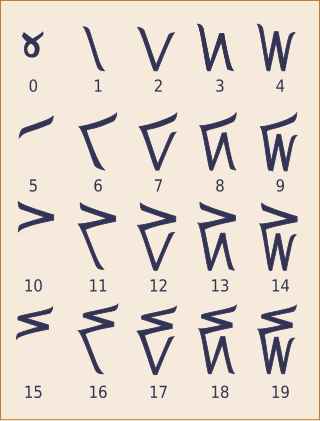
The Kaktovik numerals or Kaktovik Iñupiaq numerals are a base-20 system of numerical digits created by Alaskan Iñupiat. They are visually iconic, with shapes that indicate the number being represented.
Playing with Infinity: Mathematical Explorations and Excursions is a book in popular mathematics by Hungarian mathematician Rózsa Péter, published in German in 1955 and in English in 1961.













