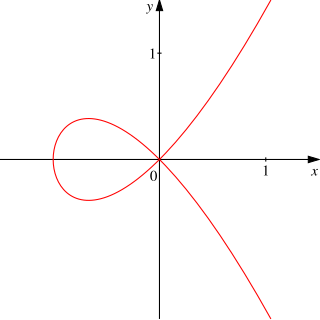In geometry, the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant concyclic points defined from the triangle. These nine points are:

In geometry, symmedians are three particular geometrical lines associated with every triangle. They are constructed by taking a median of the triangle, and reflecting the line over the corresponding angle bisector. The angle formed by the symmedian and the angle bisector has the same measure as the angle between the median and the angle bisector, but it is on the other side of the angle bisector.
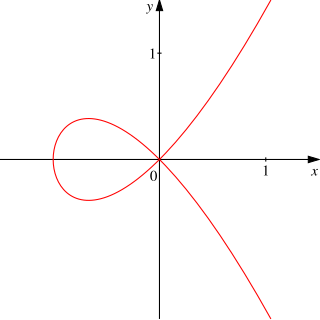
In mathematics, a cubic plane curve is a plane algebraic curve C defined by a cubic equation

In geometry, the isogonal conjugate of a point P with respect to a triangle ABC is constructed by reflecting the lines PA, PB, and PC about the angle bisectors of A, B, and C respectively. These three reflected lines concur at the isogonal conjugate of P. This is a direct result of the trigonometric form of Ceva's theorem.

In geometry, three or more lines in a plane or higher-dimensional space are said to be concurrent if they intersect at a single point.
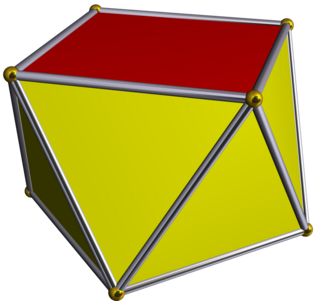
In geometry, the square antiprism is the second in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. It is also known as an anticube.
In geometry, collinearity of a set of points is the property of their lying on a single line. A set of points with this property is said to be collinear. In greater generality, the term has been used for aligned objects, that is, things being "in a line" or "in a row".

In geometry, a centre of an object is a point in some sense in the middle of the object. According to the specific definition of centre taken into consideration, an object might have no centre. If geometry is regarded as the study of isometry groups then a centre is a fixed point of all the isometries which move the object onto itself.

In Euclidean geometry, the isodynamic points of a triangle are points associated with the triangle, with the properties that an inversion centered at one of these points transforms the given triangle into an equilateral triangle, and that the distances from the isodynamic point to the triangle vertices are inversely proportional to the opposite side lengths of the triangle. Triangles that are similar to each other have isodynamic points in corresponding locations in the plane, so the isodynamic points are triangle centers, and unlike other triangle centers the isodynamic points are also invariant under Möbius transformations. A triangle that is itself equilateral has a unique isodynamic point, at its centroid; every non-equilateral triangle has two isodynamic points. Isodynamic points were first studied and named by Joseph Neuberg (1885).
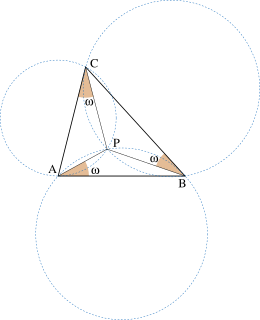
In geometry, Brocard points are special points within a triangle. They are named after Henri Brocard, a French mathematician.

In geometry, the Desargues configuration is a configuration of ten points and ten lines, with three points per line and three lines per point. It is named after Girard Desargues, and closely related to Desargues' theorem, which proves the existence of the configuration.
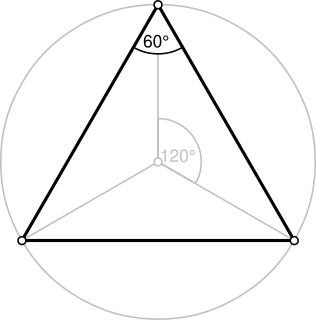
In geometry, a triangle center is a point in the plane that is in some sense a center of a triangle akin to the centers of squares and circles, i.e. a point that is in the middle of the figure by some measure. For example the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions.

In geometry, a transversal is a line that passes through two lines in the same plane at two distinct points. Transversals play a role in establishing whether two other lines in the Euclidean plane are parallel. The intersections of a transversal with two lines create various types of pairs of angles: consecutive interior angles, corresponding angles, and alternate angles. By Euclid's parallel postulate, if the two lines are parallel, consecutive interior angles are supplementary, corresponding angles are equal, and alternate angles are equal.
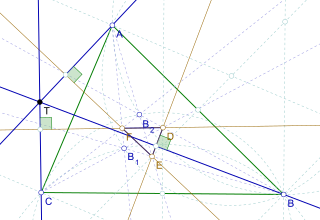
In geometry, the Tarry point T for a triangle ABC is a point of concurrency of the lines through the vertices of the triangle perpendicular to the corresponding sides of the triangle's first Brocard triangle DEF. The Tarry point lies on the other endpoint of the diameter of the circumcircle drawn through the Steiner point. The point is named for Gaston Tarry.

In geometry, the Brocard circle for a triangle is a circle defined from a given triangle. It passes through the circumcenter and symmedian of the triangle, and is centered at the midpoint of the line segment joining them.

Pierre René Jean Baptiste Henri Brocard was a French meteorologist and mathematician, in particular a geometer. His best-known achievement is the invention and discovery of the properties of the Brocard points, the Brocard circle, and the Brocard triangle, all bearing his name.
In geometry, polygons are associated into pairs called duals, where the vertices of one correspond to the edges of the other.
In triangle geometry, the Steiner point is a particular point associated with a plane triangle. It is a triangle center and it is designated as the center X(99) in Clark Kimberling's Encyclopedia of Triangle Centers. Jakob Steiner (1796–1863), Swiss mathematician, described this point in 1826. The point was given Steiner's name by Joseph Neuberg in 1886.

In plane geometry, an extended side or sideline of a polygon is the line that contains one side of the polygon. The extension of a side arises in various contexts.

In geometry, the orthocentroidal circle of a non-equilateral triangle is the circle that has the triangle's orthocenter and its centroid at opposite ends of a diameter. This diameter also contains the triangle's nine-point center and is a subset of the Euler line, which also contains the circumcenter outside the orthocentroidal circle.