In geometry, an icosidodecahedron or pentagonal gyrobirotunda is a polyhedron with twenty (icosi-) triangular faces and twelve (dodeca-) pentagonal faces. An icosidodecahedron has 30 identical vertices, with two triangles and two pentagons meeting at each, and 60 identical edges, each separating a triangle from a pentagon. As such, it is one of the Archimedean solids and more particularly, a quasiregular polyhedron.
In geometry, a polyhedral compound is a figure that is composed of several polyhedra sharing a common centre. They are the three-dimensional analogs of polygonal compounds such as the hexagram.

In geometry, the rhombicosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.
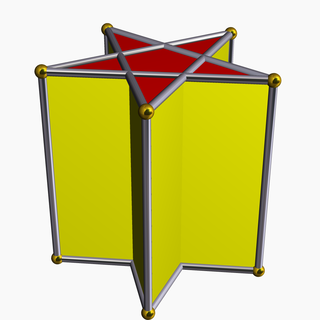
In geometry, the pentagrammic prism is one of an infinite set of nonconvex prisms formed by square sides and two regular star polygon caps, in this case two pentagrams.

In geometry, the truncated great dodecahedron is a nonconvex uniform polyhedron, indexed as U37. It has 24 faces (12 pentagrams and 12 decagons), 90 edges, and 60 vertices. It is given a Schläfli symbol t{5,5/2}.
In geometry, the small stellated truncated dodecahedron (or quasitruncated small stellated dodecahedron or small stellatruncated dodecahedron) is a nonconvex uniform polyhedron, indexed as U58. It has 24 faces (12 pentagons and 12 decagrams), 90 edges, and 60 vertices. It is given a Schläfli symbol t{5⁄3,5}, and Coxeter diagram .
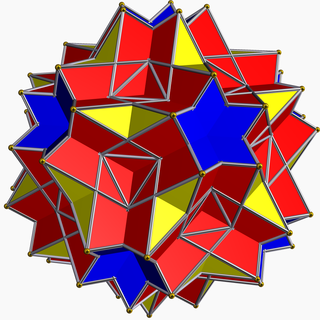
In geometry, the great dodecicosidodecahedron (or great dodekicosidodecahedron) is a nonconvex uniform polyhedron, indexed as U61. It has 44 faces (20 triangles, 12 pentagrams and 12 decagrams), 120 edges and 60 vertices.

In geometry, the rhombidodecadodecahedron is a nonconvex uniform polyhedron, indexed as U38. It has 54 faces (30 squares, 12 pentagons and 12 pentagrams), 120 edges and 60 vertices. It is given a Schläfli symbol t0,2{5⁄2,5}, and by the Wythoff construction this polyhedron can also be named a cantellated great dodecahedron.

In geometry, the icosidodecadodecahedron (or icosified dodecadodecahedron) is a nonconvex uniform polyhedron, indexed as U44. It has 44 faces (12 pentagons, 12 pentagrams and 20 hexagons), 120 edges and 60 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the nonconvex great rhombicosidodecahedron is a nonconvex uniform polyhedron, indexed as U67. It has 62 faces (20 triangles, 30 squares and 12 pentagrams), 120 edges, and 60 vertices. It is also called the quasirhombicosidodecahedron. It is given a Schläfli symbol rr{5⁄3,3}. Its vertex figure is a crossed quadrilateral.

In geometry, the great rhombidodecahedron is a nonconvex uniform polyhedron, indexed as U73. It has 42 faces (30 squares, 12 decagrams), 120 edges and 60 vertices. Its vertex figure is a crossed quadrilateral.

The compound of five cubes is one of the five regular polyhedral compounds. It was first described by Edmund Hess in 1876.
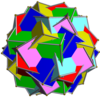
In geometry, a uniform polyhedron compound is a polyhedral compound whose constituents are identical uniform polyhedra, in an arrangement that is also uniform, i.e. the symmetry group of the compound acts transitively on the compound's vertices.

In geometry, a prismatic uniform polyhedron is a uniform polyhedron with dihedral symmetry. They exist in two infinite families, the uniform prisms and the uniform antiprisms. All have their vertices in parallel planes and are therefore prismatoids.

The compound of twenty octahedra is a uniform polyhedron compound. It's composed of a symmetric arrangement of 20 octahedra. It is a special case of the compound of 20 octahedra with rotational freedom, in which pairs of octahedral vertices coincide.

This uniform polyhedron compound is a symmetric arrangement of 20 triangular prisms, aligned in pairs with the axes of three-fold rotational symmetry of an icosahedron.

This uniform polyhedron compound is a symmetric arrangement of 8 triangular prisms, aligned in pairs with the axes of three-fold rotational symmetry of an octahedron. It results from composing the two enantiomorphs of the compound of 4 triangular prisms.

This uniform polyhedron compound is a chiral symmetric arrangement of six pentagonal prisms, aligned with the axes of fivefold rotational symmetry of a dodecahedron.

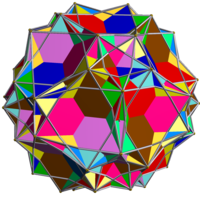
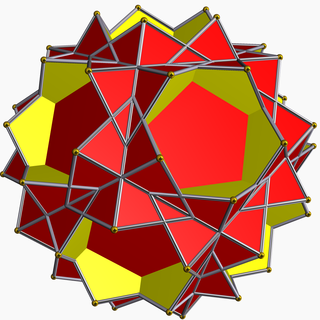
This uniform polyhedron compound is a symmetric arrangement of 12 pentagrammic prisms, aligned in pairs with the axes of fivefold rotational symmetry of a dodecahedron.