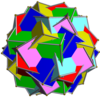
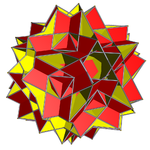
In geometry, the rhombicosidodecahedron is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.

In geometry, the cubohemioctahedron is a nonconvex uniform polyhedron, indexed as U15. It has 10 faces (6 squares and 4 regular hexagons), 24 edges and 12 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the small rhombihexahedron (or small rhombicube) is a nonconvex uniform polyhedron, indexed as U18. It has 18 faces (12 squares and 6 octagons), 48 edges, and 24 vertices. Its vertex figure is an antiparallelogram.

In geometry, the nonconvex great rhombicuboctahedron is a nonconvex uniform polyhedron, indexed as U17. It has 26 faces (8 triangles and 18 squares), 48 edges, and 24 vertices. It is represented by the Schläfli symbol rr{4,3⁄2} and Coxeter-Dynkin diagram of . Its vertex figure is a crossed quadrilateral.
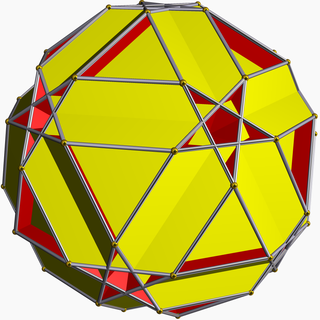
In geometry, the small dodecicosahedron (or small dodekicosahedron) is a nonconvex uniform polyhedron, indexed as U50. It has 32 faces (20 hexagons and 12 decagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the small dodecicosidodecahedron (or small dodekicosidodecahedron) is a nonconvex uniform polyhedron, indexed as U33. It has 44 faces (20 triangles, 12 pentagons, and 12 decagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.
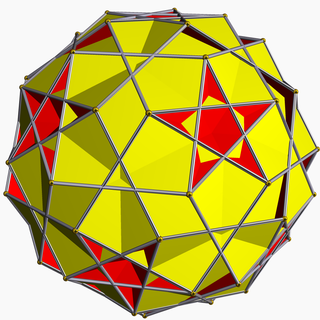
In geometry, the rhombicosahedron is a nonconvex uniform polyhedron, indexed as U56. It has 50 faces (30 squares and 20 hexagons), 120 edges and 60 vertices. Its vertex figure is an antiparallelogram.

In geometry, the great icosicosidodecahedron (or great icosified icosidodecahedron) is a nonconvex uniform polyhedron, indexed as U48. It has 52 faces (20 triangles, 12 pentagrams, and 20 hexagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the small rhombidodecahedron is a nonconvex uniform polyhedron, indexed as U39. It has 42 faces (30 squares and 12 decagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the great cubicuboctahedron is a nonconvex uniform polyhedron, indexed as U14. It has 20 faces (8 triangles, 6 squares and 6 octagrams), 48 edges, and 24 vertices. Its square faces and its octagrammic faces are parallel to those of a cube, while its triangular faces are parallel to those of an octahedron: hence the name cubicuboctahedron. The great suffix serves to distinguish it from the small cubicuboctahedron, which also has faces in the aforementioned directions.

In geometry, the truncated great dodecahedron is a nonconvex uniform polyhedron, indexed as U37. It has 24 faces (12 pentagrams and 12 decagons), 90 edges, and 60 vertices. It is given a Schläfli symbol t{5,5/2}.
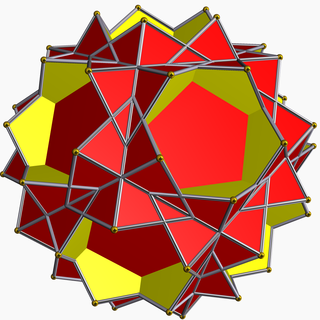
In geometry, the small stellated truncated dodecahedron (or quasitruncated small stellated dodecahedron or small stellatruncated dodecahedron) is a nonconvex uniform polyhedron, indexed as U58. It has 24 faces (12 pentagons and 12 decagrams), 90 edges, and 60 vertices. It is given a Schläfli symbol t{5⁄3,5}, and Coxeter diagram .

In geometry, the great ditrigonal dodecicosidodecahedron (or great dodekified icosidodecahedron) is a nonconvex uniform polyhedron, indexed as U42. It has 44 faces (20 triangles, 12 pentagons, and 12 decagrams), 120 edges, and 60 vertices. Its vertex figure is an isosceles trapezoid.
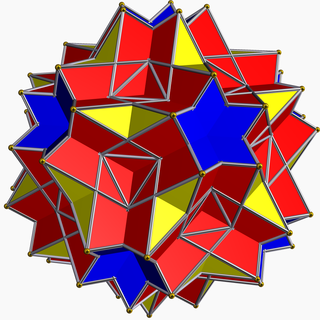
In geometry, the great dodecicosidodecahedron (or great dodekicosidodecahedron) is a nonconvex uniform polyhedron, indexed as U61. It has 44 faces (20 triangles, 12 pentagrams and 12 decagrams), 120 edges and 60 vertices.

In geometry, the rhombidodecadodecahedron is a nonconvex uniform polyhedron, indexed as U38. It has 54 faces (30 squares, 12 pentagons and 12 pentagrams), 120 edges and 60 vertices. It is given a Schläfli symbol t0,2{5⁄2,5}, and by the Wythoff construction this polyhedron can also be named a cantellated great dodecahedron.
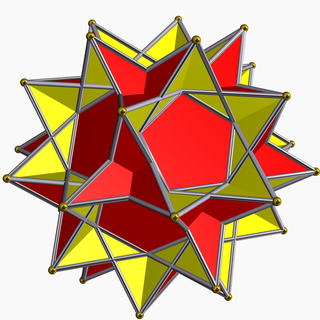
In geometry, the great dodecahemidodecahedron is a nonconvex uniform polyhedron, indexed as U70. It has 18 faces (12 pentagrams and 6 decagrams), 60 edges, and 30 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the small ditrigonal dodecicosidodecahedron (or small dodekified icosidodecahedron) is a nonconvex uniform polyhedron, indexed as U43. It has 44 faces (20 triangles, 12 pentagrams and 12 decagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.

In geometry, the nonconvex great rhombicosidodecahedron is a nonconvex uniform polyhedron, indexed as U67. It has 62 faces (20 triangles, 30 squares and 12 pentagrams), 120 edges, and 60 vertices. It is also called the quasirhombicosidodecahedron. It is given a Schläfli symbol rr{5⁄3,3}. Its vertex figure is a crossed quadrilateral.
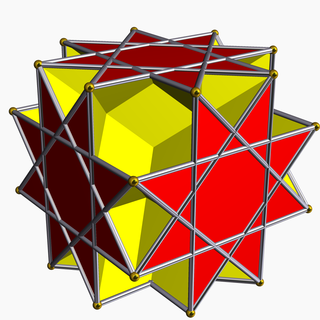
In geometry, the great rhombihexahedron (or great rhombicube) is a nonconvex uniform polyhedron, indexed as U21. It has 18 faces (12 squares and 6 octagrams), 48 edges, and 24 vertices. Its dual is the great rhombihexacron. Its vertex figure is a crossed quadrilateral.
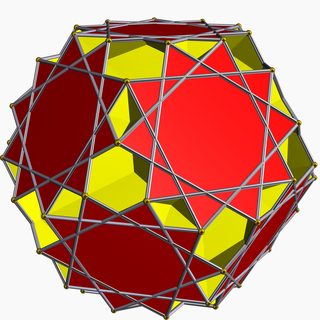
In geometry, the great dodecicosahedron (or great dodekicosahedron) is a nonconvex uniform polyhedron, indexed as U63. It has 32 faces (20 hexagons and 12 decagrams), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.