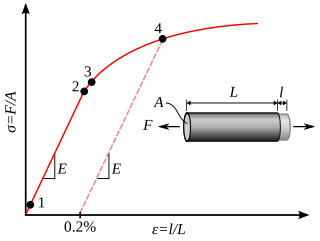
In physics and materials science, plasticity is the ability of a solid material to undergo permanent deformation, a non-reversible change of shape in response to applied forces. For example, a solid piece of metal being bent or pounded into a new shape displays plasticity as permanent changes occur within the material itself. In engineering, the transition from elastic behavior to plastic behavior is known as yielding.

In materials science, creep is the tendency of a solid material to undergo slow deformation while subject to persistent mechanical stresses. It can occur as a result of long-term exposure to high levels of stress that are still below the yield strength of the material. Creep is more severe in materials that are subjected to heat for long periods and generally increases as they near their melting point.

In materials science, a dislocation or Taylor's dislocation is a linear crystallographic defect or irregularity within a crystal structure that contains an abrupt change in the arrangement of atoms. The movement of dislocations allow atoms to slide over each other at low stress levels and is known as glide or slip. The crystalline order is restored on either side of a glide dislocation but the atoms on one side have moved by one position. The crystalline order is not fully restored with a partial dislocation. A dislocation defines the boundary between slipped and unslipped regions of material and as a result, must either form a complete loop, intersect other dislocations or defects, or extend to the edges of the crystal. A dislocation can be characterised by the distance and direction of movement it causes to atoms which is defined by the Burgers vector. Plastic deformation of a material occurs by the creation and movement of many dislocations. The number and arrangement of dislocations influences many of the properties of materials.

Crystal twinning occurs when two or more adjacent crystals of the same mineral are oriented so that they share some of the same crystal lattice points in a symmetrical manner. The result is an intergrowth of two separate crystals that are tightly bonded to each other. The surface along which the lattice points are shared in twinned crystals is called a composition surface or twin plane.
The stacking-fault energy (SFE) is a materials property on a very small scale. It is noted as γSFE in units of energy per area.

In materials science, critical resolved shear stress (CRSS) is the component of shear stress, resolved in the direction of slip, necessary to initiate slip in a grain. Resolved shear stress (RSS) is the shear component of an applied tensile or compressive stress resolved along a slip plane that is other than perpendicular or parallel to the stress axis. The RSS is related to the applied stress by a geometrical factor, m, typically the Schmid factor:

In materials science, slip is the large displacement of one part of a crystal relative to another part along crystallographic planes and directions. Slip occurs by the passage of dislocations on close/packed planes, which are planes containing the greatest number of atoms per area and in close-packed directions. Close-packed planes are known as slip or glide planes. A slip system describes the set of symmetrically identical slip planes and associated family of slip directions for which dislocation motion can easily occur and lead to plastic deformation. The magnitude and direction of slip are represented by the Burgers vector, b.
In metallurgy, solid solution strengthening is a type of alloying that can be used to improve the strength of a pure metal. The technique works by adding atoms of one element to the crystalline lattice of another element, forming a solid solution. The local nonuniformity in the lattice due to the alloying element makes plastic deformation more difficult by impeding dislocation motion through stress fields. In contrast, alloying beyond the solubility limit can form a second phase, leading to strengthening via other mechanisms.

In materials science, the Burgers vector, named after Dutch physicist Jan Burgers, is a vector, often denoted as b, that represents the magnitude and direction of the lattice distortion resulting from a dislocation in a crystal lattice.
A deformation mechanism, in geology, is a process occurring at a microscopic scale that is responsible for changes in a material's internal structure, shape and volume. The process involves planar discontinuity and/or displacement of atoms from their original position within a crystal lattice structure. These small changes are preserved in various microstructures of materials such as rocks, metals and plastics, and can be studied in depth using optical or digital microscopy.
Methods have been devised to modify the yield strength, ductility, and toughness of both crystalline and amorphous materials. These strengthening mechanisms give engineers the ability to tailor the mechanical properties of materials to suit a variety of different applications. For example, the favorable properties of steel result from interstitial incorporation of carbon into the iron lattice. Brass, a binary alloy of copper and zinc, has superior mechanical properties compared to its constituent metals due to solution strengthening. Work hardening has also been used for centuries by blacksmiths to introduce dislocations into materials, increasing their yield strengths.

In materials science, a Frank–Read source is a mechanism explaining the generation of multiple dislocations in specific well-spaced slip planes in crystals when they are deformed. When a crystal is deformed, in order for slip to occur, dislocations must be generated in the material. This implies that, during deformation, dislocations must be primarily generated in these planes. Cold working of metal increases the number of dislocations by the Frank–Read mechanism. Higher dislocation density increases yield strength and causes work hardening of metals.
Dislocation creep is a deformation mechanism in crystalline materials. Dislocation creep involves the movement of dislocations through the crystal lattice of the material, in contrast to diffusion creep, in which diffusion is the dominant creep mechanism. It causes plastic deformation of the individual crystals, and thus the material itself.
In materials science, the yield strength anomaly refers to materials wherein the yield strength increases with temperature. For the majority of materials, the yield strength decreases with increasing temperature. In metals, this decrease in yield strength is due to the thermal activation of dislocation motion, resulting in easier plastic deformation at higher temperatures.
Dynamic strain aging (DSA) for materials science is an instability in plastic flow of materials, associated with interaction between moving dislocations and diffusing solutes. Although sometimes dynamic strain aging is used interchangeably with the Portevin–Le Chatelier effect, dynamic strain aging refers specifically to the microscopic mechanism that induces the Portevin–Le Chatelier effect. This strengthening mechanism is related to solid-solution strengthening and has been observed in a variety of fcc and bcc substitutional and interstitial alloys, metalloids like silicon, and ordered intermetallics within specific ranges of temperature and strain rate.
In materials science, a partial dislocation is a decomposed form of dislocation that occurs within a crystalline material. An extended dislocation is a dislocation that has dissociated into a pair of partial dislocations. The vector sum of the Burgers vectors of the partial dislocations is the Burgers vector of the extended dislocation.

In crystallography, a stacking fault is a planar defect that can occur in crystalline materials. Crystalline materials form repeating patterns of layers of atoms. Errors can occur in the sequence of these layers and are known as stacking faults. Stacking faults are in a higher energy state which is quantified by the formation enthalpy per unit area called the stacking-fault energy. Stacking faults can arise during crystal growth or from plastic deformation. In addition, dislocations in low stacking-fault energy materials typically dissociate into an extended dislocation, which is a stacking fault bounded by partial dislocations.
Geometrically necessary dislocations are like-signed dislocations needed to accommodate for plastic bending in a crystalline material. They are present when a material's plastic deformation is accompanied by internal plastic strain gradients. They are in contrast to statistically stored dislocations, with statistics of equal positive and negative signs, which arise during plastic flow from multiplication processes like the Frank-Read source.
Kinks are deviations of a dislocation defect along its glide plane. In edge dislocations, the constant glide plane allows short regions of the dislocation to turn, converting into screw dislocations and producing kinks. Screw dislocations have rotatable glide planes, thus kinks that are generated along screw dislocations act as an anchor for the glide plane. Kinks differ from jogs in that kinks are strictly parallel to the glide plane, while jogs shift away from the glide plane.
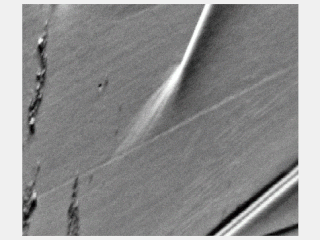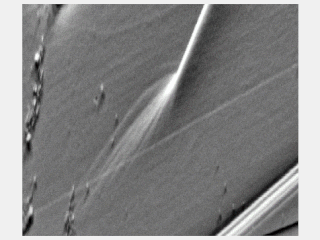
Slip bands or stretcher-strain marks are localized bands of plastic deformation in metals experiencing stresses. Formation of slip bands indicates a concentrated unidirectional slip on certain planes causing a stress concentration. Typically, slip bands induce surface steps and a stress concentration which can be a crack nucleation site. Slip bands extend until impinged by a boundary, and the generated stress from dislocations pile-up against that boundary will either stop or transmit the operating slip depening on its (mis)orientation.









