Combinatorics is an area of mathematics primarily concerned with counting, both as a means and as an end to obtaining results, and certain properties of finite structures. It is closely related to many other areas of mathematics and has many applications ranging from logic to statistical physics and from evolutionary biology to computer science.

Discrete mathematics is the study of mathematical structures that can be considered "discrete" rather than "continuous". Objects studied in discrete mathematics include integers, graphs, and statements in logic. By contrast, discrete mathematics excludes topics in "continuous mathematics" such as real numbers, calculus or Euclidean geometry. Discrete objects can often be enumerated by integers; more formally, discrete mathematics has been characterized as the branch of mathematics dealing with countable sets. However, there is no exact definition of the term "discrete mathematics".
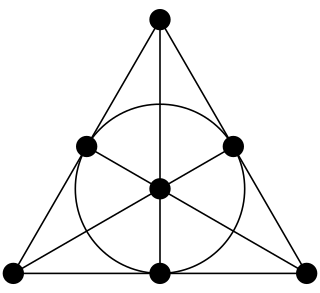
In combinatorial mathematics, a Steiner system is a type of block design, specifically a t-design with λ = 1 and t = 2 or (recently) t ≥ 2.
Combinatorics is a branch of mathematics concerning the study of finite or countable discrete structures.
Scott A. Vanstone was a mathematician and cryptographer in the University of Waterloo Faculty of Mathematics. He was a member of the school's Centre for Applied Cryptographic Research, and was also a founder of the cybersecurity company Certicom. He received his PhD in 1974 at the University of Waterloo, and for about a decade worked principally in combinatorial design theory, finite geometry, and finite fields. In the 1980s he started working in cryptography. An early result of Vanstone was an improved algorithm for computing discrete logarithms in binary fields, which inspired Don Coppersmith to develop his famous exp(n^{1/3+ε}) algorithm.

In mathematics, an incidence structure is an abstract system consisting of two types of objects and a single relationship between these types of objects. Consider the points and lines of the Euclidean plane as the two types of objects and ignore all the properties of this geometry except for the relation of which points are on which lines for all points and lines. What is left is the incidence structure of the Euclidean plane.

Raj Chandra Bose (or Basu) (19 June 1901 – 31 October 1987) was an Indian American mathematician and statistician best known for his work in design theory, finite geometry and the theory of error-correcting codes in which the class of BCH codes is partly named after him. He also invented the notions of partial geometry, association scheme, and strongly regular graph and started a systematic study of difference sets to construct symmetric block designs. He was notable for his work along with S. S. Shrikhande and E. T. Parker in their disproof of the famous conjecture made by Leonhard Euler dated 1782 that for no n do there exist two mutually orthogonal Latin squares of order 4n + 2.
In combinatorial mathematics, a block design is an incidence structure consisting of a set together with a family of subsets known as blocks, chosen such that frequency of the elements satisfies certain conditions making the collection of blocks exhibit symmetry (balance). Block designs have applications in many areas, including experimental design, finite geometry, physical chemistry, software testing, cryptography, and algebraic geometry.
Combinatorial design theory is the part of combinatorial mathematics that deals with the existence, construction and properties of systems of finite sets whose arrangements satisfy generalized concepts of balance and/or symmetry. These concepts are not made precise so that a wide range of objects can be thought of as being under the same umbrella. At times this might involve the numerical sizes of set intersections as in block designs, while at other times it could involve the spatial arrangement of entries in an array as in sudoku grids.
In combinatorics, a difference set is a subset of size of a group of order such that every non-identity element of can be expressed as a product of elements of in exactly ways. A difference set is said to be cyclic, abelian, non-abelian, etc., if the group has the corresponding property. A difference set with is sometimes called planar or simple. If is an abelian group written in additive notation, the defining condition is that every non-zero element of can be written as a difference of elements of in exactly ways. The term "difference set" arises in this way.
A logical matrix, binary matrix, relation matrix, Boolean matrix, or (0, 1)-matrix is a matrix with entries from the Boolean domain B = {0, 1}. Such a matrix can be used to represent a binary relation between a pair of finite sets. It is an important tool in combinatorial mathematics and theoretical computer science.
In combinatorial mathematics, a Dowling geometry, named after Thomas A. Dowling, is a matroid associated with a group. There is a Dowling geometry of each rank for each group. If the rank is at least 3, the Dowling geometry uniquely determines the group. Dowling geometries have a role in matroid theory as universal objects ; in that respect they are analogous to projective geometries, but based on groups instead of fields.

In (polyhedral) geometry, a flag is a sequence of faces of a polytope, each contained in the next, with exactly one face from each dimension.

Hanfried Lenz was a German mathematician, who is mainly known for his work in geometry and combinatorics.

Algebraic combinatorics is an area of mathematics that employs methods of abstract algebra, notably group theory and representation theory, in various combinatorial contexts and, conversely, applies combinatorial techniques to problems in algebra.
In mathematics, a non-Desarguesian plane is a projective plane that does not satisfy Desargues' theorem, or in other words a plane that is not a Desarguesian plane. The theorem of Desargues is true in all projective spaces of dimension not 2; in other words, the only projective spaces of dimension not equal to 2 are the classical projective geometries over a field. However, David Hilbert found that some projective planes do not satisfy it. The current state of knowledge of these examples is not complete.
Mathematics is a broad subject that is commonly divided in many areas that may be defined by their objects of study, by the used methods, or by both. For example, analytic number theory is a subarea of number theory devoted to the use of methods of analysis for the study of natural numbers.

James William Peter Hirschfeld is an Australian mathematician, resident in the United Kingdom, specializing in combinatorial geometry and the geometry of finite fields. He is an emeritus professor and Tutorial Fellow at the University of Sussex.
Combinatorics of Experimental Design is a textbook on the design of experiments, a subject that connects applications in statistics to the theory of combinatorial mathematics. It was written by mathematician Anne Penfold Street and her daughter, statistician Deborah Street, and published in 1987 by the Oxford University Press under their Clarendon Press imprint.
In geometry, a truncated projective plane (TPP), also known as a dual affine plane, is a special kind of a hypergraph or geometric configuration that is constructed in the following way.







