Related Research Articles

Alexander Grothendieck was a German-born mathematician who became the leading figure in the creation of modern algebraic geometry. His research extended the scope of the field and added elements of commutative algebra, homological algebra, sheaf theory, and category theory to its foundations, while his so-called "relative" perspective led to revolutionary advances in many areas of pure mathematics. He is considered by many to be the greatest mathematician of the twentieth century.

Category theory is a general theory of mathematical structures and their relations. It was introduced by Samuel Eilenberg and Saunders Mac Lane in the middle of the 20th century in their foundational work on algebraic topology. Category theory is used in almost all areas of mathematics. In particular, many constructions of new mathematical objects from previous ones that appear similarly in several contexts are conveniently expressed and unified in terms of categories. Examples include quotient spaces, direct products, completion, and duality.
In category theory, a branch of mathematics, a Grothendieck topology is a structure on a category C that makes the objects of C act like the open sets of a topological space. A category together with a choice of Grothendieck topology is called a site.
In mathematics, pointless topology, also called point-free topology and locale theory, is an approach to topology that avoids mentioning points, and in which the lattices of open sets are the primitive notions. In this approach it becomes possible to construct topologically interesting spaces from purely algebraic data.
Topology is the part of mathematics concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself.

In mathematics, a category is a collection of "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose objects are sets and whose arrows are functions.
In mathematics, a sheaf is a tool for systematically tracking data attached to the open sets of a topological space and defined locally with regard to them. For example, for each open set, the data could be the ring of continuous functions defined on that open set. Such data are well behaved in that they can be restricted to smaller open sets, and also the data assigned to an open set are equivalent to all collections of compatible data assigned to collections of smaller open sets covering the original open set.
This article gives some very general background to the mathematical idea of topos. This is an aspect of category theory, and has a reputation for being abstruse. The level of abstraction involved cannot be reduced beyond a certain point; but on the other hand context can be given. This is partly in terms of historical development, but also to some extent an explanation of differing attitudes to category theory.
Noncommutative geometry (NCG) is a branch of mathematics concerned with a geometric approach to noncommutative algebras, and with the construction of spaces that are locally presented by noncommutative algebras of functions, possibly in some generalized sense. A noncommutative algebra is an associative algebra in which the multiplication is not commutative, that is, for which does not always equal ; or more generally an algebraic structure in which one of the principal binary operations is not commutative; one also allows additional structures, e.g. topology or norm, to be possibly carried by the noncommutative algebra of functions.
The following outline is provided as an overview of and guide to category theory, the area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows, where these collections satisfy certain basic conditions. Many significant areas of mathematics can be formalised as categories, and the use of category theory allows many intricate and subtle mathematical results in these fields to be stated, and proved, in a much simpler way than without the use of categories.
In category theory, a subobject classifier is a special object Ω of a category such that, intuitively, the subobjects of any object X in the category correspond to the morphisms from X to Ω. In typical examples, that morphism assigns "true" to the elements of the subobject and "false" to the other elements of X. Therefore, a subobject classifier is also known as a "truth value object" and the concept is widely used in the categorical description of logic. Note however that subobject classifiers are often much more complicated than the simple binary logic truth values {true, false}.

Francis William Lawvere was an American mathematician known for his work in category theory, topos theory and the philosophy of mathematics.
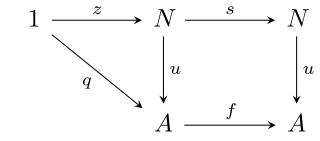
In category theory, a natural numbers object (NNO) is an object endowed with a recursive structure similar to natural numbers. More precisely, in a category E with a terminal object 1, an NNO N is given by:
- a global element z : 1 → N, and
- an arrow s : N → N,
Categorical logic is the branch of mathematics in which tools and concepts from category theory are applied to the study of mathematical logic. It is also notable for its connections to theoretical computer science. In broad terms, categorical logic represents both syntax and semantics by a category, and an interpretation by a functor. The categorical framework provides a rich conceptual background for logical and type-theoretic constructions. The subject has been recognisable in these terms since around 1970.
In mathematics, a *-autonomous category C is a symmetric monoidal closed category equipped with a dualizing object . The concept is also referred to as Grothendieck—Verdier category in view of its relation to the notion of Verdier duality.

In mathematics, a space is a set with a definition (structure) of relationships among the elements of the set. While modern mathematics uses many types of spaces, such as Euclidean spaces, linear spaces, topological spaces, Hilbert spaces, or probability spaces, it does not define the notion of "space" itself.

Steve Vickers is a British mathematician and computer scientist. In the early 1980s, he wrote ROM firmware and manuals for three home computers, the ZX81, ZX Spectrum, and Jupiter Ace. The latter was produced by Jupiter Cantab, a short-lived company Vickers formed together with Richard Altwasser, after the two had left Sinclair Research. Since the late 1980s, Vickers has been an academic in the field of geometric logic, writing over 30 papers in scholarly journals on mathematical aspects of computer science. His book Topology via Logic has been influential over a range of fields. In October 2018, he retired as senior lecturer at the University of Birmingham. As announced on his university homepage, he continues to supervise PhD students at the university and focus on his research.
In mathematics, a topos is a category that behaves like the category of sheaves of sets on a topological space. Topoi behave much like the category of sets and possess a notion of localization; they are a direct generalization of point-set topology. The Grothendieck topoi find applications in algebraic geometry; the more general elementary topoi are used in logic.
Mathematics is a broad subject that is commonly divided in many areas that may be defined by their objects of study, by the used methods, or by both. For example, analytic number theory is a subarea of number theory devoted to the use of methods of analysis for the study of natural numbers.
In mathematics, a ringed topos is a generalization of a ringed space; that is, the notion is obtained by replacing a "topological space" by a "topos". The notion of a ringed topos has applications to deformation theory in algebraic geometry and the mathematical foundation of quantum mechanics. In the latter subject, a Bohr topos is a ringed topos that plays the role of a quantum phase space.
References
- ↑ Grothendieck & Verdier 1972
- ↑ Lawvere 1975
- ↑ "Locales as geometric objects". MathOverflow. Retrieved 2024-07-22.
- ↑ Johnstone 1985
- ↑ "On a Topological Topos at The n-Category Café". golem.ph.utexas.edu.
- Lawvere, F. William (1975). "Continuously Variable Sets; Algebraic Geometry = Geometric Logic". Logic Colloquium '73, Proceedings of the Logic Colloquium. Studies in Logic and the Foundations of Mathematics. Vol. 80. pp. 135–156. doi:10.1016/S0049-237X(08)71947-5. ISBN 978-0-444-10642-1.
- Lawvere, Categories of spaces may not be generalized spaces as exemplified by directed graphs
- Johnstone, Peter T. (1985). "How general is a generalized space?". Aspects of Topology. pp. 77–112. doi:10.1017/CBO9781107359925.004. ISBN 978-0-521-27815-7.
- Grothendieck, A.; Verdier, J. L. (1972), "Topos", Théorie des Topos et Cohomologie Etale des Schémas, Lecture Notes in Mathematics, vol. 269, Springer, doi:10.1007/BFb0081555