Related Research Articles

In probability theory and related fields, a stochastic or random process is a mathematical object usually defined as a sequence of random variables in a probability space, where the index of the sequence often has the interpretation of time. Stochastic processes are widely used as mathematical models of systems and phenomena that appear to vary in a random manner. Examples include the growth of a bacterial population, an electrical current fluctuating due to thermal noise, or the movement of a gas molecule. Stochastic processes have applications in many disciplines such as biology, chemistry, ecology, neuroscience, physics, image processing, signal processing, control theory, information theory, computer science, and telecommunications. Furthermore, seemingly random changes in financial markets have motivated the extensive use of stochastic processes in finance.
Stochastic calculus is a branch of mathematics that operates on stochastic processes. It allows a consistent theory of integration to be defined for integrals of stochastic processes with respect to stochastic processes. This field was created and started by the Japanese mathematician Kiyosi Itô during World War II.
In probability theory and related fields, Malliavin calculus is a set of mathematical techniques and ideas that extend the mathematical field of calculus of variations from deterministic functions to stochastic processes. In particular, it allows the computation of derivatives of random variables. Malliavin calculus is also called the stochastic calculus of variations. P. Malliavin first initiated the calculus on infinite dimensional space. Then, the significant contributors such as S. Kusuoka, D. Stroock, J-M. Bismut, Shinzo Watanabe, I. Shigekawa, and so on finally completed the foundations.

Kiyosi Itô was a Japanese mathematician who made fundamental contributions to probability theory, in particular, the theory of stochastic processes. He invented the concept of stochastic integral and stochastic differential equation, and is known as the founder of so-called Itô calculus. He also pioneered the world connections between stochastic calculus and differential geometry, known as stochastic differential geometry, invited for the ICM in Stockholm.
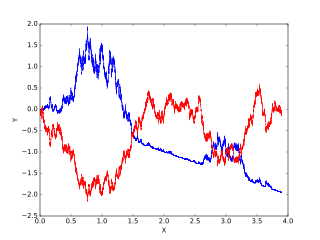
Itô calculus, named after Kiyosi Itô, extends the methods of calculus to stochastic processes such as Brownian motion. It has important applications in mathematical finance and stochastic differential equations.
Bernt Karsten Øksendal is a Norwegian mathematician. He completed his undergraduate studies at the University of Oslo, working under Otte Hustad. He obtained his PhD from University of California, Los Angeles in 1971; his thesis was titled Peak Sets and Interpolation Sets for Some Algebras of Analytic Functions and was supervised by Theodore Gamelin. In 1991, he was appointed as a professor at the University of Oslo. In 1992, he was appointed as an adjunct professor at the Norwegian School of Economics and Business Administration, Bergen, Norway.

Paul Malliavin was a French mathematician who made important contributions to harmonic analysis and stochastic analysis. He is known for the Malliavin calculus, an infinite dimensional calculus for functionals on the Wiener space and his probabilistic proof of Hörmander's theorem. He was Professor at the Pierre and Marie Curie University and a member of the French Academy of Sciences from 1979 to 2010.

Jean-Michel Bismut is a French mathematician who has been a professor at the Université Paris-Sud since 1981. His mathematical career covers two apparently different branches of mathematics: probability theory and differential geometry. Ideas from probability play an important role in his works on geometry.

Moshe Zakai was a Distinguished Professor at the Technion, Israel in electrical engineering, member of the Israel Academy of Sciences and Humanities and Rothschild Prize winner.

Peng Shige is a Chinese mathematician noted for his contributions in stochastic analysis and mathematical finance.
Quantitative analysis is the use of mathematical and statistical methods in finance and investment management. Those working in the field are quantitative analysts (quants). Quants tend to specialize in specific areas which may include derivative structuring or pricing, risk management, investment management and other related finance occupations. The occupation is similar to those in industrial mathematics in other industries. The process usually consists of searching vast databases for patterns, such as correlations among liquid assets or price-movement patterns.
Mathematical finance, also known as quantitative finance and financial mathematics, is a field of applied mathematics, concerned with mathematical modeling of financial markets.
Mark Herbert Ainsworth Davis was Professor of Mathematics at Imperial College London. He made fundamental contributions to the theory of stochastic processes, stochastic control and mathematical finance.
David Nualart is a Spanish mathematician working in the field of probability theory, in particular on aspects of stochastic processes and stochastic analysis.
Marta Sanz-Solé is a Spanish mathematician specializing in probability theory. She obtained her PhD in 1978 from the University of Barcelona under the supervision of David Nualart.
Shinzō Watanabe is a Japanese mathematician, who has made fundamental contributions to probability theory, stochastic processes and stochastic differential equations. He is regarded and revered as one of the fundamental contributors to the modern probability theory and Stochastic calculus. The pioneering book “Stochastic Differential Equations and Diffusion Processes” he wrote with Nobuyuki Ikeda has attracted a lot of researchers into the area and is known as the “Ikeda-Watanabe” for researchers in the field of stochastic analysis. He had been served as the editor of Springer Mathematics.
Yuliya Stepanivna Mishura is a Ukrainian mathematician specializing in probability theory and mathematical finance. She is a professor at the Taras Shevchenko National University of Kyiv.
Francesca Biagini is a German and Italian mathematician specializing in mathematical finance, stochastic calculus, and probability theory. Topics in her research include fractional Brownian motion and portfolio optimization for inside traders. She is a professor of applied mathematics and vice president for international affairs and diversity at Ludwig Maximilian University of Munich, and president of the Bachelier Finance Society.
Agnès Sulem is a French applied mathematician whose research topics include stochastic control, jump diffusion, and mathematical finance.

Nizar Touzi is a Tunisian-French mathematician. He is a professor of applied mathematics at École polytechnique. His research focuses on analysis, statistics and algebra. He is being known for publications on optimization and stochastic control.
References
- 1 2 3 ICIAM Prizes, International Council for Industrial and Applied Mathematics, 2018
- 1 2 3 4 Curriculum vitae , retrieved 2018-10-11
- ↑ Giulia Di Nunno at the Mathematics Genealogy Project
- ↑ Privault, Nicolas (2010), "Review of Malliavin calculus for Lévy processes", Mathematical Reviews, MR 2460554