In number theory, an arithmetic, arithmetical, or number-theoretic function is generally any function whose domain is the set of positive integers and whose range is a subset of the complex numbers. Hardy & Wright include in their definition the requirement that an arithmetical function "expresses some arithmetical property of n". There is a larger class of number-theoretic functions that do not fit this definition, for example, the prime-counting functions. This article provides links to functions of both classes.

In number theory, a Carmichael number is a composite number which in modular arithmetic satisfies the congruence relation:
In number theory, a multiplicative function is an arithmetic function f(n) of a positive integer n with the property that f(1) = 1 and whenever a and b are coprime.

In number theory, a weird number is a natural number that is abundant but not semiperfect. In other words, the sum of the proper divisors of the number is greater than the number, but no subset of those divisors sums to the number itself.

In mathematics, an almost perfect number (sometimes also called slightly defective or least deficientnumber) is a natural number n such that the sum of all divisors of n (the sum-of-divisors function σ(n)) is equal to 2n − 1, the sum of all proper divisors of n, s(n) = σ(n) − n, then being equal to n − 1. The only known almost perfect numbers are powers of 2 with non-negative exponents (sequence A000079 in the OEIS). Therefore the only known odd almost perfect number is 20 = 1, and the only known even almost perfect numbers are those of the form 2k for some positive integer k; however, it has not been shown that all almost perfect numbers are of this form. It is known that an odd almost perfect number greater than 1 would have at least six prime factors.
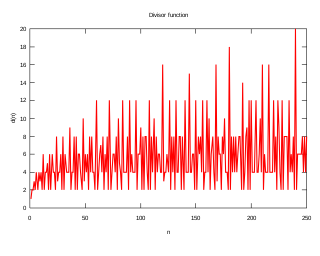
In mathematics, and specifically in number theory, a divisor function is an arithmetic function related to the divisors of an integer. When referred to as the divisor function, it counts the number of divisors of an integer. It appears in a number of remarkable identities, including relationships on the Riemann zeta function and the Eisenstein series of modular forms. Divisor functions were studied by Ramanujan, who gave a number of important congruences and identities; these are treated separately in the article Ramanujan's sum.
In mathematics, an untouchable number is a positive integer that cannot be expressed as the sum of all the proper divisors of any positive integer. That is, these numbers are not in the image of the aliquot sum function. Their study goes back at least to Abu Mansur al-Baghdadi, who observed that both 2 and 5 are untouchable.

In number theory, a practical number or panarithmic number is a positive integer such that all smaller positive integers can be represented as sums of distinct divisors of . For example, 12 is a practical number because all the numbers from 1 to 11 can be expressed as sums of its divisors 1, 2, 3, 4, and 6: as well as these divisors themselves, we have 5 = 3 + 2, 7 = 6 + 1, 8 = 6 + 2, 9 = 6 + 3, 10 = 6 + 3 + 1, and 11 = 6 + 3 + 2.

The Ramanujan tau function, studied by Ramanujan, is the function :\mathbb {N} \rightarrow \mathbb {Z} } defined by the following identity:
In mathematics, a superabundant number is a certain kind of natural number. A natural number n is called superabundant precisely when, for all m < n:

In number theory, a colossally abundant number is a natural number that, in a particular, rigorous sense, has many divisors. Particularly, it is defined by a ratio between the sum of an integer's divisors and that integer raised to a power higher than one. For any such exponent, whichever integer has the highest ratio is a colossally abundant number. It is a stronger restriction than that of a superabundant number, but not strictly stronger than that of an abundant number.

In number theory, a highly abundant number is a natural number with the property that the sum of its divisors is greater than the sum of the divisors of any smaller natural number.
A Fibonacci prime is a Fibonacci number that is prime, a type of integer sequence prime.
In mathematics, a Kloosterman sum is a particular kind of exponential sum. They are named for the Dutch mathematician Hendrik Kloosterman, who introduced them in 1926 when he adapted the Hardy–Littlewood circle method to tackle a problem involving positive definite diagonal quadratic forms in four variables, strengthening his 1924 dissertation research on five or more variables.
In number theory, friendly numbers are two or more natural numbers with a common abundancy index, the ratio between the sum of divisors of a number and the number itself. Two numbers with the same "abundancy" form a friendly pair; n numbers with the same abundancy form a friendly n-tuple.
In number theory, Ramanujan's sum, usually denoted cq(n), is a function of two positive integer variables q and n defined by the formula
In number theory, the aliquot sums(n) of a positive integer n is the sum of all proper divisors of n, that is, all divisors of n other than n itself. That is,
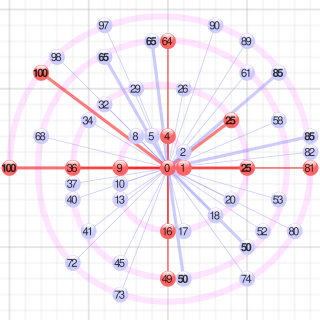
In number theory, the sum of two squares theorem relates the prime decomposition of any integer n > 1 to whether it can be written as a sum of two squares, such that n = a2 + b2 for some integers a, b.
An integer greater than one can be written as a sum of two squares if and only if its prime decomposition contains no factor pk, where prime and k is odd.
This is a glossary of concepts and results in number theory, a field of mathematics. Concepts and results in arithmetic geometry and diophantine geometry can be found in Glossary of arithmetic and diophantine geometry.














