| Great pentagrammic hexecontahedron | |
|---|---|
 | |
| Type | Star polyhedron |
| Face |  |
| Elements | F = 60, E = 150 V = 92 (χ = 2) |
| Symmetry group | I, [5,3]+, 532 |
| Index references | DU 74 |
| dual polyhedron | Great retrosnub icosidodecahedron |
In geometry, the great pentagrammic hexecontahedron (or great dentoid ditriacontahedron) is a nonconvex isohedral polyhedron. It is the dual of the great retrosnub icosidodecahedron. Its 60 faces are irregular pentagrams.
Denote the golden ratio by . Let be the largest positive zero of the polynomial . Then each pentagrammic face has four equal angles of and one angle of . Each face has three long and two short edges. The ratio between the lengths of the long and the short edges is given by
The dihedral angle equals . Part of each face lies inside the solid, hence is invisible in solid models. The other two zeroes of the polynomial play a similar role in the description of the great pentagonal hexecontahedron and the great inverted pentagonal hexecontahedron.
In geometry, the snub dodecahedron, or snub icosidodecahedron, is an Archimedean solid, one of thirteen convex isogonal nonprismatic solids constructed by two or more types of regular polygon faces.
In geometry, the triakis icosahedron is an Archimedean dual solid, or a Catalan solid. Its dual is the truncated dodecahedron.
In geometry, a pentakis dodecahedron or kisdodecahedron is the polyhedron created by attaching a pentagonal pyramid to each face of a regular dodecahedron; that is, it is the Kleetope of the dodecahedron. It is a Catalan solid, meaning that it is a dual of an Archimedean solid, in this case, the truncated icosahedron.
In geometry, a pentagonal hexecontahedron is a Catalan solid, dual of the snub dodecahedron. It has two distinct forms, which are mirror images of each other. It has 92 vertices that span 60 pentagonal faces. It is the Catalan solid with the most vertices. Among the Catalan and Archimedean solids, it has the second largest number of vertices, after the truncated icosidodecahedron, which has 120 vertices.
In geometry, the small dodecicosidodecahedron (or small dodekicosidodecahedron) is a nonconvex uniform polyhedron, indexed as U33. It has 44 faces (20 triangles, 12 pentagons, and 12 decagons), 120 edges, and 60 vertices. Its vertex figure is a crossed quadrilateral.
In geometry, the great snub icosidodecahedron is a nonconvex uniform polyhedron, indexed as U57. It has 92 faces (80 triangles and 12 pentagrams), 150 edges, and 60 vertices. It can be represented by a Schläfli symbol sr{5⁄2,3}, and Coxeter-Dynkin diagram .
In geometry, the inverted snub dodecadodecahedron (or vertisnub dodecadodecahedron) is a nonconvex uniform polyhedron, indexed as U60. It is given a Schläfli symbol sr{5/3,5}.
In geometry, the great inverted snub icosidodecahedron (or great vertisnub icosidodecahedron) is a uniform star polyhedron, indexed as U69. It is given a Schläfli symbol sr{5⁄3,3}, and Coxeter-Dynkin diagram . In the book Polyhedron Models by Magnus Wenninger, the polyhedron is misnamed great snub icosidodecahedron, and vice versa.
In geometry, the medial rhombic triacontahedron is a nonconvex isohedral polyhedron. It is a stellation of the rhombic triacontahedron, and can also be called small stellated triacontahedron. Its dual is the dodecadodecahedron.
In geometry, the great rhombic triacontahedron is a nonconvex isohedral, isotoxal polyhedron. It is the dual of the great icosidodecahedron (U54). Like the convex rhombic triacontahedron it has 30 rhombic faces, 60 edges and 32 vertices.
In geometry, the great icosacronic hexecontahedron is the dual of the great icosicosidodecahedron. Its faces are darts. A part of each dart lies inside the solid, hence is invisible in solid models.
In geometry, the medial deltoidal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the rhombidodecadodecahedron. Its 60 intersecting quadrilateral faces are kites.
In geometry, the medial pentagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the snub dodecadodecahedron. It has 60 intersecting irregular pentagonal faces.
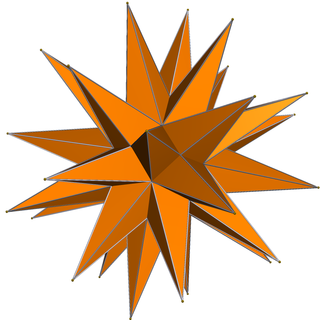
In geometry, the great deltoidal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the nonconvex great rhombicosidodecahedron. It is visually identical to the great rhombidodecacron. It has 60 intersecting cross quadrilateral faces, 120 edges, and 62 vertices. Its faces are darts. Part of each dart lies inside the solid, hence is invisible in solid models.
In geometry, the great rhombidodecacron is a nonconvex isohedral polyhedron. It is the dual of the great rhombidodecahedron. It is visually identical to the great deltoidal hexecontahedron. Its faces are antiparallelograms.
In geometry, the small hexagrammic hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the small retrosnub icosicosidodecahedron. It is partially degenerate, having coincident vertices, as its dual has coplanar triangular faces.
In geometry, the great hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform great snub dodecicosidodecahedron. It is partially degenerate, having coincident vertices, as its dual has coplanar pentagrammic faces.
In geometry, the small hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform small snub icosicosidodecahedron. It is partially degenerate, having coincident vertices, as its dual has coplanar triangular faces.
In geometry, the medial icosacronic hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform icosidodecadodecahedron. Its faces are darts. Part of each dart lies inside the solid, hence is invisible in solid models.
In geometry, the medial hexagonal hexecontahedron is a nonconvex isohedral polyhedron. It is the dual of the uniform snub icosidodecadodecahedron.
 | This polyhedron-related article is a stub. You can help Wikipedia by expanding it. |
