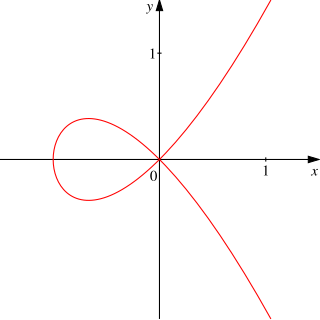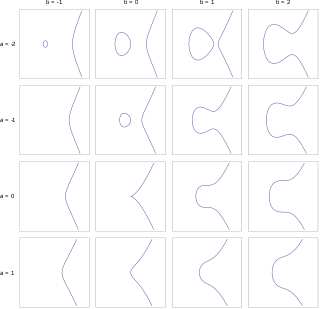
In mathematics, an elliptic curve is a plane algebraic curve defined by an equation of the form
In projective geometry, a plane at infinity is the hyperplane at infinity of a three dimensional projective space or to any plane contained in the hyperplane at infinity of any projective space of higher dimension. This article will be concerned solely with the three-dimensional case.

In mathematics, the linking number is a numerical invariant that describes the linking of two closed curves in three-dimensional space. Intuitively, the linking number represents the number of times that each curve winds around the other. The linking number is always an integer, but may be positive or negative depending on the orientation of the two curves.
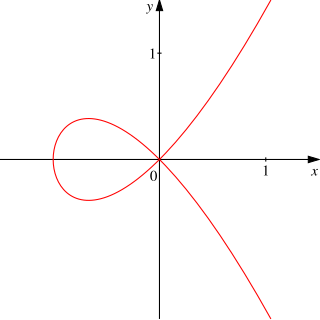
In mathematics, a cubic plane curve is a plane algebraic curve C defined by a cubic equation
In physics, a wave vector is a vector which helps describe a wave. Like any vector, it has a magnitude and direction, both of which are important. Its magnitude is either the wavenumber or angular wavenumber of the wave, and its direction is ordinarily the direction of wave propagation.
In mathematics, a Killing vector field, named after Wilhelm Killing, is a vector field on a Riemannian manifold that preserves the metric. Killing fields are the infinitesimal generators of isometries; that is, flows generated by Killing fields are continuous isometries of the manifold. More simply, the flow generates a symmetry, in the sense that moving each point on an object the same distance in the direction of the Killing vector will not distort distances on the object.
In mathematics, the Weil pairing is a pairing on the points of order dividing n of an elliptic curve E, taking values in nth roots of unity. More generally there is a similar Weil pairing between points of order n of an abelian variety and its dual. It was introduced by André Weil (1940) for Jacobians of curves, who gave an abstract algebraic definition; the corresponding results for elliptic functions were known, and can be expressed simply by use of the Weierstrass sigma function.
Variational Bayesian methods are a family of techniques for approximating intractable integrals arising in Bayesian inference and machine learning. They are typically used in complex statistical models consisting of observed variables as well as unknown parameters and latent variables, with various sorts of relationships among the three types of random variables, as might be described by a graphical model. As typical in Bayesian inference, the parameters and latent variables are grouped together as "unobserved variables". Variational Bayesian methods are primarily used for two purposes:
- To provide an analytical approximation to the posterior probability of the unobserved variables, in order to do statistical inference over these variables.
- To derive a lower bound for the marginal likelihood of the observed data. This is typically used for performing model selection, the general idea being that a higher marginal likelihood for a given model indicates a better fit of the data by that model and hence a greater probability that the model in question was the one that generated the data.
In geometry, the Hessian curve is a plane curve similar to folium of Descartes. It is named after the German mathematician Otto Hesse. This curve was suggested for application in elliptic curve cryptography, because arithmetic in this curve representation is faster and needs less memory than arithmetic in standard Weierstrass form.
In algebraic geometry, supersingular elliptic curves form a certain class of elliptic curves over a field of characteristic p > 0 with unusually large endomorphism rings. Elliptic curves over such fields which are not supersingular are called ordinary and these two classes of elliptic curves behave fundamentally differently in many aspects. Hasse (1936) discovered supersingular elliptic curves during his work on the Riemann hypothesis for elliptic curves by observing that in positive characteristic elliptic curves could have endomorphism rings of unusually large rank 4, and Deuring (1941) developed their basic theory.
In the mathematical theory of bifurcations, a Hopfbifurcation is a critical point where a system's stability switches and a periodic solution arises. More accurately, it is a local bifurcation in which a fixed point of a dynamical system loses stability, as a pair of complex conjugate eigenvalues - of the linearization around the fixed point - crosses the complex plane imaginary axis. Under reasonably generic assumptions about the dynamical system, a small-amplitude limit cycle branches from the fixed point.
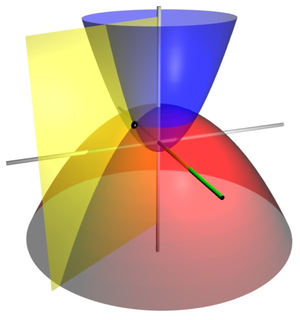
Paraboloidal coordinates are three-dimensional orthogonal coordinates that generalize two-dimensional parabolic coordinates. They possess elliptic paraboloids as one-coordinate surfaces. As such, they should be distinguished from parabolic cylindrical coordinates and parabolic rotational coordinates, both of which are also generalizations of two-dimensional parabolic coordinates. The coordinate surfaces of the former are parabolic cylinders, and the coordinate surfaces of the latter are circular paraboloids.
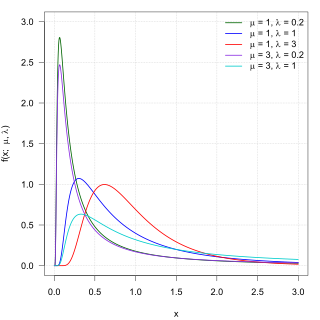
In probability theory, the inverse Gaussian distribution is a two-parameter family of continuous probability distributions with support on (0,∞).

In algebraic geometry, the first polar, or simply polar of an algebraic plane curve C of degree n with respect to a point Q is an algebraic curve of degree n−1 which contains every point of C whose tangent line passes through Q. It is used to investigate the relationship between the curve and its dual, for example in the derivation of the Plücker formulas.
In mathematics, Macdonald polynomialsPλ(x; t,q) are a family of orthogonal symmetric polynomials in several variables, introduced by Macdonald in 1987. He later introduced a non-symmetric generalization in 1995. Macdonald originally associated his polynomials with weights λ of finite root systems and used just one variable t, but later realized that it is more natural to associate them with affine root systems rather than finite root systems, in which case the variable t can be replaced by several different variables t=(t1,...,tk), one for each of the k orbits of roots in the affine root system. The Macdonald polynomials are polynomials in n variables x=(x1,...,xn), where n is the rank of the affine root system. They generalize many other families of orthogonal polynomials, such as Jack polynomials and Hall–Littlewood polynomials and Askey–Wilson polynomials, which in turn include most of the named 1-variable orthogonal polynomials as special cases. Koornwinder polynomials are Macdonald polynomials of certain non-reduced root systems. They have deep relationships with affine Hecke algebras and Hilbert schemes, which were used to prove several conjectures made by Macdonald about them.
In algebraic geometry, the conic sections in the projective plane form a linear system of dimension five, as one sees by counting the constants in the degree two equations. The condition to pass through a given point P imposes a single linear condition, so that conics C through P form a linear system of dimension 4. Other types of condition that are of interest include tangency to a given line L.

In mathematics, a conic section is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties.
In probability theory and statistics, the noncentral beta distribution is a continuous probability distribution that is a generalization of the (central) beta distribution.
In mathematics, the Hessian group is a finite group of order 216, introduced by Jordan (1877) who named it for Otto Hesse. It may be represented as the group of affine transformations with determinant 1 of the affine plane over the field of 3 elements. It has a normal subgroup that is an elementary abelian group of order 32, and the quotient by this subgroup is isomorphic to the group SL2(3) of order 24. It also acts on the Hesse pencil of elliptic curves, and forms the automorphism group of the Hesse configuration of the 9 inflection points of these curves and the 12 lines through triples of these points.

In geometry, two conic sections are called confocal, if they have the same foci. Because ellipses and hyperbolas possess two foci, there are confocal ellipses, confocal hyperbolas and confocal mixtures of ellipses and hyperbolas. In the mixture of confocal ellipses and hyperbolas, any ellipse intersects any hyperbola orthogonally. Parabolas possess only one focus, so, by convention, confocal parabolas have the same focus and the same axis of symmetry. Consequently, any point not on the axis of symmetry lies on two confocal parabolas which intersect orthogonally.