Related Research Articles
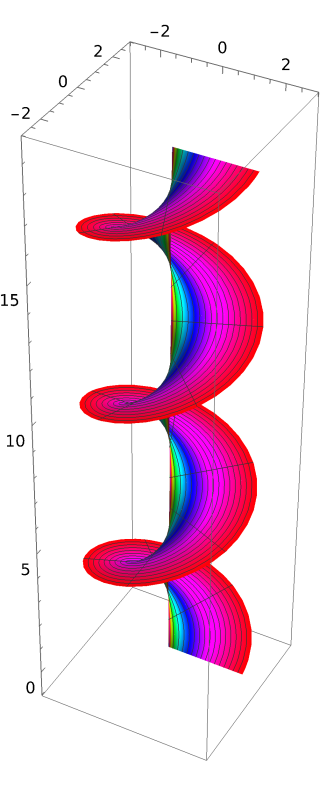
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed versions of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or several sheets glued together.

In algebraic and differential geometry, a Calabi–Yau manifold, also known as a Calabi–Yau space, is a particular type of manifold which has certain properties, such as Ricci flatness, yielding applications in theoretical physics. Particularly in superstring theory, the extra dimensions of spacetime are sometimes conjectured to take the form of a 6-dimensional Calabi–Yau manifold, which led to the idea of mirror symmetry. Their name was coined by Candelas et al. (1985), after Eugenio Calabi, who first conjectured that such surfaces might exist, and Shing-Tung Yau, who proved the Calabi conjecture.

In mathematics, particularly in algebraic geometry, complex analysis and algebraic number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functions. Abelian varieties are at the same time among the most studied objects in algebraic geometry and indispensable tools for research on other topics in algebraic geometry and number theory.
In algebraic geometry, a projective variety is an algebraic variety that is a closed subvariety of a projective space. That is, it is the zero-locus in of some finite family of homogeneous polynomials that generate a prime ideal, the defining ideal of the variety.
In relation to the history of mathematics, the Italian school of algebraic geometry refers to mathematicians and their work in birational geometry, particularly on algebraic surfaces, centered around Rome roughly from 1885 to 1935. There were 30 to 40 leading mathematicians who made major contributions, about half of those being Italian. The leadership fell to the group in Rome of Guido Castelnuovo, Federigo Enriques and Francesco Severi, who were involved in some of the deepest discoveries, as well as setting the style.

David Bryant Mumford is an American mathematician known for his work in algebraic geometry and then for research into vision and pattern theory. He won the Fields Medal and was a MacArthur Fellow. In 2010 he was awarded the National Medal of Science. He is currently a University Professor Emeritus in the Division of Applied Mathematics at Brown University.

In mathematics, a complex analytic K3 surface is a compact connected complex manifold of dimension 2 with а trivial canonical bundle and irregularity zero. An (algebraic) K3 surface over any field means a smooth proper geometrically connected algebraic surface that satisfies the same conditions. In the Enriques–Kodaira classification of surfaces, K3 surfaces form one of the four classes of minimal surfaces of Kodaira dimension zero. A simple example is the Fermat quartic surface
In mathematics, the canonical bundle of a non-singular algebraic variety of dimension over a field is the line bundle , which is the nth exterior power of the cotangent bundle on .
In algebraic geometry, the Kodaira dimensionκ(X) measures the size of the canonical model of a projective variety X.
In algebraic geometry, a surface of general type is an algebraic surface with Kodaira dimension 2. Because of Chow's theorem any compact complex manifold of dimension 2 and with Kodaira dimension 2 will actually be an algebraic surface, and in some sense most surfaces are in this class.
In algebraic geometry, a moduli space of (algebraic) curves is a geometric space whose points represent isomorphism classes of algebraic curves. It is thus a special case of a moduli space. Depending on the restrictions applied to the classes of algebraic curves considered, the corresponding moduli problem and the moduli space is different. One also distinguishes between fine and coarse moduli spaces for the same moduli problem.
In mathematics, the Enriques–Kodaira classification groups compact complex surfaces into ten classes, each parametrized by a moduli space. For most of the classes the moduli spaces are well understood, but for the class of surfaces of general type the moduli spaces seem too complicated to describe explicitly, though some components are known.
In mathematics, an elliptic surface is a surface that has an elliptic fibration, in other words a proper morphism with connected fibers to an algebraic curve such that almost all fibers are smooth curves of genus 1. This is equivalent to the generic fiber being a smooth curve of genus one. This follows from proper base change.
In mathematics, Enriques surfaces are algebraic surfaces such that the irregularity q = 0 and the canonical line bundle K is non-trivial but has trivial square. Enriques surfaces are all projective and are elliptic surfaces of genus 0. Over fields of characteristic not 2 they are quotients of K3 surfaces by a group of order 2 acting without fixed points and their theory is similar to that of algebraic K3 surfaces. Enriques surfaces were first studied in detail by Enriques as an answer to a question discussed by Castelnuovo (1895) about whether a surface with q = pg = 0 is necessarily rational, though some of the Reye congruences introduced earlier by Reye are also examples of Enriques surfaces.
In complex geometry, a Hopf surface is a compact complex surface obtained as a quotient of the complex vector space by a free action of a discrete group. If this group is the integers the Hopf surface is called primary, otherwise it is called secondary. The first example was found by Heinz Hopf, with the discrete group isomorphic to the integers, with a generator acting on by multiplication by 2; this was the first example of a compact complex surface with no Kähler metric.
In mathematics, a Raynaud surface is a particular kind of algebraic surface that was introduced in William E. Lang and named for Michel Raynaud. To be precise, a Raynaud surface is a quasi-elliptic surface over an algebraic curve of genus g greater than 1, such that all fibers are irreducible and the fibration has a section. The Kodaira vanishing theorem fails for such surfaces; in other words the Kodaira theorem, valid in algebraic geometry over the complex numbers, has such surfaces as counterexamples, and these can only exist in characteristic p.
In mathematics, the irregularity of a complex surface X is the Hodge number , usually denoted by q. The irregularity of an algebraic surface is sometimes defined to be this Hodge number, and sometimes defined to be the dimension of the Picard variety, which is the same in characteristic 0 but can be smaller in positive characteristic.
This is a glossary of algebraic geometry.
References
- Barth, Wolf P.; Hulek, Klaus; Peters, Chris A.M.; Van de Ven, Antonius (2004), Compact Complex Surfaces, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge., vol. 4, Springer-Verlag, Berlin, ISBN 978-3-540-00832-3, MR 2030225 - the standard reference book for compact complex surfaces
- Beauville, Arnaud (1996), Complex algebraic surfaces, London Mathematical Society Student Texts, vol. 34 (2nd ed.), Cambridge University Press, ISBN 978-0-521-49510-3, MR 1406314, ISBN 978-0-521-49842-5
- Bombieri, Enrico; Mumford, David (1976), "Enriques' classification of surfaces in char. p. III." (PDF), Inventiones Mathematicae , 35: 197–232, Bibcode:1976InMat..35..197B, doi:10.1007/BF01390138, ISSN 0020-9910, MR 0491720
- Bombieri, Enrico; Mumford, David (1977), "Enriques' classification of surfaces in char. p. II", Complex analysis and algebraic geometry, Tokyo: Iwanami Shoten, pp. 23–42, MR 0491719