
In mathematics, graph theory is the study of graphs, which are mathematical structures used to model pairwise relations between objects. A graph in this context is made up of vertices which are connected by edges. A distinction is made between undirected graphs, where edges link two vertices symmetrically, and directed graphs, where edges link two vertices asymmetrically; see Graph for more detailed definitions and for other variations in the types of graph that are commonly considered. Graphs are one of the prime objects of study in discrete mathematics.

Zeolites are microporous, aluminosilicate minerals commonly used as commercial adsorbents and catalysts. The term zeolite was originally coined in 1756 by Swedish mineralogist Axel Fredrik Cronstedt, who observed that rapidly heating the material, believed to have been stilbite, produced large amounts of steam from water that had been adsorbed by the material. Based on this, he called the material zeolite, from the Greek ζέω (zéō), meaning "to boil" and λίθος (líthos), meaning "stone". The classic reference for the field has been Breck's book Zeolite Molecular Sieves: Structure, Chemistry, And Use.
In a molecule, a stereocenter is a particular instance of a stereogenic element that is geometrically a point. A stereocenter or stereogenic center is any point in a molecule, though not necessarily an atom, bearing different substituents, such that interchanging any two substituents leads to a stereoisomer. The term stereocenter was introduced in 1984 by Kurt Mislow and Jay Siegel. A chirality center is a stereocenter consisting of an atom holding a set of ligands in a spatial arrangement which is not superimposable on its mirror image. The concept of a chirality center generalizes the concept of an asymmetric carbon atom such that an interchanging of any two groups gives rise to an enantiomer. In organic chemistry, a chirality center usually refers to a carbon, phosphorus, or sulfur atom, though it is also possible for other atoms to be chirality centers, especially in areas of organometallic and inorganic chemistry.

In the mathematical area of graph theory, a clique is a subset of vertices of an undirected graph such that every two distinct vertices in the clique are adjacent; that is, its induced subgraph is complete. Cliques are one of the basic concepts of graph theory and are used in many other mathematical problems and constructions on graphs. Cliques have also been studied in computer science: the task of finding whether there is a clique of a given size in a graph is NP-complete, but despite this hardness result, many algorithms for finding cliques have been studied.
In the mathematical discipline of graph theory, a matching or independent edge set in a graph is a set of edges without common vertices. Finding a matching in a bipartite graph can be treated as a network flow problem.

The diamond cubic crystal structure is a repeating pattern of 8 atoms that certain materials may adopt as they solidify. While the first known example was diamond, other elements in group 14 also adopt this structure, including α-tin, the semiconductors silicon and germanium, and silicon/germanium alloys in any proportion.
The term coordination geometry is used in a number of related fields of chemistry and solid state chemistry/physics.
In chemistry the polyhedral skeletal electron pair theory (PSEPT) provides electron counting rules useful for predicting the structures of clusters such as borane and carborane clusters. The electron counting rules were originally formulated by Kenneth Wade and were further developed by Michael Mingos and others; they are sometimes known as Wade's rules or the Wade–Mingos rules. The rules are based on a molecular orbital treatment of the bonding. These rules have been extended and unified in the form of the Jemmis mno rules.

In a tetrahedral molecular geometry, a central atom is located at the center with four substituents that are located at the corners of a tetrahedron. The bond angles are cos−1(−1⁄3) = 109.4712206...° ≈ 109.5° when all four substituents are the same, as in methane as well as its heavier analogues. Methane and other perfectly symmetrical tetrahedral molecules belong to point group Td, but most tetrahedral molecules have lower symmetry. Tetrahedral molecules can be chiral.

In graph theory, the Grundy number or Grundy chromatic number of an undirected graph is the maximum number of colors that can be used by a greedy coloring strategy that considers the vertices of the graph in sequence and assigns each vertex its first available color, using a vertex ordering chosen to use as many colors as possible. Grundy numbers are named after P. M. Grundy, who studied an analogous concept for directed graphs in 1939. The undirected version was introduced by Christen & Selkow (1979).

In geometry, a honeycomb is a space filling or close packing of polyhedral or higher-dimensional cells, so that there are no gaps. It is an example of the more general mathematical tiling or tessellation in any number of dimensions. Its dimension can be clarified as n-honeycomb for a honeycomb of n-dimensional space.

In graph theory, the Möbius ladderMn is a cubic circulant graph with an even number n of vertices, formed from an n-cycle by adding edges connecting opposite pairs of vertices in the cycle. It is so-named because Mn has exactly n/2 4-cycles which link together by their shared edges to form a topological Möbius strip. Möbius ladders were named and first studied by Guy and Harary (1967).
In topological graph theory, an embedding of a graph on a surface is a representation of on in which points of are associated with vertices and simple arcs are associated with edges in such a way that:

In graph theory, an area of mathematics, a claw-free graph is a graph that does not have a claw as an induced subgraph.
In chemical graph theory and in mathematical chemistry, a molecular graph or chemical graph is a representation of the structural formula of a chemical compound in terms of graph theory. A chemical graph is a labeled graph whose vertices correspond to the atoms of the compound and edges correspond to chemical bonds. Its vertices are labeled with the kinds of the corresponding atoms and edges are labeled with the types of bonds. For particular purposes any of the labelings may be ignored.
A combinatorial map is a combinatorial object modelling topological structures with subdivided objects. Historically, the concept was introduced informally by J. Edmonds for polyhedral surfaces which are planar graphs. It was given its first definite formal expression under the name "Constellations" by A. Jacques but the concept was already extensively used under the name "rotation" by Gerhard Ringel and J.W.T. Youngs in their famous solution of the Heawood map-coloring problem. The term "constellation" was not retained and instead "combinatorial map" was favored. The concept was later extended to represent higher-dimensional orientable subdivided objects. Combinatorial maps are used as efficient data structures in image representation and processing, in geometrical modeling. This model is related to simplicial complexes and to combinatorial topology. Note that combinatorial maps were extended to generalized maps that allow also to represent non-orientable objects like the Möbius strip and the Klein bottle. A combinatorial map is a boundary representation model; it represents object by its boundaries.

In crystallography, a periodic graph or crystal net is a three-dimensional periodic graph, i.e., a three-dimensional Euclidean graph whose vertices or nodes are points in three-dimensional Euclidean space, and whose edges are line segments connecting pairs of vertices, periodic in three linearly independent axial directions. There is usually an implicit assumption that the set of vertices are uniformly discrete, i.e., that there is a fixed minimum distance between any two vertices. The vertices may represent positions of atoms or complexes or clusters of atoms such as single-metal ions, molecular building blocks, or secondary building units, while each edge represents a chemical bond or a polymeric ligand.
A Euclidean graph is periodic if there exists a basis of that Euclidean space whose corresponding translations induce symmetries of that graph. Equivalently, a periodic Euclidean graph is a periodic realization of an abelian covering graph over a finite graph. A Euclidean graph is uniformly discrete if there is a minimal distance between any two vertices. Periodic graphs are closely related to tessellations of space and the geometry of their symmetry groups, hence to geometric group theory, as well as to discrete geometry and the theory of polytopes, and similar areas.
Igor Rivin is a Russian-Canadian mathematician, working in various fields of pure and applied mathematics, computer science, and materials science. He was the Regius Professor of Mathematics at the University of St. Andrews from 2015 to 2017, and is currently the chief research officer at Cryptos Fund.
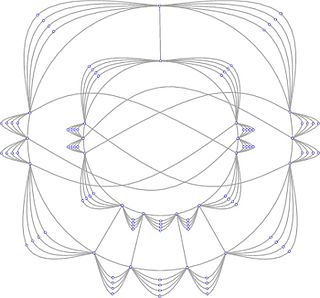
In mathematics, a topological graph is a representation of a graph in the plane, where the vertices of the graph are represented by distinct points and the edges by Jordan arcs joining the corresponding pairs of points. The points representing the vertices of a graph and the arcs representing its edges are called the vertices and the edges of the topological graph. It is usually assumed that any two edges of a topological graph cross a finite number of times, no edge passes through a vertex different from its endpoints, and no two edges touch each other (without crossing). A topological graph is also called a drawing of a graph.











