Related Research Articles
The mathematical formulations of quantum mechanics are those mathematical formalisms that permit a rigorous description of quantum mechanics. This mathematical formalism uses mainly a part of functional analysis,especially Hilbert spaces,which are a kind of linear space. Such are distinguished from mathematical formalisms for physics theories developed prior to the early 1900s by the use of abstract mathematical structures,such as infinite-dimensional Hilbert spaces,and operators on these spaces. In brief,values of physical observables such as energy and momentum were no longer considered as values of functions on phase space,but as eigenvalues;more precisely as spectral values of linear operators in Hilbert space.
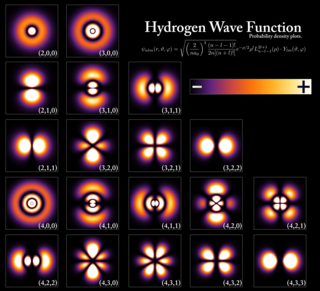
Quantum mechanics is a fundamental theory in physics that describes the behavior of nature at and below the scale of atoms. It is the foundation of all quantum physics,which includes quantum chemistry,quantum field theory,quantum technology,and quantum information science.
Analysis is the branch of mathematics dealing with continuous functions,limits,and related theories,such as differentiation,integration,measure,infinite sequences,series,and analytic functions.
The Schrödinger equation is a partial differential equation that governs the wave function of a quantum-mechanical system. Its discovery was a significant landmark in the development of quantum mechanics. It is named after Erwin Schrödinger,who postulated the equation in 1925 and published it in 1926,forming the basis for the work that resulted in his Nobel Prize in Physics in 1933.

Mathematical physics refers to the development of mathematical methods for application to problems in physics. The Journal of Mathematical Physics defines the field as "the application of mathematics to problems in physics and the development of mathematical methods suitable for such applications and for the formulation of physical theories". An alternative definition would also include those mathematics that are inspired by physics,known as physical mathematics.
In physics,an observable is a physical property or physical quantity that can be measured. In classical mechanics,an observable is a real-valued "function" on the set of all possible system states,e.g.,position and momentum. In quantum mechanics,an observable is an operator,or gauge,where the property of the quantum state can be determined by some sequence of operations. For example,these operations might involve submitting the system to various electromagnetic fields and eventually reading a value.
In science and especially in mathematical studies,a variational principle is one that enables a problem to be solved using calculus of variations,which concerns finding functions that optimize the values of quantities that depend on those functions. For example,the problem of determining the shape of a hanging chain suspended at both ends—a catenary—can be solved using variational calculus,and in this case,the variational principle is the following:The solution is a function that minimizes the gravitational potential energy of the chain.

The superposition principle,also known as superposition property,states that,for all linear systems,the net response caused by two or more stimuli is the sum of the responses that would have been caused by each stimulus individually. So that if input A produces response X,and input B produces response Y,then input (A + B) produces response (X + Y).

In mathematics,a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications,the functions generally represent physical quantities,the derivatives represent their rates of change,and the differential equation defines a relationship between the two. Such relations are common;therefore,differential equations play a prominent role in many disciplines including engineering,physics,economics,and biology.
In mathematics,generalized functions are objects extending the notion of functions. There is more than one recognized theory,for example the theory of distributions. Generalized functions are especially useful in making discontinuous functions more like smooth functions,and describing discrete physical phenomena such as point charges. They are applied extensively,especially in physics and engineering.

Franz Rellich was an Austrian-German mathematician. He made important contributions in mathematical physics,in particular for the foundations of quantum mechanics and for the theory of partial differential equations. The Rellich–Kondrachov theorem is named after him.

In mathematics,Hilbert spaces allow the methods of linear algebra and calculus to be generalized from (finite-dimensional) Euclidean vector spaces to spaces that may be infinite-dimensional. Hilbert spaces arise naturally and frequently in mathematics and physics,typically as function spaces. Formally,a Hilbert space is a vector space equipped with an inner product that induces a distance function for which the space is a complete metric space.
Burton Wendroff is an American applied mathematician known for his contributions to the development of numerical methods for the solution of hyperbolic partial differential equations. The Lax–Wendroff method for the solution of hyperbolic PDE is named for Wendroff.

Sergio Albeverio is a Swiss mathematician and mathematical physicist working in numerous fields of mathematics and its applications. In particular he is known for his work in probability theory,analysis,mathematical physics,and in the areas algebra,geometry,number theory,as well as in applications,from natural to social-economic sciences.
Israel Michael Sigal is a Canadian mathematician specializing in mathematical physics. He is a professor at the University of Toronto Department of Mathematics.
Robert C. Hermann was an American mathematician and mathematical physicist. In the 1960s Hermann worked on elementary particle physics and quantum field theory,and published books which revealed the interconnections between vector bundles on Riemannian manifolds and gauge theory in physics,before these interconnections became "common knowledge" among physicists in the 1970s.
The Koopman–von Neumann (KvN) theory is a description of classical mechanics as an operatorial theory similar to quantum mechanics,based on a Hilbert space of complex,square-integrable wavefunctions. As its name suggests,the KvN theory is loosely related to work by Bernard Koopman and John von Neumann in 1931 and 1932,respectively. As explained in this entry,however,the historical origins of the theory and its name are complicated.
The CEREMADE is a research centre in Mathematics within UniversitéParis-Dauphine. It was created in 1970.
Peter John Olver is a British-American mathematician working in differential geometry.
Lokenath Debnath was an Indian-American mathematician.
References
- ↑ "Curriculum vitae" (PDF). Retrieved June 12, 2024.
- ↑ "Karl Gustafson". Department of Mathematics. September 29, 2016. Retrieved April 30, 2022.
- ↑ Gustafson, Karl (2022). "Karl Gustafson, Department of Mathematics". University of Colorado, Boulder. Retrieved April 27, 2022.
- ↑ Gustafson, Karl (1968). "The angle of an operator and positive operator products". Bulletin of the American Mathematical Society. 74 (3): 488–492. doi: 10.1090/s0002-9904-1968-11974-3 . ISSN 0273-0979. S2CID 121445437.
- ↑ Gustafson, Karl (2012). Antieigenvalue analysis : with applications to numerical analysis, wavelets, statistics, quantum mechanics, finance and optimization. World Scientific Pub. Co. ISBN 978-981-4366-29-8. OCLC 921222071.
- ↑ E., Gustafson, Karl (1980). Partial differential equations and Hilbert space methods. Wiley. ISBN 0-471-04089-4. OCLC 310838854.
{{cite book}}: CS1 maint: multiple names: authors list (link) - ↑ Gustafson, Karl (1987). Introduction to Partial Differential Equations and Hilbert Space Methods, 2d edition. New York: Wiley. p. 438.
- ↑ Gustafson, Karl (1999). Partial Differential Equations and Hilbert Space Methods, 3d edition (revised). New York: Dover. p. 472. ISBN 0-486-61271-6. OCLC 246284657.
- ↑ Gustafson, Karl (1983). Introduction to Partial Differential Equations and Hilbert Space Methods. Taiwan: Yeh Yeh Publishers. p. 285.
- ↑ Gustafson, Karl (1991–1992). Applied Partial Differential Equations 1 and 2. Japan: Kaigai. pp. 247 & 235.
- ↑ Gustafson, Karl (1993). Introduction to Partial Differential Equations and Hilbert Space Methods, 3d edition. India: International Journal Services. p. 460.
- ↑ E., Gustafson, Karl (1997). Numerical range : the field of values of linear operators and matrices. Springer. ISBN 0-387-94835-X. OCLC 34912671.
{{cite book}}: CS1 maint: multiple names: authors list (link) - ↑ Gustafson, Karl (1981). Quantum Mechanics in Mathematics, Chemistry and Physics. New York: Springer. p. 506.
- ↑ Gustafson, Karl (1991). Vortex Methods and Vortex Motion. Philadelphia: SIAM. p. 227.
- ↑ Gustafson, Karl (1997). Lectures on Computational Fluid Dynamics, Mathematical Physics, and Linear Algebra. Japan: Kaigai Publishers. p. 169.
- ↑ Adams, Emily (February 1, 2022). "Honoree hopes new endowment will lead to quantum breakthrough". University of Colorado, Boulder, College of Engineering and Applied Science. Retrieved April 27, 2022.
- ↑ Gustafson, Karl (1952). "The Snowmass-Capitol Ridge". Trail and Timberline (Colorado Mountain Club). 404: 119–121.
- ↑ Ament, Pat; McCarty, Cleve (1995). High Over Boulder. Boulder, Colorado: Two Lights Publishing. pp. 81, 97.
- ↑ E., Gustafson, Karl (2012). The crossing of heaven : memoirs of a mathematician. Springer. ISBN 978-3-642-22558-1. OCLC 828889966.
{{cite book}}: CS1 maint: multiple names: authors list (link) - ↑ GUSTAFSON, KARL (2020). REVERBERATIONS OF A STROKE. SPRINGER. ISBN 978-3-030-12864-7. OCLC 1164825312.