Related Research Articles

Chaos theory is an interdisciplinary area of scientific study and branch of mathematics. It focuses on underlying patterns and deterministic laws of dynamical systems that are highly sensitive to initial conditions. These were once thought to have completely random states of disorder and irregularities. Chaos theory states that within the apparent randomness of chaotic complex systems, there are underlying patterns, interconnection, constant feedback loops, repetition, self-similarity, fractals and self-organization. The butterfly effect, an underlying principle of chaos, describes how a small change in one state of a deterministic nonlinear system can result in large differences in a later state. A metaphor for this behavior is that a butterfly flapping its wings in Brazil can cause a tornado in Texas.

In mathematics, a dynamical system is a system in which a function describes the time dependence of a point in an ambient space, such as in a parametric curve. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, the random motion of particles in the air, and the number of fish each springtime in a lake. The most general definition unifies several concepts in mathematics such as ordinary differential equations and ergodic theory by allowing different choices of the space and how time is measured. Time can be measured by integers, by real or complex numbers or can be a more general algebraic object, losing the memory of its physical origin, and the space may be a manifold or simply a set, without the need of a smooth space-time structure defined on it.

Stephen Smale is an American mathematician, known for his research in topology, dynamical systems and mathematical economics. He was awarded the Fields Medal in 1966 and spent more than three decades on the mathematics faculty of the University of California, Berkeley, where he currently is Professor Emeritus, with research interests in algorithms, numerical analysis and global analysis.

Ralph Herman Abraham is an American mathematician. Since 1968, he has been a member of the faculty of the University of California, Santa Cruz, where he is currently professor emeritus of mathematics.

Dynamical systems theory is an area of mathematics used to describe the behavior of complex dynamical systems, usually by employing differential equations or difference equations. When differential equations are employed, the theory is called continuous dynamical systems. From a physical point of view, continuous dynamical systems is a generalization of classical mechanics, a generalization where the equations of motion are postulated directly and are not constrained to be Euler–Lagrange equations of a least action principle. When difference equations are employed, the theory is called discrete dynamical systems. When the time variable runs over a set that is discrete over some intervals and continuous over other intervals or is any arbitrary time-set such as a Cantor set, one gets dynamic equations on time scales. Some situations may also be modeled by mixed operators, such as differential-difference equations.
In mathematics, symbolic dynamics is the practice of modeling a topological or smooth dynamical system by a discrete space consisting of infinite sequences of abstract symbols, each of which corresponds to a state of the system, with the dynamics (evolution) given by the shift operator. Formally, a Markov partition is used to provide a finite cover for the smooth system; each set of the cover is associated with a single symbol, and the sequences of symbols result as a trajectory of the system moves from one covering set to another.
In dynamical systems theory, a period-doubling bifurcation occurs when a slight change in a system's parameters causes a new periodic trajectory to emerge from an existing periodic trajectory—the new one having double the period of the original. With the doubled period, it takes twice as long for the numerical values visited by the system to repeat themselves.
Jump diffusion is a stochastic process that involves jumps and diffusion. It has important applications in magnetic reconnection, coronal mass ejections, condensed matter physics, option pricing, and pattern theory and computational vision.
John Barkley Rosser Jr. was a mathematical economist and Professor of Economics at James Madison University in Harrisonburg, Virginia since 1988. He was known for work in nonlinear economic dynamics, including applications in economics of catastrophe theory, chaos theory, and complexity theory. With Marina V. Rosser he invented the concept of the "new traditional economy". He introduced into economic discourse the concepts of chaotic bubbles, chaotic hysteresis, and econochemistry. He also invented the concepts of the megacorpstate and hypercyclic morphogenesis. He was the first to provide a mathematical model of the period of financial distress in a speculative bubble. With Marina V. Rosser and Ehsan Ahmed, he was the first to argue for a two-way positive link between income inequality and the size of an underground economy in a nation. Rosser's equation has been used to forecast ratios of future Social Security benefits to current ones in real terms.
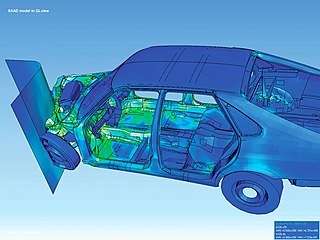
The finite element method (FEM) is a popular method for numerically solving differential equations arising in engineering and mathematical modeling. Typical problem areas of interest include the traditional fields of structural analysis, heat transfer, fluid flow, mass transport, and electromagnetic potential.

Howell Tong is a statistician who has made fundamental contributions to nonlinear time series analysis, semi-parametric statistics, non-parametric statistics, dimension reduction, model selection, likelihood-free statistics and other areas. In the words of Professor Peter Whittle (FRS): "The striking feature of Howell Tong's … is the continuing freshness, boldness and spirit of enquiry which inform them-indeed, proper qualities for an explorer. He stands as the recognised innovator and authority in his subject, while remaining disarmingly direct and enthusiastic." His work, in the words of Sir David Cox, "links two fascinating fields, nonlinear time series and deterministic dynamical systems." He is the father of the threshold time series models, which have extensive applications in ecology, economics, epidemiology and finance. Besides nonlinear time series analysis, he was the co-author of a seminal paper, which he read to the Royal Statistical Society, on dimension reduction in semi-parametric statistics by pioneering the approach based on minimum average variance estimation. He has also made numerous novel contributions to nonparametric statistics, Markov chain modelling, reliability, non-stationary time series analysis and wavelets.
Valentin Afraimovich was a Soviet, Russian and Mexican mathematician. He made contributions to dynamical systems theory, qualitative theory of ordinary differential equations, bifurcation theory, concept of attractor, strange attractors, space-time chaos, mathematical models of non-equilibrium media and biological systems, travelling waves in lattices, complexity of orbits and dimension-like characteristics in dynamical systems.

The Rulkov map is a two-dimensional iterated map used to model a biological neuron. It was proposed by Nikolai F. Rulkov in 2001. The use of this map to study neural networks has computational advantages because the map is easier to iterate than a continuous dynamical system. This saves memory and simplifies the computation of large neural networks.

Fair cake-cutting is a kind of fair division problem. The problem involves a heterogeneous resource, such as a cake with different toppings, that is assumed to be divisible – it is possible to cut arbitrarily small pieces of it without destroying their value. The resource has to be divided among several partners who have different preferences over different parts of the cake, i.e., some people prefer the chocolate toppings, some prefer the cherries, some just want as large a piece as possible. The division should be unanimously fair – each person should receive a piece believed to be a fair share.

Albert C.J. Luo is a mechanical engineering researcher who has been serving as a distinguished research professor at Southern Illinois University Edwardsville in Illinois since 1998.

In numerical mathematics, the gradient discretisation method (GDM) is a framework which contains classical and recent numerical schemes for diffusion problems of various kinds: linear or non-linear, steady-state or time-dependent. The schemes may be conforming or non-conforming, and may rely on very general polygonal or polyhedral meshes.

Soumitro Banerjee is an Indian electrical engineer and former director of the Indian Institute of Science Education and Research, Kolkata. He is known for his studies on bifurcation phenomena in power electronic circuits and is an elected fellow of all three major Indian science academies: the National Academy of Sciences, India, Indian Academy of Sciences, and Indian National Science Academy. He is also a fellow of The World Academy of Sciences, Institute of Electrical and Electronics Engineers, West Bengal Academy of Sciences and the Indian National Academy of Engineering. The Council of Scientific and Industrial Research, the apex agency of the Government of India for scientific research, awarded him the Shanti Swarup Bhatnagar Prize for Science and Technology, one of the highest Indian science awards for his contributions to Engineering Sciences in 2003.
Yuliya Stepanivna Mishura is a Ukrainian mathematician specializing in probability theory and mathematical finance. She is a professor at the Taras Shevchenko National University of Kyiv.
Iryna Sushko is a Ukrainian mathematician who works as a senior research fellow in the Institute of Mathematics of the National Academy of Sciences of Ukraine and as a visiting professor at the Kyiv School of Economics. Her research concerns nonlinear dynamical systems and their applications in economics and radio engineering.
References
- 1 2 "Laura Gardini", Who is who, Research group MDEF Modelli dinamici in economia e finanza (Dynamic models in Economics and Finance), retrieved 2023-01-20
- ↑ Bischi, Gian Italo; Chiarella, Carl; Sushko, Iryna (2013), "Introduction", in Bischi, Gian Italo; Chiarella, Carl; Sushko, Iryna (eds.), Global Analysis of Dynamic Models in Economics and Finance, Springer Berlin Heidelberg, pp. ix–xvi, doi:10.1007/978-3-642-29503-4, ISBN 978-3-642-29502-7
- ↑ "Editorial board", Mathematics and Computers in Simulation, Elsevier, retrieved 2023-01-20
- 1 2 Horníková, Adriana (February 2014), "Review of Global Analysis of Dynamic Models in Economics and Finance: Essays in Honour of Laura Gardini", Technometrics, 56 (1): 125–126, JSTOR 24587285
- ↑ Reviews of Chaos in Discrete Dynamical Systems: Robert L. Devaney, SIAM Review, JSTOR 2653051; E.Ershov, Zbl 0883.58019; Frederick R. Marotto, MR 1451369; James Sochacki, Discrete Dynamics in Nature and Society,
- ↑ Reviews of Continuous and Discontinuous Piecewise-Smooth One-Dimensional Maps: Adriana Buică, Zbl 1452.37002; Michał Misiurewicz, SIAM Review, doi:10.1137/20N975038; Davide Radi, Journal of Difference Equations and Applications, doi:10.1080/10236198.2021.1971210