Neural gas

Last updated January 12, 2025

Neural gas is an artificial neural network, inspired by the self-organizing map and introduced in 1991 by Thomas Martinetz and Klaus Schulten.^[1] The neural gas is a simple algorithm for finding optimal data representations based on feature vectors. The algorithm was coined "neural gas" because of the dynamics of the feature vectors during the adaptation process, which distribute themselves like a gas within the data space. It is applied where data compression or vector quantization is an issue, for example speech recognition,^[2] image processing ^[3] or pattern recognition. As a robustly converging alternative to the k-means clustering it is also used for cluster analysis.^[4]

Algorithm

Suppose we want to model a probability distribution $P(x)$ of data vectors $x$ using a finite number of feature vectors $w_{i}$ , where $i=1,\cdots ,N$ .

For each time step $t$ $Neural gas$
1. Sample data vector $x$ from $P(x)$
2. Compute the distance between $x$ and each feature vector. Rank the distances.
3. Let $i_{0}$ be the index of the closest feature vector, $i_{1}$ the index of the second closest feature vector, and so on.
4. Update each feature vector by: $w_{i_{k}}^{t+1}=w_{i_{k}}^{t}+\varepsilon \cdot e^{-k/\lambda }\cdot (x-w_{i_{k}}^{t}),k=0,\cdots ,N-1$

In the algorithm, $\varepsilon$ can be understood as the learning rate, and $\lambda$ as the neighborhood range. $\varepsilon$ and $\lambda$ are reduced with increasing $t$ so that the algorithm converges after many adaptation steps.

The adaptation step of the neural gas can be interpreted as gradient descent on a cost function. By adapting not only the closest feature vector but all of them with a step size decreasing with increasing distance order, compared to (online) k-means clustering a much more robust convergence of the algorithm can be achieved. The neural gas model does not delete a node and also does not create new nodes.

Comparison with SOM

Compared to self-organized map, the neural gas model does not assume that some vectors are neighbors. If two vectors happen to be close together, they would tend to move together, and if two vectors happen to be apart, they would tend to not move together. In contrast, in an SOM, if two vectors are neighbors in the underlying graph, then they will always tend to move together, no matter whether the two vectors happen to be neighbors in the Euclidean space.

The name "neural gas" is because one can imagine it to be what an SOM would be like if there is no underlying graph, and all points are free to move without the bonds that bind them together.

Variants

A number of variants of the neural gas algorithm exists in the literature so as to mitigate some of its shortcomings. More notable is perhaps Bernd Fritzke's growing neural gas,^[5] but also one should mention further elaborations such as the Growing When Required network^[6] and also the incremental growing neural gas.^[7] A performance-oriented approach that avoids the risk of overfitting is the Plastic Neural gas model.^[8]

Growing neural gas

Fritzke describes the growing neural gas (GNG) as an incremental network model that learns topological relations by using a "Hebb-like learning rule",^[5] only, unlike the neural gas, it has no parameters that change over time and it is capable of continuous learning, i.e. learning on data streams. GNG has been widely used in several domains,^[9] demonstrating its capabilities for clustering data incrementally. The GNG is initialized with two randomly positioned nodes which are initially connected with a zero age edge and whose errors are set to 0. Since in the GNG input data is presented sequentially one by one, the following steps are followed at each iteration:

It is calculating the errors (distances) between the two closest nodes to the current input data.
The error of the winner node (only the closest one) is respectively accumulated.
The winner node and its topological neighbors (connected by an edge) are moving towards the current input by different fractions of their respective errors.
The age of all edges connected to the winner node are incremented.
If the winner node and the second-winner are connected by an edge, such an edge is set to 0. Else, an edge is created between them.
If there are edges with an age larger than a threshold, they are removed. Nodes without connections are eliminated.
If the current iteration is an integer multiple of a predefined frequency-creation threshold, a new node is inserted between the node with the largest error (among all) and its topological neighbor presenting the highest error. The link between the former and the latter nodes is eliminated (their errors are decreased by a given factor) and the new node is connected to both of them. The error of the new node is initialized as the updated error of the node which had the largest error (among all).
The accumulated error of all nodes is decreased by a given factor.
If the stopping criterion is not met, the algorithm takes a following input. The criterion might be a given number of epochs, i.e., a pre-set number of times where all data is presented, or the reach of a maximum number of nodes.

Incremental growing neural gas

Another neural gas variant inspired by the GNG algorithm is the incremental growing neural gas (IGNG). The authors propose the main advantage of this algorithm to be "learning new data (plasticity) without degrading the previously trained network and forgetting the old input data (stability)."^[7]

Growing when required

Having a network with a growing set of nodes, like the one implemented by the GNG algorithm was seen as a great advantage, however some limitation on the learning was seen by the introduction of the parameter λ, in which the network would only be able to grow when iterations were a multiple of this parameter.^[6] The proposal to mitigate this problem was a new algorithm, the Growing When Required network (GWR), which would have the network grow more quickly, by adding nodes as quickly as possible whenever the network identified that the existing nodes would not describe the input well enough.

Plastic neural gas

The ability to only grow a network may quickly introduce overfitting; on the other hand, removing nodes on the basis of age only, as in the GNG model, does not ensure that the removed nodes are actually useless, because removal depends on a model parameter that should be carefully tuned to the "memory length" of the stream of input data.

The "Plastic Neural Gas" model^[8] solves this problem by making decisions to add or remove nodes using an unsupervised version of cross-validation, which controls an equivalent notion of "generalization ability" for the unsupervised setting.

While growing-only methods only cater for the incremental learning scenario, the ability to grow and shrink is suited to the more general streaming data problem.

Implementations

To find the ranking $i_{0},i_{1},\ldots ,i_{N-1}$ of the feature vectors, the neural gas algorithm involves sorting, which is a procedure that does not lend itself easily to parallelization or implementation in analog hardware. However, implementations in both parallel software ^[10] and analog hardware^[11] were actually designed.

Related Research Articles

In machine learning, supervised learning (SL) is a paradigm where a model is trained using input objects and desired output values, which are often human-made labels. The training process builds a function that maps new data to expected output values. An optimal scenario will allow for the algorithm to accurately determine output values for unseen instances. This requires the learning algorithm to generalize from the training data to unseen situations in a "reasonable" way. This statistical quality of an algorithm is measured via a generalization error.

Vector quantization (VQ) is a classical quantization technique from signal processing that allows the modeling of probability density functions by the distribution of prototype vectors. Developed in the early 1980s by Robert M. Gray, it was originally used for data compression. It works by dividing a large set of points (vectors) into groups having approximately the same number of points closest to them. Each group is represented by its centroid point, as in k-means and some other clustering algorithms. In simpler terms, vector quantization chooses a set of points to represent a larger set of points.

A self-organizing map (SOM) or self-organizing feature map (SOFM) is an unsupervised machine learning technique used to produce a low-dimensional representation of a higher-dimensional data set while preserving the topological structure of the data. For example, a data set with $variables measured in observations could be represented as clusters of observations with similar values for the variables. These clusters then could be visualized as a two-dimensional "map" such that observations in proximal clusters have more similar values than observations in distal clusters. This can make high-dimensional data easier to visualize and analyze.$

In machine learning, the perceptron is an algorithm for supervised learning of binary classifiers. A binary classifier is a function which can decide whether or not an input, represented by a vector of numbers, belongs to some specific class. It is a type of linear classifier, i.e. a classification algorithm that makes its predictions based on a linear predictor function combining a set of weights with the feature vector.

Unsupervised learning is a framework in machine learning where, in contrast to supervised learning, algorithms learn patterns exclusively from unlabeled data. Other frameworks in the spectrum of supervisions include weak- or semi-supervision, where a small portion of the data is tagged, and self-supervision. Some researchers consider self-supervised learning a form of unsupervised learning.

In computer science, learning vector quantization (LVQ) is a prototype-based supervised classification algorithm. LVQ is the supervised counterpart of vector quantization systems.

In machine learning, backpropagation is a gradient estimation method commonly used for training a neural network to compute its parameter updates.

Recurrent neural networks (RNNs) are a class of artificial neural network commonly used for sequential data processing. Unlike feedforward neural networks, which process data in a single pass, RNNs process data across multiple time steps, making them well-adapted for modelling and processing text, speech, and time series.

Feedforward refers to recognition-inference architecture of neural networks. Artificial neural network architectures are based on inputs multiplied by weights to obtain outputs (inputs-to-output): feedforward. Recurrent neural networks, or neural networks with loops allow information from later processing stages to feed back to earlier stages for sequence processing. However, at every stage of inference a feedforward multiplication remains the core, essential for backpropagation or backpropagation through time. Thus neural networks cannot contain feedback like negative feedback or positive feedback where the outputs feed back to the very same inputs and modify them, because this forms an infinite loop which is not possible to rewind in time to generate an error signal through backpropagation. This issue and nomenclature appear to be a point of confusion between some computer scientists and scientists in other fields studying brain networks.

In deep learning, a multilayer perceptron (MLP) is a name for a modern feedforward neural network consisting of fully connected neurons with nonlinear activation functions, organized in layers, notable for being able to distinguish data that is not linearly separable.

An autoencoder is a type of artificial neural network used to learn efficient codings of unlabeled data. An autoencoder learns two functions: an encoding function that transforms the input data, and a decoding function that recreates the input data from the encoded representation. The autoencoder learns an efficient representation (encoding) for a set of data, typically for dimensionality reduction, to generate lower-dimensional embeddings for subsequent use by other machine learning algorithms.

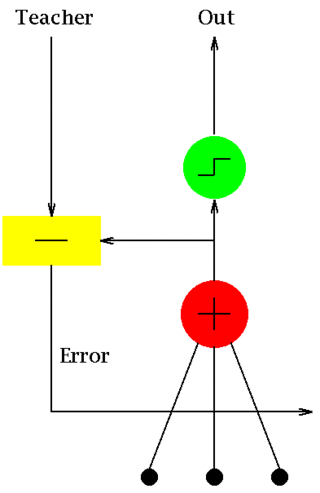
ADALINE is an early single-layer artificial neural network and the name of the physical device that implemented it. It was developed by professor Bernard Widrow and his doctoral student Marcian Hoff at Stanford University in 1960. It is based on the perceptron and consists of weights, a bias, and a summation function. The weights and biases were implemented by rheostats, and later, memistors.

The generalized Hebbian algorithm, also known in the literature as Sanger's rule, is a linear feedforward neural network for unsupervised learning with applications primarily in principal components analysis. First defined in 1989, it is similar to Oja's rule in its formulation and stability, except it can be applied to networks with multiple outputs. The name originates because of the similarity between the algorithm and a hypothesis made by Donald Hebb about the way in which synaptic strengths in the brain are modified in response to experience, i.e., that changes are proportional to the correlation between the firing of pre- and post-synaptic neurons.

In computer science, online machine learning is a method of machine learning in which data becomes available in a sequential order and is used to update the best predictor for future data at each step, as opposed to batch learning techniques which generate the best predictor by learning on the entire training data set at once. Online learning is a common technique used in areas of machine learning where it is computationally infeasible to train over the entire dataset, requiring the need of out-of-core algorithms. It is also used in situations where it is necessary for the algorithm to dynamically adapt to new patterns in the data, or when the data itself is generated as a function of time, e.g., prediction of prices in the financial international markets. Online learning algorithms may be prone to catastrophic interference, a problem that can be addressed by incremental learning approaches.

Competitive learning is a form of unsupervised learning in artificial neural networks, in which nodes compete for the right to respond to a subset of the input data. A variant of Hebbian learning, competitive learning works by increasing the specialization of each node in the network. It is well suited to finding clusters within data.

Extension neural network is a pattern recognition method found by M. H. Wang and C. P. Hung in 2003 to classify instances of data sets. Extension neural network is composed of artificial neural network and extension theory concepts. It uses the fast and adaptive learning capability of neural network and correlation estimation property of extension theory by calculating extension distance.
ENN was used in:

In machine learning (ML), feature learning or representation learning is a set of techniques that allow a system to automatically discover the representations needed for feature detection or classification from raw data. This replaces manual feature engineering and allows a machine to both learn the features and use them to perform a specific task.

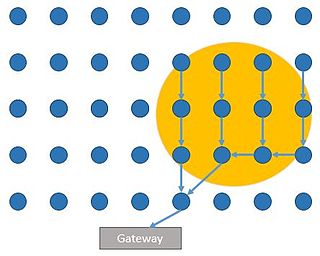
Wireless sensor networks (WSN) are a spatially distributed network of autonomous sensors used for monitoring an environment. Energy cost is a major limitation for WSN requiring the need for energy efficient networks and processing. One of major energy costs in WSN is the energy spent on communication between nodes and it is sometimes desirable to only send data to a gateway node when an event of interest is triggered at a sensor. Sensors will then only open communication during a probable event, saving on communication costs. Fields interested in this type of network include surveillance, home automation, disaster relief, traffic control, health care and more.

Fusion adaptive resonance theory (fusion ART) is a generalization of self-organizing neural networks known as the original Adaptive Resonance Theory models for learning recognition categories across multiple pattern channels. There is a separate stream of work on fusion ARTMAP, that extends fuzzy ARTMAP consisting of two fuzzy ART modules connected by an inter-ART map field to an extended architecture consisting of multiple ART modules.

Graph neural networks (GNN) are specialized artificial neural networks that are designed for tasks whose inputs are graphs.

References

↑ Thomas Martinetz and Klaus Schulten (1991). "A "neural gas" network learns topologies" (PDF). Artificial Neural Networks. Elsevier. pp. 397–402.
↑ F. Curatelli; O. Mayora-Iberra (2000). "Competitive learning methods for efficient Vector Quantizations in a speech recognition environment". In Osvaldo Cairó; L. Enrique Sucar; Francisco J. Cantú-Ortiz (eds.). MICAI 2000: Advances in artificial intelligence : Mexican International Conference on Artificial Intelligence, Acapulco, Mexico, April 2000 : proceedings . Springer. p. 109. ISBN 978-3-540-67354-5.
↑ Angelopoulou, Anastassia; Psarrou, Alexandra; Garcia Rodriguez, Jose; Revett, Kenneth (2005). "Computer Vision for Biomedical Image Applications". In Yanxi Liu; Tianzi Jiang; Changshui Zhang (eds.). Computer vision for biomedical image applications: first international workshop, CVBIA 2005, Beijing, China, October 21, 2005 : proceedings. Lecture Notes in Computer Science. Vol. 3765. Springer. p. 210. doi:10.1007/11569541_22. ISBN 978-3-540-29411-5.
↑ Fernando Canales; Max Chacon (2007). "Progress in Pattern Recognition, Image Analysis and Applications". In Luis Rueda; Domingo Mery (eds.). Progress in pattern recognition, image analysis and applications: 12th Iberoamerican Congress on Pattern Recognition, CIARP 2007, Viña del Mar-Valparaiso, Chile, November 13–16, 2007; proceedings. Lecture Notes in Computer Science. Vol. 4756. Springer. pp. 684–693. doi: 10.1007/978-3-540-76725-1_71 . ISBN 978-3-540-76724-4.
1 2 Fritzke, Bernd (1995). "A Growing Neural Gas Network Learns Topologies". Advances in Neural Information Processing Systems. 7: 625–632. Retrieved 2016-04-26.
1 2 Marsland, Stephen; Shapiro, Jonathan; Nehmzow, Ulrich (2002). "A self-organising network that grows when required". Neural Networks. 15 (8): 1041–1058. CiteSeerX 10.1.1.14.8763 . doi:10.1016/s0893-6080(02)00078-3. PMID 12416693.
1 2 Prudent, Yann; Ennaji, Abdellatif (2005). "An incremental growing neural gas learns topologies". Proceedings. 2005 IEEE International Joint Conference on Neural Networks, 2005. Vol. 2. pp. 1211–1216. doi:10.1109/IJCNN.2005.1556026. ISBN 978-0-7803-9048-5. S2CID 41517545.
1 2 Ridella, Sandro; Rovetta, Stefano; Zunino, Rodolfo (1998). "Plastic algorithm for adaptive vector quantisation". Neural Computing & Applications. 7: 37–51. doi:10.1007/BF01413708. S2CID 1184174.
↑ Iqbal, Hafsa; Campo, Damian; Baydoun, Mohamad; Marcenaro, Lucio; Martin, David; Regazzoni, Carlo (2019). "Clustering Optimization for Abnormality Detection in Semi-Autonomous Systems". 1st International Workshop on Multimodal Understanding and Learning for Embodied Applications. pp. 33–41. doi: 10.1145/3347450.3357657 . ISBN 978-1-4503-6918-3.
↑ Ancona, Fabio; Rovetta, Stefano; Zunino, Rodolfo (1996). "A parallel approach to plastic neural gas". Proceedings of International Conference on Neural Networks (ICNN'96). Vol. 1. pp. 126–130. doi:10.1109/ICNN.1996.548878. ISBN 0-7803-3210-5. S2CID 61686854.
↑ Ancona, Fabio; Rovetta, Stefano; Zunino, Rodolfo (1997). "Hardware implementation of the neural gas". Proceedings of International Conference on Neural Networks (ICNN'97). Vol. 2. pp. 991–994. doi:10.1109/ICNN.1997.616161. ISBN 0-7803-4122-8. S2CID 62480597.

External links

DemoGNG.js Javascript simulator for Neural Gas (and other network models)
Java Competitive Learning Applications Unsupervised Neural Networks (including Self-organizing map) in Java with source codes.
formal description of Neural gas algorithm
A GNG and GWR Classifier implementation in Matlab

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Thomas Martinetz and Klaus Schulten (1991). "A "neural gas" network learns topologies" (PDF). Artificial Neural Networks. Elsevier. pp. 397–402.

[2] F. Curatelli; O. Mayora-Iberra (2000). "Competitive learning methods for efficient Vector Quantizations in a speech recognition environment". In Osvaldo Cairó; L. Enrique Sucar; Francisco J. Cantú-Ortiz (eds.). MICAI 2000: Advances in artificial intelligence : Mexican International Conference on Artificial Intelligence, Acapulco, Mexico, April 2000 : proceedings . Springer. p. 109. ISBN 978-3-540-67354-5.

[3] Angelopoulou, Anastassia; Psarrou, Alexandra; Garcia Rodriguez, Jose; Revett, Kenneth (2005). "Computer Vision for Biomedical Image Applications". In Yanxi Liu; Tianzi Jiang; Changshui Zhang (eds.). Computer vision for biomedical image applications: first international workshop, CVBIA 2005, Beijing, China, October 21, 2005 : proceedings. Lecture Notes in Computer Science. Vol. 3765. Springer. p. 210. doi:10.1007/11569541_22. ISBN 978-3-540-29411-5.

[4] Fernando Canales; Max Chacon (2007). "Progress in Pattern Recognition, Image Analysis and Applications". In Luis Rueda; Domingo Mery (eds.). Progress in pattern recognition, image analysis and applications: 12th Iberoamerican Congress on Pattern Recognition, CIARP 2007, Viña del Mar-Valparaiso, Chile, November 13–16, 2007; proceedings. Lecture Notes in Computer Science. Vol. 4756. Springer. pp. 684–693. doi: 10.1007/978-3-540-76725-1_71 . ISBN 978-3-540-76724-4.

[:0-5] 1 2 Fritzke, Bernd (1995). "A Growing Neural Gas Network Learns Topologies". Advances in Neural Information Processing Systems. 7: 625–632. Retrieved 2016-04-26.

[:1-6] 1 2 Marsland, Stephen; Shapiro, Jonathan; Nehmzow, Ulrich (2002). "A self-organising network that grows when required". Neural Networks. 15 (8): 1041–1058. CiteSeerX 10.1.1.14.8763 . doi:10.1016/s0893-6080(02)00078-3. PMID 12416693.

[:2-7] 1 2 Prudent, Yann; Ennaji, Abdellatif (2005). "An incremental growing neural gas learns topologies". Proceedings. 2005 IEEE International Joint Conference on Neural Networks, 2005. Vol. 2. pp. 1211–1216. doi:10.1109/IJCNN.2005.1556026. ISBN 978-0-7803-9048-5. S2CID 41517545.

[pgas-8] 1 2 Ridella, Sandro; Rovetta, Stefano; Zunino, Rodolfo (1998). "Plastic algorithm for adaptive vector quantisation". Neural Computing & Applications. 7: 37–51. doi:10.1007/BF01413708. S2CID 1184174.

[9] Iqbal, Hafsa; Campo, Damian; Baydoun, Mohamad; Marcenaro, Lucio; Martin, David; Regazzoni, Carlo (2019). "Clustering Optimization for Abnormality Detection in Semi-Autonomous Systems". 1st International Workshop on Multimodal Understanding and Learning for Embodied Applications. pp. 33–41. doi: 10.1145/3347450.3357657 . ISBN 978-1-4503-6918-3.

[10] Ancona, Fabio; Rovetta, Stefano; Zunino, Rodolfo (1996). "A parallel approach to plastic neural gas". Proceedings of International Conference on Neural Networks (ICNN'96). Vol. 1. pp. 126–130. doi:10.1109/ICNN.1996.548878. ISBN 0-7803-3210-5. S2CID 61686854.

[11] Ancona, Fabio; Rovetta, Stefano; Zunino, Rodolfo (1997). "Hardware implementation of the neural gas". Proceedings of International Conference on Neural Networks (ICNN'97). Vol. 2. pp. 991–994. doi:10.1109/ICNN.1997.616161. ISBN 0-7803-4122-8. S2CID 62480597.

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]