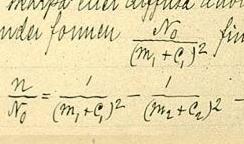Conversion of units is the conversion of the unit of measurement in which a quantity is expressed, typically through a multiplicative conversion factor that changes the unit without changing the quantity. This is also often loosely taken to include replacement of a quantity with a corresponding quantity that describes the same physical property.
In mathematics, the factorial of a non-negative integer , denoted by , is the product of all positive integers less than or equal to . The factorial of also equals the product of with the next smaller factorial: For example, The value of 0! is 1, according to the convention for an empty product.

In mathematics, the gamma function is the most common extension of the factorial function to complex numbers. Derived by Daniel Bernoulli, the gamma function is defined for all complex numbers except non-positive integers, and for every positive integer , The gamma function can be defined via a convergent improper integral for complex numbers with positive real part:
In physical chemistry, the Arrhenius equation is a formula for the temperature dependence of reaction rates. The equation was proposed by Svante Arrhenius in 1889, based on the work of Dutch chemist Jacobus Henricus van 't Hoff who had noted in 1884 that the van 't Hoff equation for the temperature dependence of equilibrium constants suggests such a formula for the rates of both forward and reverse reactions. This equation has a vast and important application in determining the rate of chemical reactions and for calculation of energy of activation. Arrhenius provided a physical justification and interpretation for the formula. Currently, it is best seen as an empirical relationship. It can be used to model the temperature variation of diffusion coefficients, population of crystal vacancies, creep rates, and many other thermally induced processes and reactions. The Eyring equation, developed in 1935, also expresses the relationship between rate and energy.

In mathematics, Stirling's approximation is an asymptotic approximation for factorials. It is a good approximation, leading to accurate results even for small values of . It is named after James Stirling, though a related but less precise result was first stated by Abraham de Moivre.

A solenoid is a type of electromagnet formed by a helical coil of wire whose length is substantially greater than its diameter, which generates a controlled magnetic field. The coil can produce a uniform magnetic field in a volume of space when an electric current is passed through it.
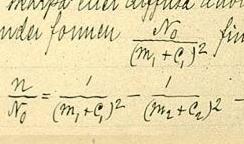
In atomic physics, the Rydberg formula calculates the wavelengths of a spectral line in many chemical elements. The formula was primarily presented as a generalization of the Balmer series for all atomic electron transitions of hydrogen. It was first empirically stated in 1888 by the Swedish physicist Johannes Rydberg, then theoretically by Niels Bohr in 1913, who used a primitive form of quantum mechanics. The formula directly generalizes the equations used to calculate the wavelengths of the hydrogen spectral series.
Runs created (RC) is a baseball statistic invented by Bill James to estimate the number of runs a hitter contributes to their team.
Pythagorean expectation is a sports analytics formula devised by Bill James to estimate the percentage of games a baseball team "should" have won based on the number of runs they scored and allowed. Comparing a team's actual and Pythagorean winning percentage can be used to make predictions and evaluate which teams are over-performing and under-performing. The name comes from the formula's resemblance to the Pythagorean theorem.
In classical statistical mechanics, the equipartition theorem relates the temperature of a system to its average energies. The equipartition theorem is also known as the law of equipartition, equipartition of energy, or simply equipartition. The original idea of equipartition was that, in thermal equilibrium, energy is shared equally among all of its various forms; for example, the average kinetic energy per degree of freedom in translational motion of a molecule should equal that in rotational motion.
The Graham number or Benjamin Graham number is a figure used in securities investing that measures a stock's so-called fair value. Named after Benjamin Graham, the founder of value investing, the Graham number can be calculated as follows:
In corporate finance, distressed securities are securities over companies or government entities that are experiencing financial or operational distress, default, or are under bankruptcy. As far as debt securities, this is called distressed debt. Purchasing or holding such distressed-debt creates significant risk due to the possibility that bankruptcy may render such securities worthless.

Linear discriminant analysis (LDA), normal discriminant analysis (NDA), or discriminant function analysis is a generalization of Fisher's linear discriminant, a method used in statistics and other fields, to find a linear combination of features that characterizes or separates two or more classes of objects or events. The resulting combination may be used as a linear classifier, or, more commonly, for dimensionality reduction before later classification.
Financial distress is a term in corporate finance used to indicate a condition when promises to creditors of a company are broken or honored with difficulty. If financial distress cannot be relieved, it can lead to bankruptcy. Financial distress is usually associated with some costs to the company; these are known as costs of financial distress.
Edward I. Altman is a Professor of Finance, Emeritus, at New York University's Stern School of Business. He is best known for the development of the Altman Z-score for predicting bankruptcy which he published in 1968. Professor Altman is a leading academic on the High-Yield and Distressed Debt markets and is the pioneer in the building of models for credit risk management and bankruptcy prediction.

The Z-score formula for predicting bankruptcy was published in 1968 by Edward I. Altman, who was, at the time, an Assistant Professor of Finance at New York University. The formula may be used to determine the probability that a firm will go into bankruptcy within two years. Z-scores are used to predict corporate defaults and an easy-to-calculate control measure for the financial distress status of companies in academic studies. The Z-score uses multiple corporate income and balance sheet values to measure the financial health of a company.
Bankruptcy prediction is the art of predicting bankruptcy and various measures of financial distress of public firms. It is a vast area of finance and accounting research. The importance of the area is due in part to the relevance for creditors and investors in evaluating the likelihood that a firm may go bankrupt.
A period of financial distress occurs when the price of a company or an asset or an index of a set of assets in a market is declining with the danger of a sudden crash of value occurring, either because the company is experiencing increasing problems of cash flow or a deteriorating credit balance or because the price had become too high as a result of a speculative bubble that has now peaked.
The Beneish model is a statistical model that uses financial ratios calculated with accounting data of a specific company in order to check if it is likely that the reported earnings of the company have been manipulated.
Piotroski F-score is a number between 0 and 9 which is used to assess strength of company's financial position. The score is used by financial investors in order to find the best value stocks. The score is named after Stanford accounting professor Joseph Piotroski.