Related Research Articles
Charles Babbage was an English polymath. A mathematician, philosopher, inventor and mechanical engineer, Babbage originated the concept of a digital programmable computer.
Differential geometry is a mathematical discipline that studies the geometry of smooth shapes and smooth spaces, otherwise known as smooth manifolds. It uses the techniques of differential calculus, integral calculus, linear algebra and multilinear algebra. The field has its origins in the study of spherical geometry as far back as antiquity. It also relates to astronomy, the geodesy of the Earth, and later the study of hyperbolic geometry by Lobachevsky. The simplest examples of smooth spaces are the plane and space curves and surfaces in the three-dimensional Euclidean space, and the study of these shapes formed the basis for development of modern differential geometry during the 18th and 19th centuries.
The Large Scale Structure of Space–Time is a 1973 treatise on the theoretical physics of spacetime by the physicist Stephen Hawking and the mathematician George Ellis. It is intended for specialists in general relativity rather than newcomers.
In mathematics, a submersion is a differentiable map between differentiable manifolds whose differential is everywhere surjective. This is a basic concept in differential topology. The notion of a submersion is dual to the notion of an immersion.
Sir William Vallance Douglas Hodge was a British mathematician, specifically a geometer.
David Bryant Mumford is an American mathematician known for his work in algebraic geometry and then for research into vision and pattern theory. He won the Fields Medal and was a MacArthur Fellow. In 2010 he was awarded the National Medal of Science. He is currently a University Professor Emeritus in the Division of Applied Mathematics at Brown University.
In mathematics, two functions have a contact of order k if, at a point P, they have the same value and k equal derivatives. This is an equivalence relation, whose equivalence classes are generally called jets. The point of osculation is also called the double cusp. Contact is a geometric notion; it can be defined algebraically as a valuation.
In mathematics, a cubic surface is a surface in 3-dimensional space defined by one polynomial equation of degree 3. Cubic surfaces are fundamental examples in algebraic geometry. The theory is simplified by working in projective space rather than affine space, and so cubic surfaces are generally considered in projective 3-space . The theory also becomes more uniform by focusing on surfaces over the complex numbers rather than the real numbers; note that a complex surface has real dimension 4. A simple example is the Fermat cubic surface
George Francis Rayner Ellis, FRS, Hon. FRSSAf, is the emeritus distinguished professor of complex systems in the Department of Mathematics and Applied Mathematics at the University of Cape Town in South Africa. He co-authored The Large Scale Structure of Space-Time with University of Cambridge physicist Stephen Hawking, published in 1973, and is considered one of the world's leading theorists in cosmology. From 1989 to 1992 he served as president of the International Society on General Relativity and Gravitation. He is a past president of the International Society for Science and Religion. He is an A-rated researcher with the NRF.
In mathematics, Belyi's theorem on algebraic curves states that any non-singular algebraic curve C, defined by algebraic number coefficients, represents a compact Riemann surface which is a ramified covering of the Riemann sphere, ramified at three points only.
Richard E. Blahut, former chair of the Electrical and Computer Engineering Department at the University of Illinois at Urbana–Champaign, is best known for his work in information theory. He received his PhD Electrical Engineering from Cornell University in 1972.
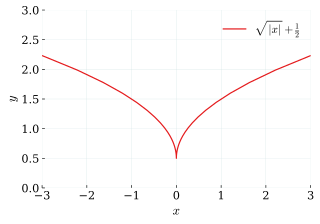
In mathematics, a cusp, sometimes called spinode in old texts, is a point on a curve where a moving point must reverse direction. A typical example is given in the figure. A cusp is thus a type of singular point of a curve.
In mathematics, an immersion is a differentiable function between differentiable manifolds whose differential pushforward is everywhere injective. Explicitly, f : M → N is an immersion if
Peter John Hilton was a British mathematician, noted for his contributions to homotopy theory and for code-breaking during World War II.
In mathematics, geometric measure theory (GMT) is the study of geometric properties of sets through measure theory. It allows mathematicians to extend tools from differential geometry to a much larger class of surfaces that are not necessarily smooth.
Michael J. T. Guy is a British computer scientist and mathematician. He is known for early work on computer systems, such as the Phoenix system at the University of Cambridge, and for contributions to number theory, computer algebra, and the theory of polyhedra in higher dimensions. He worked closely with John Horton Conway, and is the son of Conway's collaborator Richard K. Guy.
Ian Robertson Porteous was a Scottish mathematician at the University of Liverpool and an educator on Merseyside. He is best known for three books on geometry and modern algebra. In Liverpool he and Peter Giblin are known for their registered charity Mathematical Education on Merseyside which promotes enthusiasm for mathematics through sponsorship of an annual competition.
Sabir Medgidovich Gusein-Zade is a Russian mathematician and a specialist in singularity theory and its applications.
James William Peter Hirschfeld is an Australian mathematician, resident in the United Kingdom, specializing in combinatorial geometry and the geometry of finite fields. He is an emeritus professor and Tutorial Fellow at the University of Sussex.
References
- ↑ Giblin's University of Liverpool homepage
- ↑ "Peter J. Giblin", IEEE Xplore, IEEE, 2 December 2022, retrieved 27 April 2024
- ↑ Giblin, Peter, Bibliography
| International | |
|---|---|
| National | |
| Academics | |
| Other | |
 | This article about a United Kingdom mathematician is a stub. You can help Wikipedia by expanding it. |