In mathematics, the determinant is a scalar-valued function of the entries of a square matrix. The determinant of a matrix A is commonly denoted det(A), det A, or |A|. Its value characterizes some properties of the matrix and the linear map represented, on a given basis, by the matrix. In particular, the determinant is nonzero if and only if the matrix is invertible and the corresponding linear map is an isomorphism.
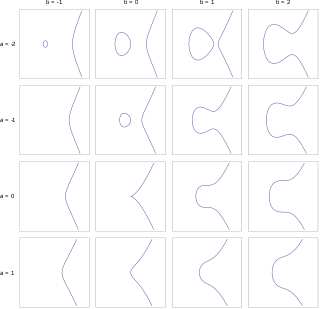
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point O. An elliptic curve is defined over a field K and describes points in K2, the Cartesian product of K with itself. If the field's characteristic is different from 2 and 3, then the curve can be described as a plane algebraic curve which consists of solutions (x, y) for:

In complex analysis, the Riemann mapping theorem states that if is a non-empty simply connected open subset of the complex number plane which is not all of , then there exists a biholomorphic mapping from onto the open unit disk
In vector calculus, the divergence theorem, also known as Gauss's theorem or Ostrogradsky's theorem, is a theorem relating the flux of a vector field through a closed surface to the divergence of the field in the volume enclosed.

The time value of money refers to the fact that there is normally a greater benefit to receiving a sum of money now rather than an identical sum later. It may be seen as an implication of the later-developed concept of time preference.
In mathematics, the Laplace operator or Laplacian is a differential operator given by the divergence of the gradient of a scalar function on Euclidean space. It is usually denoted by the symbols , (where is the nabla operator), or . In a Cartesian coordinate system, the Laplacian is given by the sum of second partial derivatives of the function with respect to each independent variable. In other coordinate systems, such as cylindrical and spherical coordinates, the Laplacian also has a useful form. Informally, the Laplacian Δf (p) of a function f at a point p measures by how much the average value of f over small spheres or balls centered at p deviates from f (p).
In differential geometry, the Ricci curvature tensor, named after Gregorio Ricci-Curbastro, is a geometric object which is determined by a choice of Riemannian or pseudo-Riemannian metric on a manifold. It can be considered, broadly, as a measure of the degree to which the geometry of a given metric tensor differs locally from that of ordinary Euclidean space or pseudo-Euclidean space.
In mathematical analysis, a function of bounded variation, also known as BV function, is a real-valued function whose total variation is bounded (finite): the graph of a function having this property is well behaved in a precise sense. For a continuous function of a single variable, being of bounded variation means that the distance along the direction of the y-axis, neglecting the contribution of motion along x-axis, traveled by a point moving along the graph has a finite value. For a continuous function of several variables, the meaning of the definition is the same, except for the fact that the continuous path to be considered cannot be the whole graph of the given function, but can be every intersection of the graph itself with a hyperplane parallel to a fixed x-axis and to the y-axis.
In Fourier analysis, a multiplier operator is a type of linear operator, or transformation of functions. These operators act on a function by altering its Fourier transform. Specifically they multiply the Fourier transform of a function by a specified function known as the multiplier or symbol. Occasionally, the term multiplier operator itself is shortened simply to multiplier. In simple terms, the multiplier reshapes the frequencies involved in any function. This class of operators turns out to be broad: general theory shows that a translation-invariant operator on a group which obeys some regularity conditions can be expressed as a multiplier operator, and conversely. Many familiar operators, such as translations and differentiation, are multiplier operators, although there are many more complicated examples such as the Hilbert transform.
In mathematical economics, the Arrow–Debreu model is a theoretical general equilibrium model. It posits that under certain economic assumptions there must be a set of prices such that aggregate supplies will equal aggregate demands for every commodity in the economy.
The money flow index (MFI) is an oscillator that ranges from 0 to 100. It is used to show the money flow over several days.
In algebraic geometry, divisors are a generalization of codimension-1 subvarieties of algebraic varieties. Two different generalizations are in common use, Cartier divisors and Weil divisors. Both are derived from the notion of divisibility in the integers and algebraic number fields.
In mathematics, a volume form or top-dimensional form is a differential form of degree equal to the differentiable manifold dimension. Thus on a manifold of dimension , a volume form is an -form. It is an element of the space of sections of the line bundle , denoted as . A manifold admits a nowhere-vanishing volume form if and only if it is orientable. An orientable manifold has infinitely many volume forms, since multiplying a volume form by a nowhere-vanishing real valued function yields another volume form. On non-orientable manifolds, one may instead define the weaker notion of a density.
In mathematics, the Riemann–Roch theorem for surfaces describes the dimension of linear systems on an algebraic surface. The classical form of it was first given by Castelnuovo, after preliminary versions of it were found by Max Noether and Enriques. The sheaf-theoretic version is due to Hirzebruch.
In mathematics, the Abel–Jacobi map is a construction of algebraic geometry which relates an algebraic curve to its Jacobian variety. In Riemannian geometry, it is a more general construction mapping a manifold to its Jacobi torus. The name derives from the theorem of Abel and Jacobi that two effective divisors are linearly equivalent if and only if they are indistinguishable under the Abel–Jacobi map.
In mathematics – specifically, in the theory of stochastic processes – Doob's martingale convergence theorems are a collection of results on the limits of supermartingales, named after the American mathematician Joseph L. Doob. Informally, the martingale convergence theorem typically refers to the result that any supermartingale satisfying a certain boundedness condition must converge. One may think of supermartingales as the random variable analogues of non-increasing sequences; from this perspective, the martingale convergence theorem is a random variable analogue of the monotone convergence theorem, which states that any bounded monotone sequence converges. There are symmetric results for submartingales, which are analogous to non-decreasing sequences.
The dual of a given linear program (LP) is another LP that is derived from the original LP in the following schematic way:
In mathematics the Function Field Sieve is one of the most efficient algorithms to solve the Discrete Logarithm Problem (DLP) in a finite field. It has heuristic subexponential complexity. Leonard Adleman developed it in 1994 and then elaborated it together with M. D. Huang in 1999. Previous work includes the work of D. Coppersmith about the DLP in fields of characteristic two.

Gale L. Pooley is an American economist, professor, and author, recognized for his work in economics and real estate valuation. Dr. Gale Pooley is currently a professor of economic history at Utah Tech University. He previously held a position as Associate Professor at Brigham Young University–Hawaii (BYU-Hawaii), where he taught in the Department of Business and Government as the lead for the economics program. His academic career also includes teaching positions at Alfaisal University in Riyadh, Brigham Young University–Idaho, Boise State University, and the College of Idaho, in the fields of business, statistics, and economics. Pooley holds professional designations from the Appraisal Institute, the Royal Institution of Chartered Surveyors, and the CCIM Institute. Pooley is renowned for his contributions to the field of economics, particularly through his work on the concept of abundance and population growth.
Marian L. Tupy is a Czechoslovak-born researcher and writer specializing in globalization, global well-being, and the politics and economics of Europe and Southern Africa. He is the editor of HumanProgress.org and a senior fellow at the Cato Institute's Center for Global Liberty and Prosperity. Tupy is known for his contributions to discussions on population growth and resource abundance.


















