Propositiones ad Acuendos Juvenes

Last updated October 31, 2024

The medieval Latin manuscript Propositiones ad Acuendos Juvenes (English: Problems to Sharpen the Young) is one of the earliest known collections of recreational mathematics problems.^[1] The oldest known copy of the manuscript dates from the late 9th century. The text is attributed to Alcuin of York (died 804.) Some editions of the text contain 53 problems, others 56. It has been translated into English by John Hadley, with annotations by John Hadley and David Singmaster.^[2]

The manuscript contains the first known occurrences of several types of problem, including three river-crossing problems:

Problem 17: The jealous husbands problem. In Alcuin's version of this problem, three men, each with a sister, must cross a boat which can carry only two people, so that a woman whose brother is not present is never left in the company of another man,^[2]^{, p. 111.}
Problem 18: The problem of the wolf, goat, and cabbage ^[2]^{, p. 112.}, and
Problem 19: Propositio de viro et muliere ponderantibus plaustrum. In this problem, a man and a woman of equal weight, together with two children, each of half their weight, wish to cross a river using a boat which can only carry the weight of one adult;^[2]^{, p. 112.}

a so-called "barrel-sharing" problem:

Problem 12: A certain father died and left as an inheritance to his three sons 30 glass flasks, of which 10 were full of oil, another 10 were half full, while another 10 were empty. Divide the oil and flasks so that an equal share of the commodities should equally come down to the three sons, both of oil and glass;^[2]^{, p. 109.} The number of solutions to this problem for n of each type of flask are terms of Alcuin's sequence.

a variant of the jeep problem:

Problem 52: A certain head of household ordered that 90 modia of grain be taken from one of his houses to another 30 leagues away. Given that this load of grain can be carried by a camel in three trips and that the camel eats one modius per league, how many modia were left over at the end of the journey?^[2]^{, pp. 124–125.}

and three packing problems:^[3]

Problem 27: Proposition concerning a quadrangular city. There is a quadrangular city which has one side of 1100 feet, another side of 1000 feet, a front of 600 feet, and a final side of 600 feet. I want to put some houses there so that each house is 40 feet long and 30 feet wide. Let him say, he who wishes, How many houses ought the city to contain?
Problem 28: Proposition concerning a triangular city. There is a triangular city which has one side of 100 feet, another side of 100 feet, and a third of 90 feet. Inside of this, I want to build a structure of houses, however, in such a way that each house is 20 feet in length, 10 feet in width. Let him say, he who can, How many houses should be contained?
Problem 29: Proposition concerning a round city. There is a city which is 8000 feet in circumference. Let him say, he who is able, How many houses should the city contain, such that each [house] is 30 feet long, and 20 feet wide?

Some further problems are:

Problem 5: A merchant wanted to buy 100 pigs for 100 pence. For a boar, he would pay 10 pence; for a sow, 5 pence; while he would pay 1 penny for a couple of piglets. How many boars, sows, and piglets must there have been for him to have paid exactly 100 pence for the 100 animals?

This problem dates back at least as far as 5th century China, and occurs in Indian and Arabic texts of the time.^[2]^{, p. 106.}

Problems 32, 33, 34, 38, 39, and 47^[4] are similar, in that each divides a given quantity of money or food among a given number of people or animals consisting of three types, according to set ratios, and asks the number of each type. Algebraically, this is equivalent to two equations in three unknowns. However, since a sensible solution can only have whole people or animals, most of the problems have only one solution consisting of positive integers. In each case, Alcuin gives a solution and proves that it is correct, without describing how the solution was found.

Problem 26: There is a field that is 150 feet long. At one end stood a dog; at the other, a hare. The dog chased the hare. Whereas the dog went 9 feet per stride, the hare went only 7. How many feet and how many leaps did the dog take in pursuing the fleeing hare until it was caught?

Overtaking problems of this type date back to 150 BC, but this is the first known European example.^[2]^{, p. 115.}

Problem 42: There is a staircase that has 100 steps. One pigeon sat on the first step, two pigeons on the second, three on the third, four on the fourth, five on the fifth, and so on up to the hundredth step. How many pigeons were there in all?

Note that this word problem is equivalent to the arithmetic problem of adding all numbers from 1 through 100. Alcuin's solution is to note that there are 100 pigeons in total on the first and 99th steps combined, 100 more on the second and 98th combined, and so on for all the pairs of steps, except the 50th and 100th. Carl Friedrich Gauss as a pupil is presumed to have solved the equivalent arithmetic problem by pairing 1 and 100, 2 and 99, ..., 50 and 51, thus yielding 50 times 101 = 5050, a solution which is more elegant than Alcuin's solution 1000 years before.^[2]^{, p. 121.}

Problem 43: A certain man has 300 pigs. He ordered all of them slaughtered in 3 days, but with an uneven number killed each day. What number were to be killed each day?

This problem seems to be composed for rebuking troublesome students, and no solution is given. (Three odd numbers cannot add up to 300.)^[2]^{, p. 121.}

Problem 14: How many footprints in the last furrow does an ox make which has been plowing all day?^[4]

Another humorous problem: the answer is none, as the plow destroys them in making the furrow.

Related Research Articles

Alcuin of York – also called Ealhwine, Alhwin, or Alchoin – was a scholar, clergyman, poet, and teacher from York, Northumbria. He was born around 735 and became the student of Archbishop Ecgbert at York. At the invitation of Charlemagne, he became a leading scholar and teacher at the Carolingian court, where he remained a figure in the 780s and 790s. Before that, he was also a court chancellor in Aachen. "The most learned man anywhere to be found", according to Einhard's Life of Charlemagne, he is considered among the most important intellectual architects of the Carolingian Renaissance. Among his pupils were many of the dominant intellectuals of the Carolingian era.

The Rubik's Cube is a 3D combination puzzle invented in 1974 by Hungarian sculptor and professor of architecture Ernő Rubik. Originally called the Magic Cube, the puzzle was licensed by Rubik to be sold by Pentangle Puzzles in the UK in 1978, and then by Ideal Toy Corp in 1980 via businessman Tibor Laczi and Seven Towns founder Tom Kremer. The cube was released internationally in 1980 and became one of the most recognized icons in popular culture. It won the 1980 German Game of the Year special award for Best Puzzle. As of January 2024, around 500 million cubes had been sold worldwide, making it the world's bestselling puzzle game and bestselling toy. The Rubik's Cube was inducted into the US National Toy Hall of Fame in 2014.

In mathematics, the harmonic series is the infinite series formed by summing all positive unit fractions:

<span class="mw-page-title-main">Apollonius of Perga</span> Ancient Greek geometer and astronomer (c. 240–190 BC)

Apollonius of Perga was an ancient Greek geometer and astronomer known for his work on conic sections. Beginning from the earlier contributions of Euclid and Archimedes on the topic, he brought them to the state prior to the invention of analytic geometry. His definitions of the terms ellipse, parabola, and hyperbola are the ones in use today. With his predecessors Euclid and Archimedes, Apollonius is generally considered among the greatest mathematicians of antiquity.

Claude Gaspar Bachet Sieur de Méziriac was a French mathematician and poet born in Bourg-en-Bresse, at that time belonging to Duchy of Savoy. He wrote Problèmes plaisans et délectables qui se font par les nombres, Les éléments arithmétiques, and a Latin translation of the Arithmetica of Diophantus. He also discovered means of solving indeterminate equations using continued fractions, a method of constructing magic squares, and a proof of Bézout's identity.

<span class="mw-page-title-main">Optimal solutions for the Rubik's Cube</span>

Optimal solutions for the Rubik's Cube are solutions that are the shortest in some sense. There are two common ways to measure the length of a solution. The first is to count the number of quarter turns. The second is to count the number of outer-layer twists, called "face turns". A move to turn an outer layer two quarter (90°) turns in the same direction would be counted as two moves in the quarter turn metric (QTM), but as one turn in the face metric.

Pappus of Alexandria was a Greek mathematician of late antiquity known for his Synagoge (Συναγωγή) or Collection, and for Pappus's hexagon theorem in projective geometry. Almost nothing is known about his life except for what can be found in his own writings, many of which are lost. Pappus apparently lived in Alexandria, where he worked as a mathematics teacher to higher level students, one of whom was named Hermodorus.

The wolf, goat and cabbage problem is a river crossing puzzle. It dates back to at least the 9th century, and has entered the folklore of several cultures.

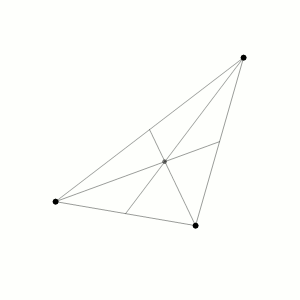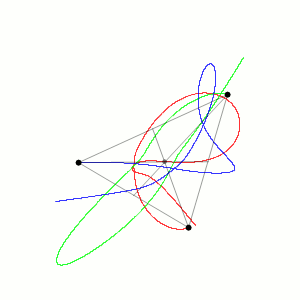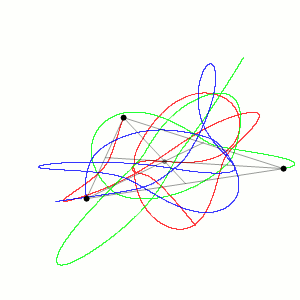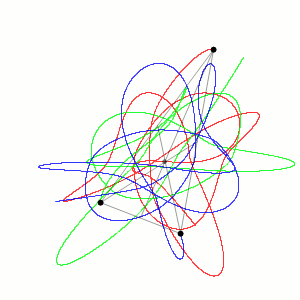
<span class="mw-page-title-main">Three-body problem</span> Physics problem related to laws of motion and gravity

In physics, specifically classical mechanics, the three-body problem is to take the initial positions and velocities of three point masses that orbit each other in space and calculate their subsequent trajectories using Newton's laws of motion and Newton's law of universal gravitation.

David Breyer Singmaster was an American-British mathematician who was emeritus professor of mathematics at London South Bank University, England. He had a huge personal collection of mechanical puzzles and books of brain teasers. He was most famous for being an early adopter and enthusiastic promoter of the Rubik's Cube. His Notes on Rubik's "Magic Cube" which he began compiling in 1979 provided the first mathematical analysis of the Cube as well as providing one of the first published solutions. The book contained his cube notation which allowed the recording of Rubik's Cube moves, and which quickly became the standard.

The missionaries and cannibals problem, and the closely related jealous husbands problem, are classic river-crossing logic puzzles. The missionaries and cannibals problem is a well-known toy problem in artificial intelligence, where it was used by Saul Amarel as an example of problem representation.

A porism is a mathematical proposition or corollary. It has been used to refer to a direct consequence of a proof, analogous to how a corollary refers to a direct consequence of a theorem. In modern usage, it is a relationship that holds for an infinite range of values but only if a certain condition is assumed, such as Steiner's porism. The term originates from three books of Euclid that have been lost. A proposition may not have been proven, so a porism may not be a theorem or true.

A river crossing puzzle is a type of puzzle in which the object is to carry items from one river bank to another, usually in the fewest trips. The difficulty of the puzzle may arise from restrictions on which or how many items can be transported at the same time, or which or how many items may be safely left together. The setting may vary cosmetically, for example, by replacing the river by a bridge. The earliest known river-crossing problems occur in the manuscript Propositiones ad Acuendos Juvenes, traditionally said to be written by Alcuin. The earliest copies of this manuscript date from the 9th century; it contains three river-crossing problems, including the fox, goose, and bag of beans puzzle and the jealous husbands problem.

The missing dollar riddle is a famous riddle that involves an informal fallacy. It dates to at least the 1930s, although similar puzzles are much older.

In mathematics, specifically in elementary arithmetic and elementary algebra, given an equation between two fractions or rational expressions, one can cross-multiply to simplify the equation or determine the value of a variable.

In the wine/water mixing problem, one starts with two barrels, one holding wine and the other an equal volume of water. A cup of wine is taken from the wine barrel and added to the water. A cup of the wine/water mixture is then returned to the wine barrel, so that the volumes in the barrels are again equal. The question is then posed—which of the two mixtures is purer? The answer is that the mixtures will be of equal purity. The solution still applies no matter how many cups of any sizes and compositions are exchanged, or how little or much stirring at any point in time is done to any barrel, as long as at the end each barrel has the same amount of liquid.

The jeep problem, desert crossing problem or exploration problem is a mathematics problem in which a jeep must maximize the distance it can travel into a desert with a given quantity of fuel. The jeep can only carry a fixed and limited amount of fuel, but it can leave fuel and collect fuel at fuel dumps anywhere in the desert.

<i>The Simple Solution to Rubiks Cube</i> 1981 book by James G. Nourse

The Simple Solution to Rubik's Cube by James G. Nourse is a book that was published in 1981. The book explains how to solve the Rubik's Cube. The book became the best-selling book of 1981, selling 6,680,000 copies that year. It was the fastest-selling title in the 36-year history of Bantam Books.

In mathematics, Alcuin's sequence, named after Alcuin of York, is the sequence of coefficients of the power-series expansion of:

The Hundred Fowls Problem is a problem first discussed in the fifth century CE Chinese mathematics text Zhang Qiujian suanjing, a book of mathematical problems written by Zhang Qiujian. It is one of the best known examples of indeterminate problems in the early history of mathematics. The problem appears as the final problem in Zhang Qiujian suanjing. However, the problem and its variants have appeared in the medieval mathematical literature of India, Europe and the Arab world.

References

↑ Alcuin (735-804), David Darling, The Internet Encyclopedia of Science. Accessed on line February 7, 2008.
1 2 3 4 5 6 7 8 9 10 Problems to Sharpen the Young, John Hadley and David Singmaster, The Mathematical Gazette, 76, #475 (March 1992), pp. 102–126.
↑ Nikolai Yu. Zolotykh, Alcuin's Propositiones de Civitatibus: the Earliest Packing Problems. arXiv preprint arXiv : 1308.0892 (2013)
1 2 Burkholder, Peter J. "Alcuin of York's "Propositiones ad Acuendos Juvenes"" (PDF). Retrieved 6 January 2020.

External links and further reading

(in Latin) Propositiones ad acuendos iuuenes, Latin text.
Problems to Sharpen the Young, John Hadley and David Singmaster, The Mathematical Gazette, 76, #475 (March 1992), pp. 102–126. Annotated translation of the text into English.
(in English and Latin) HOST: An Electronic Bulletin for the History and Philosophy of Science and Technology, 1, #2 (Spring/Summer; June 1993), ISSN 1192-084X. Contains a translation by Peter J. Burkholder of the text into English, together with introduction, commentary, and the original text.
Rutger Kramer, "'Ecce fabula!' Problem-Solving by Numbers in the Carolingian World: The Case of the Propositiones ad Acuendos Iuvenes", http://epub.oeaw.ac.at/0xc1aa5576_0x0036d428.pdf
Nikolai Yu. Zolotykh, Alcuin's Propositiones de Civitatibus: the Earliest Packing Problems. arXiv preprint arXiv : 1308.0892 (2013)

This page is based on this Wikipedia article
Text is available under the CC BY-SA 4.0 license; additional terms may apply.
Images, videos and audio are available under their respective licenses.

[1] Alcuin (735-804), David Darling, The Internet Encyclopedia of Science. Accessed on line February 7, 2008.

[a-2] 1 2 3 4 5 6 7 8 9 10 Problems to Sharpen the Young, John Hadley and David Singmaster, The Mathematical Gazette, 76, #475 (March 1992), pp. 102–126.

[zolotykh-3] Nikolai Yu. Zolotykh, Alcuin's Propositiones de Civitatibus: the Earliest Packing Problems. arXiv preprint arXiv : 1308.0892 (2013)

[burk-4] 1 2 Burkholder, Peter J. "Alcuin of York's "Propositiones ad Acuendos Juvenes"" (PDF). Retrieved 6 January 2020.

[1]

[2]

[3]

[4]