Definition
The Racah parameters are defined as
where are Slater integrals
and are the Slater-Condon parameters
where is the normalized radial part of an electron orbital, and . [2]
The Racah parameters are a set of parameters used in atomic and molecular spectroscopy to describe the amount of total electrostatic repulsion in an atom that has multiple electrons.
When an atom has more than one electron, there will be some electrostatic repulsion between the electrons. The amount of repulsion varies from atom to atom, depending upon the number of electrons, their spin, and the orbitals that they occupy. The total repulsion can be expressed in terms of three parameters A, B and C which are known as the Racah parameters after Giulio Racah, who first described them. They are generally obtained empirically from gas-phase spectroscopic studies of atoms. [1]
They are often used in transition-metal chemistry to describe the repulsion energy associated with an electronic term. For example, the interelectronic repulsion of a 3P term is A + 7B, and of a 3F term is A - 8B, and the difference between them is therefore 15B.
The Racah parameters are defined as
where are Slater integrals
and are the Slater-Condon parameters
where is the normalized radial part of an electron orbital, and . [2]
In spectroscopy, the Rydberg constant, symbol for heavy atoms or for hydrogen, named after the Swedish physicist Johannes Rydberg, is a physical constant relating to the electromagnetic spectra of an atom. The constant first arose as an empirical fitting parameter in the Rydberg formula for the hydrogen spectral series, but Niels Bohr later showed that its value could be calculated from more fundamental constants according to his model of the atom.
In mathematics, a Gaussian function, often simply referred to as a Gaussian, is a function of the base form
In geometry and complex analysis, a Möbius transformation of the complex plane is a rational function of the form
In computational physics and chemistry, the Hartree–Fock (HF) method is a method of approximation for the determination of the wave function and the energy of a quantum many-body system in a stationary state.
In physics, the S-matrix or scattering matrix relates the initial state and the final state of a physical system undergoing a scattering process. It is used in quantum mechanics, scattering theory and quantum field theory (QFT).
A bound state is a composite of two or more fundamental building blocks, such as particles, atoms, or bodies, that behaves as a single object and in which energy is required to split them.
A continuous-time Markov chain (CTMC) is a continuous stochastic process in which, for each state, the process will change state according to an exponential random variable and then move to a different state as specified by the probabilities of a stochastic matrix. An equivalent formulation describes the process as changing state according to the least value of a set of exponential random variables, one for each possible state it can move to, with the parameters determined by the current state.
In physics, tunnel ionization is a process in which electrons in an atom tunnel through the potential barrier and escape from the atom. In an intense electric field, the potential barrier of an atom (molecule) is distorted drastically. Therefore, as the length of the barrier that electrons have to pass decreases, the electrons can escape from the atom's potential more easily. Tunneling ionization is a quantum mechanical phenomenon, since in the classical picture an electron does not have sufficient energy to overcome the potential barrier of the atom.
Jellium, also known as the uniform electron gas (UEG) or homogeneous electron gas (HEG), is a quantum mechanical model of interacting electrons in a solid where the positive charges are assumed to be uniformly distributed in space; the electron density is a uniform quantity as well in space. This model allows one to focus on the effects in solids that occur due to the quantum nature of electrons and their mutual repulsive interactions without explicit introduction of the atomic lattice and structure making up a real material. Jellium is often used in solid-state physics as a simple model of delocalized electrons in a metal, where it can qualitatively reproduce features of real metals such as screening, plasmons, Wigner crystallization and Friedel oscillations.
In quantum physics, the spin–orbit interaction is a relativistic interaction of a particle's spin with its motion inside a potential. A key example of this phenomenon is the spin–orbit interaction leading to shifts in an electron's atomic energy levels, due to electromagnetic interaction between the electron's magnetic dipole, its orbital motion, and the electrostatic field of the positively charged nucleus. This phenomenon is detectable as a splitting of spectral lines, which can be thought of as a Zeeman effect product of two relativistic effects: the apparent magnetic field seen from the electron perspective and the magnetic moment of the electron associated with its intrinsic spin. A similar effect, due to the relationship between angular momentum and the strong nuclear force, occurs for protons and neutrons moving inside the nucleus, leading to a shift in their energy levels in the nucleus shell model. In the field of spintronics, spin–orbit effects for electrons in semiconductors and other materials are explored for technological applications. The spin–orbit interaction is at the origin of magnetocrystalline anisotropy and the spin Hall effect.
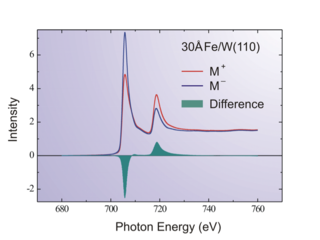
X-ray magnetic circular dichroism (XMCD) is a difference spectrum of two X-ray absorption spectra (XAS) taken in a magnetic field, one taken with left circularly polarized light, and one with right circularly polarized light. By closely analyzing the difference in the XMCD spectrum, information can be obtained on the magnetic properties of the atom, such as its spin and orbital magnetic moment. Using XMCD magnetic moments below 10−5µB can be observed.
In coordination chemistry, Tanabe–Sugano diagrams are used to predict absorptions in the ultraviolet (UV), visible and infrared (IR) electromagnetic spectrum of coordination compounds. The results from a Tanabe–Sugano diagram analysis of a metal complex can also be compared to experimental spectroscopic data. They are qualitatively useful and can be used to approximate the value of 10Dq, the ligand field splitting energy. Tanabe–Sugano diagrams can be used for both high spin and low spin complexes, unlike Orgel diagrams, which apply only to high spin complexes. Tanabe–Sugano diagrams can also be used to predict the size of the ligand field necessary to cause high-spin to low-spin transitions.
The theoretical and experimental justification for the Schrödinger equation motivates the discovery of the Schrödinger equation, the equation that describes the dynamics of nonrelativistic particles. The motivation uses photons, which are relativistic particles with dynamics described by Maxwell's equations, as an analogue for all types of particles.
A hydrogen-like atom (or hydrogenic atom) is any atom or ion with a single valence electron. These atoms are isoelectronic with hydrogen. Examples of hydrogen-like atoms include, but are not limited to, hydrogen itself, all alkali metals such as Rb and Cs, singly ionized alkaline earth metals such as Ca+ and Sr+ and other ions such as He+, Li2+, and Be3+ and isotopes of any of the above. A hydrogen-like atom includes a positively charged core consisting of the atomic nucleus and any core electrons as well as a single valence electron. Because helium is common in the universe, the spectroscopy of singly ionized helium is important in EUV astronomy, for example, of DO white dwarf stars.
The nephelauxetic effect is a term used in the inorganic chemistry of transition metals. It refers to a decrease in the Racah interelectronic repulsion parameter, given the symbol B, that occurs when a transition-metal free ion forms a complex with ligands. The name "nephelauxetic" comes from the Greek for cloud-expanding and was proposed by the Danish inorganic chemist C. K. Jorgensen. The presence of this effect highlights the disadvantages of crystal field theory, which treats metal-ligand interactions as purely electrostatic, since the nephelauxetic effect reveals the covalent character in the metal-ligand interaction.
Spin is an intrinsic form of angular momentum carried by elementary particles, and thus by composite particles such as hadrons, atomic nuclei, and atoms. The spinning electron was originally proposed as a small rigid particle rotating about an axis, as ordinary use of the word may suggest. Instead, as described by Ohanian, "spin may be regarded as an angular momentum generated by a circulating flow of charge in the wave field of the electron".
A helium atom is an atom of the chemical element helium. Helium is composed of two electrons bound by the electromagnetic force to a nucleus containing two protons along with two neutrons, depending on the isotope, held together by the strong force. Unlike for hydrogen, a closed-form solution to the Schrödinger equation for the helium atom has not been found. However, various approximations, such as the Hartree–Fock method, can be used to estimate the ground state energy and wavefunction of the atom. Historically, the first such helium spectrum calculation was done by Albrecht Unsöld in 1927. Its success was considered to be one of the earliest signs of validity of Schrödinger's wave mechanics.
In quantum chemistry, Brillouin's theorem, proposed by the French physicist Léon Brillouin in 1934, relates to Hartree–Fock wavefunctions. Hartree–Fock, or the self-consistent field method, is a non-relativistic method of generating approximate wavefunctions for a many-bodied quantum system, based on the assumption that each electron is exposed to an average of the positions of all other electrons, and that the solution is a linear combination of pre-specified basis functions.

Metal L-edge spectroscopy is a spectroscopic technique used to study the electronic structures of transition metal atoms and complexes. This method measures X-ray absorption caused by the excitation of a metal 2p electron to unfilled d orbitals, which creates a characteristic absorption peak called the L-edge. Similar features can also be studied by Electron Energy Loss Spectroscopy. According to the selection rules, the transition is formally electric-dipole allowed, which not only makes it more intense than an electric-dipole forbidden metal K pre-edge transition, but also makes it more feature-rich as the lower required energy results in a higher-resolution experiment.
In solid state physics the electronic specific heat, sometimes called the electron heat capacity, is the specific heat of an electron gas. Heat is transported by phonons and by free electrons in solids. For pure metals, however, the electronic contributions dominate in the thermal conductivity. In impure metals, the electron mean free path is reduced by collisions with impurities, and the phonon contribution may be comparable with the electronic contribution.