Related Research Articles

A quasiperiodic crystal, or quasicrystal, is a structure that is ordered but not periodic. A quasicrystalline pattern can continuously fill all available space, but it lacks translational symmetry. While crystals, according to the classical crystallographic restriction theorem, can possess only two-, three-, four-, and six-fold rotational symmetries, the Bragg diffraction pattern of quasicrystals shows sharp peaks with other symmetry orders—for instance, five-fold.

Wang tiles, first proposed by mathematician, logician, and philosopher Hao Wang in 1961, are a class of formal systems. They are modelled visually by square tiles with a color on each side. A set of such tiles is selected, and copies of the tiles are arranged side by side with matching colors, without rotating or reflecting them.

A tessellation or tiling is the covering of a surface, often a plane, using one or more geometric shapes, called tiles, with no overlaps and no gaps. In mathematics, tessellation can be generalized to higher dimensions and a variety of geometries.
In mathematical logic and theoretical computer science, a register machine is a generic class of abstract machines used in a manner similar to a Turing machine. All models of register machines are Turing equivalent.

An aperiodic tiling is a non-periodic tiling with the additional property that it does not contain arbitrarily large periodic regions or patches. A set of tile-types is aperiodic if copies of these tiles can form only non-periodic tilings.
Hao Wang was a Chinese-American logician, philosopher, mathematician, and commentator on Kurt Gödel.

Jarkko J. Kari is a Finnish mathematician and computer scientist, known for his contributions to the theory of Wang tiles and cellular automata. Kari is currently a professor at the Department of Mathematics, University of Turku.
In geometry, a tile substitution is a method for constructing highly ordered tilings. Most importantly, some tile substitutions generate aperiodic tilings, which are tilings whose prototiles do not admit any tiling with translational symmetry. The most famous of these are the Penrose tilings. Substitution tilings are special cases of finite subdivision rules, which do not require the tiles to be geometrically rigid.

The Harvard John A. Paulson School of Engineering and Applied Sciences (SEAS) is the engineering school within Harvard University's Faculty of Arts and Sciences, offering degrees in engineering and applied sciences to graduate students admitted directly to SEAS, and to undergraduates admitted first to Harvard College. Previously the Lawrence Scientific School and then the Division of Engineering and Applied Sciences, the Paulson School assumed its current structure in 2007. David C. Parkes has been its dean since 2023.

In geometry, a domino tiling of a region in the Euclidean plane is a tessellation of the region by dominoes, shapes formed by the union of two unit squares meeting edge-to-edge. Equivalently, it is a perfect matching in the grid graph formed by placing a vertex at the center of each square of the region and connecting two vertices when they correspond to adjacent squares.
A three-dimensional integrated circuit is a MOS integrated circuit (IC) manufactured by stacking as many as 16 or more ICs and interconnecting them vertically using, for instance, through-silicon vias (TSVs) or Cu-Cu connections, so that they behave as a single device to achieve performance improvements at reduced power and smaller footprint than conventional two dimensional processes. The 3D IC is one of several 3D integration schemes that exploit the z-direction to achieve electrical performance benefits in microelectronics and nanoelectronics.
In mathematics, a domino is a polyomino of order 2, that is, a polygon in the plane made of two equal-sized squares connected edge-to-edge. When rotations and reflections are not considered to be distinct shapes, there is only one free domino.

A Penrose tiling is an example of an aperiodic tiling. Here, a tiling is a covering of the plane by non-overlapping polygons or other shapes, and a tiling is aperiodic if it does not contain arbitrarily large periodic regions or patches. However, despite their lack of translational symmetry, Penrose tilings may have both reflection symmetry and fivefold rotational symmetry. Penrose tilings are named after mathematician and physicist Roger Penrose, who investigated them in the 1970s.
Flexible solar cell research is a research-level technology, an example of which was created at the Massachusetts Institute of Technology in which solar cells are manufactured by depositing photovoltaic material on flexible substrates, such as ordinary paper, using chemical vapor deposition technology.
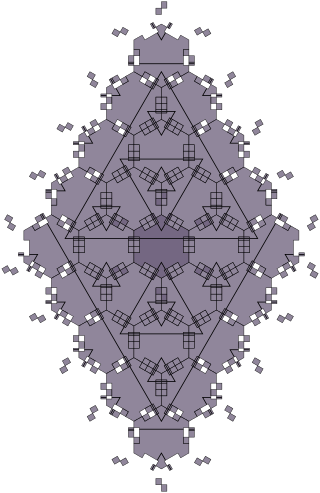
The Socolar–Taylor tile is a single non-connected tile which is aperiodic on the Euclidean plane, meaning that it admits only non-periodic tilings of the plane, with rotations and reflections of the tile allowed. It is the first known example of a single aperiodic tile, or "einstein". The basic version of the tile is a simple hexagon, with printed designs to enforce a local matching rule, regarding how the tiles may be placed. It is currently unknown whether this rule may be geometrically implemented in two dimensions while keeping the tile a connected set.

A set of prototiles is aperiodic if copies of the prototiles can be assembled to create tilings, such that all possible tessellation patterns are non-periodic. The aperiodicity referred to is a property of the particular set of prototiles; the various resulting tilings themselves are just non-periodic.
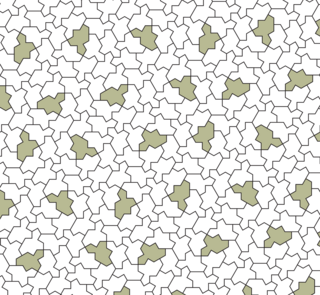
In plane geometry, the einstein problem asks about the existence of a single prototile that by itself forms an aperiodic set of prototiles; that is, a shape that can tessellate space but only in a nonperiodic way. Such a shape is called an einstein, a word play on ein Stein, German for "one stone".

Chaim Goodman-Strauss is an American mathematician who works in convex geometry, especially aperiodic tiling. He retired from the faculty of the University of Arkansas and currently serves as outreach mathematician for the National Museum of Mathematics. He is co-author with John H. Conway and Heidi Burgiel of The Symmetries of Things, a comprehensive book surveying the mathematical theory of patterns.
Polyominoes: Puzzles, Patterns, Problems, and Packings is a mathematics book on polyominoes, the shapes formed by connecting some number of unit squares edge-to-edge. It was written by Solomon Golomb, and is "universally regarded as a classic in recreational mathematics". The Basic Library List Committee of the Mathematical Association of America has strongly recommended its inclusion in undergraduate mathematics libraries.
References
- ↑ Darling, David J. (2004). The universal book of mathematics: from Abracadabra to Zeno's paradoxes. John Wiley and Sons. pp. 18–. ISBN 978-0-471-27047-8 . Retrieved 29 September 2011.
- ↑ Büchi, J. R. "The undecidability of the domino problem". Mathematical Reviews . 36 (49). MR 0216954.
- ↑ Author biography from Raffel, J. I.; Mann, J. R.; Berger, R.; Soares, A. M.; Gilbert, S. (1989), "A generic architecture for wafer-scale neuromorphic systems" (PDF), The Lincoln Laboratory Journal, 2 (1): 63–76, Bibcode:1989LLabJ...2...63R, archived from the original (PDF) on 2012-05-21, retrieved 2011-09-30.
- ↑ Berger, Robert (1966), "The Undecidability of the Domino Problem", Memoirs of the American Mathematical Society , 66 (66): 72 pp, doi:10.1090/memo/0066 .
- ↑ Robert Berger at the Mathematics Genealogy Project.
- ↑ Awards and Recognition, Lincoln Laboratory Annual Report 2010, p. 50, retrieved 2011-09-30.
- ↑ MIT Lincoln Laboratory receives five R&D 100 Awards, Lincoln Laboratory, retrieved 2011-09-30.