
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre; equivalently it is the curve traced out by a point that moves in a plane so that its distance from a given point is constant. The distance between any point of the circle and the centre is called the radius. Usually, the radius is required to be a positive number. This article is about circles in Euclidean geometry, and, in particular, the Euclidean plane, except where otherwise noted.

In elementary geometry, two geometric objects are perpendicular if they intersect at a right angle.

In geometry, the nine-point circle is a circle that can be constructed for any given triangle. It is so named because it passes through nine significant concyclic points defined from the triangle. These nine points are:

In geometry, an orthocentric system is a set of four points on a plane, one of which is the orthocenter of the triangle formed by the other three.
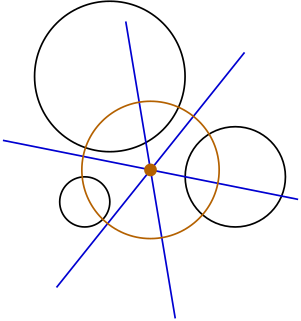
In geometry, the power center of three circles, also called the radical center, is the intersection point of the three radical axes of the pairs of circles. If the radical center lies outside of all three circles, then it is the center of the unique circle that intersects the three given circles orthogonally; the construction of this orthogonal circle corresponds to Monge's problem. This is a special case of the three conics theorem.
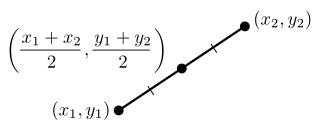
In geometry, the midpoint is the middle point of a line segment. It is equidistant from both endpoints, and it is the centroid both of the segment and of the endpoints. It bisects the segment.

In geometry, a set of points are said to be concyclic if they lie on a common circle. All concyclic points are at the same distance from the center of the circle. Three points in the plane that do not all fall on a straight line are concyclic, but four or more such points in the plane are not necessarily concyclic.

In projective geometry, Pascal's theorem states that if six arbitrary points are chosen on a conic and joined by line segments in any order to form a hexagon, then the three pairs of opposite sides of the hexagon meet at three points which lie on a straight line, called the Pascal line of the hexagon. It is named after Blaise Pascal.
Lines in a plane or higher-dimensional space are said to be concurrent if they intersect at a single point. They are in contrast to parallel lines.

In mathematics, specifically in incidence geometry and especially in projective geometry, a complete quadrangle is a system of geometric objects consisting of any four points in a plane, no three of which are on a common line, and of the six lines connecting the six pairs of points. Dually, a complete quadrilateral is a system of four lines, no three of which pass through the same point, and the six points of intersection of these lines. The complete quadrangle was called a tetrastigm by Lachlan (1893), and the complete quadrilateral was called a tetragram; those terms are occasionally still used.
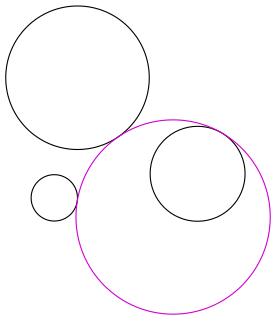
In Euclidean plane geometry, Apollonius's problem is to construct circles that are tangent to three given circles in a plane (Figure 1). Apollonius of Perga posed and solved this famous problem in his work Ἐπαφαί ; this work has been lost, but a 4th-century AD report of his results by Pappus of Alexandria has survived. Three given circles generically have eight different circles that are tangent to them (Figure 2), a pair of solutions for each way to divide the three given circles in two subsets.

The four-vertex theorem of geometry states that the curvature function of a simple, closed, smooth plane curve has at least four local extrema. The name of the theorem derives from the convention of calling an extreme point of the curvature function a vertex. This theorem has many generalizations, including a version for space curves where a vertex is defined as a point of vanishing torsion.

In geometry, a set of Johnson circles comprises three circles of equal radius r sharing one common point of intersection H. In such a configuration the circles usually have a total of four intersections : the common point H that they all share, and for each of the three pairs of circles one more intersection point. If any two of the circles happen to osculate, they only have H as a common point, and it will then be considered that H be their 2-wise intersection as well; if they should coincide we declare their 2-wise intersection be the point diametrically opposite H. The three 2-wise intersection points define the reference triangle of the figure. The concept is named after Roger Arthur Johnson.

In geometry, Monge's theorem, named after Gaspard Monge, states that for any three circles in a plane, none of which is completely inside one of the others, the intersection points of each of the three pairs of external tangent lines are collinear.

In geometry, a Steiner chain is a set of n circles, all of which are tangent to two given non-intersecting circles, where n is finite and each circle in the chain is tangent to the previous and next circles in the chain. In the usual closed Steiner chains, the first and last (nth) circles are also tangent to each other; by contrast, in open Steiner chains, they need not be. The given circles α and β do not intersect, but otherwise are unconstrained; the smaller circle may lie completely inside or outside of the larger circle. In these cases, the centers of Steiner-chain circles lie on an ellipse or a hyperbola, respectively.
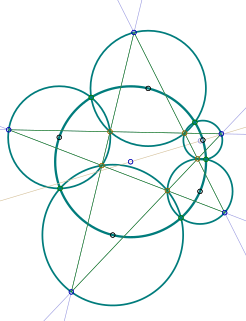
In geometry, the five circles theorem states that, given five circles centered on a common sixth circle and intersecting each other chainwise on the same circle, the lines joining their second intersection points forms a pentagram whose points lie on the circles themselves.

Miquel's theorem is a result in geometry, named after Auguste Miquel, concerning the intersection of three circles, each drawn through one vertex of a triangle and two points on its adjacent sides. It is one of several results concerning circles in Euclidean geometry due to Miquel, whose work was published in Liouville's newly founded journal Journal de mathématiques pures et appliquées.

In geometry, Clifford's theorems, named after the English geometer William Kingdon Clifford, are a sequence of theorems relating to intersections of circles.
In geometry, tangent circles are circles in a common plane that intersect in a single point. There are two types of tangency: internal and external. Many problems and constructions in geometry are related to tangent circles; such problems often have real-life applications such as trilateration and maximizing the use of materials.

In plane geometry, an extended side or sideline of a polygon is the line that contains one side of the polygon. The extension of a side arises in various contexts.


















