
Diffraction refers to various phenomena that occur when a wave encounters an obstacle or a slit. It is defined as the bending of waves around the corners of an obstacle or through an aperture into the region of geometrical shadow of the obstacle/aperture. The diffracting object or aperture effectively becomes a secondary source of the propagating wave. Italian scientist Francesco Maria Grimaldi coined the word "diffraction" and was the first to record accurate observations of the phenomenon in 1660.

In optics, the refractive index of a material is a dimensionless number that describes how fast light travels through the material. It is defined as
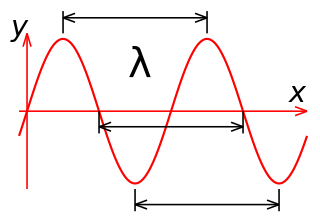
In physics, the wavelength is the spatial period of a periodic wave—the distance over which the wave's shape repeats. It is the distance between consecutive corresponding points of the same phase on the wave, such as two adjacent crests, troughs, or zero crossings, and is a characteristic of both traveling waves and standing waves, as well as other spatial wave patterns. The inverse of the wavelength is called the spatial frequency. Wavelength is commonly designated by the Greek letter lambda (λ). The term wavelength is also sometimes applied to modulated waves, and to the sinusoidal envelopes of modulated waves or waves formed by interference of several sinusoids.
In physics, coherence length is the propagation distance over which a coherent wave maintains a specified degree of coherence. Wave interference is strong when the paths taken by all of the interfering waves differ by less than the coherence length. A wave with a longer coherence length is closer to a perfect sinusoidal wave. Coherence length is important in holography and telecommunications engineering.

In the physical sciences, the wavenumber is the spatial frequency of a wave, measured in cycles per unit distance or radians per unit distance. Whereas temporal frequency can be thought of as the number of waves per unit time, wavenumber is the number of waves per unit distance.

In optics, dispersion is the phenomenon in which the phase velocity of a wave depends on its frequency. Media having this common property may be termed dispersive media. Sometimes the term chromatic dispersion is used for specificity. Although the term is used in the field of optics to describe light and other electromagnetic waves, dispersion in the same sense can apply to any sort of wave motion such as acoustic dispersion in the case of sound and seismic waves, in gravity waves, and for telecommunication signals along transmission lines or optical fiber.
Angular resolution describes the ability of any image-forming device such as an optical or radio telescope, a microscope, a camera, or an eye, to distinguish small details of an object, thereby making it a major determinant of image resolution. The closely related term spatial resolution refers to the precision of a measurement with respect to space, which is directly connected to angular resolution in imaging instruments.

An optical telescope is a telescope that gathers and focuses light, mainly from the visible part of the electromagnetic spectrum, to create a magnified image for direct view, or to make a photograph, or to collect data through electronic image sensors.

The resolution of an optical imaging system – a microscope, telescope, or camera – can be limited by factors such as imperfections in the lenses or misalignment. However, there is a principal limit to the resolution of any optical system, due to the physics of diffraction. An optical system with resolution performance at the instrument's theoretical limit is said to be diffraction-limited.

In optics, the Airy disk and Airy pattern are descriptions of the best-focused spot of light that a perfect lens with a circular aperture can make, limited by the diffraction of light. The Airy disk is of importance in physics, optics, and astronomy.

Magnification is the process of enlarging the apparent size, not physical size, of something. This enlargement is quantified by a calculated number also called "magnification". When this number is less than one, it refers to a reduction in size, sometimes called minification or de-magnification.

The point spread function (PSF) describes the response of an imaging system to a point source or point object. A more general term for the PSF is a system's impulse response, the PSF being the impulse response of a focused optical system. The PSF in many contexts can be thought of as the extended blob in an image that represents a single point object. In functional terms, it is the spatial domain version of the optical transfer function of the imaging system. It is a useful concept in Fourier optics, astronomical imaging, medical imaging, electron microscopy and other imaging techniques such as 3D microscopy and fluorescence microscopy.
Optical resolution describes the ability of an imaging system to resolve detail in the object that is being imaged.
The spectral resolution of a spectrograph, or, more generally, of a frequency spectrum, is a measure of its ability to resolve features in the electromagnetic spectrum. It is usually denoted by , and is closely related to the resolving power of the spectrograph, defined as

The optical transfer function (OTF) of an optical system such as a camera, microscope, human eye, or projector specifies how different spatial frequencies are handled by the system. It is used by optical engineers to describe how the optics project light from the object or scene onto a photographic film, detector array, retina, screen, or simply the next item in the optical transmission chain. A variant, the modulation transfer function (MTF), neglects phase effects, but is equivalent to the OTF in many situations.
High-resolution transmission electron microscopy (HRTEM) is an imaging mode of specialized transmission electron microscopes (TEMs) that allows for direct imaging of the atomic structure of the sample. HRTEM is a powerful tool to study properties of materials on the atomic scale, such as semiconductors, metals, nanoparticles and sp2-bonded carbon. While HRTEM is often also used to refer to high resolution scanning TEM, this article describes mainly the imaging of an object by recording the 2D spatial wave amplitude distribution in the image plane, in analogy to a "classic" light microscope. For disambiguation, the technique is also often referred to as phase contrast TEM. At present, the highest point resolution realised in phase contrast TEM is around 0.5 ångströms (0.050 nm). At these small scales, individual atoms of a crystal and its defects can be resolved. For 3-dimensional crystals, it may be necessary to combine several views, taken from different angles, into a 3D map. This technique is called electron crystallography.
The Strehl ratio is a measure of the quality of optical image formation, originally proposed by Karl Strehl, after whom the term is named. Used variously in situations where optical resolution is compromised due to lens aberrations or due to imaging through the turbulent atmosphere, the Strehl ratio has a value between 0 and 1, with a hypothetical, perfectly unaberrated optical system having a Strehl ratio of 1.

Acousto-optics is a branch of physics that studies the interactions between sound waves and light waves, especially the diffraction of laser light by ultrasound through an ultrasonic grating.
The Fried parameter or Fried's coherence length is a measure of the quality of optical transmission through the atmosphere due to random inhomogeneities in the atmosphere's refractive index. In practice, such inhomogeneities are primarily due to tiny variations in temperature on smaller spatial scales resulting from random turbulent mixing of larger temperature variations on larger spatial scales as first described by Kolmogorov. The Fried parameter has units of length and is typically expressed in centimeters. It is defined as the diameter of a circular area over which the rms wavefront aberration due to passage through the atmosphere is equal to 1 radian. For a telescope with an aperture, , the smallest spot that can be observed is given by the telescopes Point spread function (PSF). Atmospheric turbulence increases the diameter of the smallest spot by a factor approximately . As such, imaging from telescopes with apertures much smaller than is less affected by atmospheric seeing than diffraction due to the telescope's small aperture. However, the imaging resolution of telescopes with apertures much larger than will be limited by the turbulent atmosphere, preventing the instruments from approaching the diffraction limit.
Super-resolution photoacoustic imaging is a set of techniques used to enhance spatial resolution in photoacoustic imaging. Specifically, these techniques primarily break the optical diffraction limit of the photoacoustic imaging system. It can be achieved in a variety of mechanisms, such as blind structured illumination, multi-speckle illumination, or photo-imprint photoacoustic microscopy in Figure 1.














