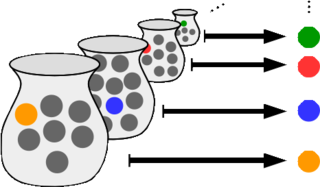
In mathematics, the axiom of choice, abbreviated AC or AoC, is an axiom of set theory equivalent to the statement that a Cartesian product of a collection of non-empty sets is non-empty. Informally put, the axiom of choice says that given any collection of sets, each containing at least one element, it is possible to construct a new set by choosing one element from each set, even if the collection is infinite. Formally, it states that for every indexed family of nonempty sets, there exists an indexed set such that for every . The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem. The axiom of choice is equivalent to the statement that every partition has a transversal.

Euclidean geometry is a mathematical system attributed to ancient Greek mathematician Euclid, which he described in his textbook on geometry, Elements. Euclid's approach consists in assuming a small set of intuitively appealing axioms (postulates) and deducing many other propositions (theorems) from these. Although many of Euclid's results had been stated earlier, Euclid was the first to organize these propositions into a logical system in which each result is proved from axioms and previously proved theorems.
Mathematical logic is the study of formal logic within mathematics. Major subareas include model theory, proof theory, set theory, and recursion theory. Research in mathematical logic commonly addresses the mathematical properties of formal systems of logic such as their expressive or deductive power. However, it can also include uses of logic to characterize correct mathematical reasoning or to establish foundations of mathematics.

In geometry, the Wallace–Bolyai–Gerwien theorem, named after William Wallace, Farkas Bolyai and P. Gerwien, is a theorem related to dissections of polygons. It answers the question when one polygon can be formed from another by cutting it into a finite number of pieces and recomposing these by translations and rotations. The Wallace–Bolyai–Gerwien theorem states that this can be done if and only if two polygons have the same area.
In mathematics, a non-measurable set is a set which cannot be assigned a meaningful "volume". The existence of such sets is construed to provide information about the notions of length, area and volume in formal set theory. In Zermelo–Fraenkel set theory, the axiom of choice entails that non-measurable subsets of exist.
The Hausdorff paradox is a paradox in mathematics named after Felix Hausdorff. It involves the sphere . It states that if a certain countable subset is removed from , then the remainder can be divided into three disjoint subsets and such that and are all congruent. In particular, it follows that on there is no finitely additive measure defined on all subsets such that the measure of congruent sets is equal.
In mathematics, an amenable group is a locally compact topological group G carrying a kind of averaging operation on bounded functions that is invariant under translation by group elements. The original definition, in terms of a finitely additive measure on subsets of G, was introduced by John von Neumann in 1929 under the German name "messbar" in response to the Banach–Tarski paradox. In 1949 Mahlon M. Day introduced the English translation "amenable", apparently as a pun on "mean".
In mathematics, a fixed-point theorem is a result saying that a function F will have at least one fixed point (a point x for which F(x) = x), under some conditions on F that can be stated in general terms.
In the mathematical discipline of measure theory, a Banach measure is a certain way to assign a size to all subsets of the Euclidean plane, consistent with but extending the commonly used Lebesgue measure. While there are certain subsets of the plane which are not Lebesgue measurable, all subsets of the plane have a Banach measure. On the other hand, the Lebesgue measure is countably additive while a Banach measure is only finitely additive.
In geometry, a dissection problem is the problem of partitioning a geometric figure into smaller pieces that may be rearranged into a new figure of equal content. In this context, the partitioning is called simply a dissection. It is usually required that the dissection use only a finite number of pieces. Additionally, to avoid set-theoretic issues related to the Banach–Tarski paradox and Tarski's circle-squaring problem, the pieces are typically required to be well-behaved. For instance, they may be restricted to being the closures of disjoint open sets.

Matthew Dean Foreman is an American mathematician at University of California, Irvine. He has made notable contributions in set theory and in ergodic theory.

Miklós Laczkovich is a Hungarian mathematician mainly noted for his work on real analysis and geometric measure theory. His most famous result is the solution of Tarski's circle-squaring problem in 1989.
In set theory, a paradoxical set is a set that has a paradoxical decomposition. A paradoxical decomposition of a set is two families of disjoint subsets, along with appropriate group actions that act on some universe, such that each partition can be mapped back onto the entire set using only finitely many distinct functions to accomplish the mapping. A set that admits such a paradoxical decomposition where the actions belong to a group is called -paradoxical or paradoxical with respect to .
This article contains a discussion of paradoxes of set theory. As with most mathematical paradoxes, they generally reveal surprising and counter-intuitive mathematical results, rather than actual logical contradictions within modern axiomatic set theory.
The Banach–Tarski paradox is a theorem in set-theoretic geometry, which states the following: Given a solid ball in three-dimensional space, there exists a decomposition of the ball into a finite number of disjoint subsets, which can then be put back together in a different way to yield two identical copies of the original ball. Indeed, the reassembly process involves only moving the pieces around and rotating them, without changing their original shape. However, the pieces themselves are not "solids" in the traditional sense, but infinite scatterings of points. The reconstruction can work with as few as five pieces.
In mathematics, the von Neumann paradox, named after John von Neumann, is the idea that one can break a planar figure such as the unit square into sets of points and subject each set to an area-preserving affine transformation such that the result is two planar figures of the same size as the original. This was proved in 1929 by John von Neumann, assuming the axiom of choice. It is based on the earlier Banach–Tarski paradox, which is in turn based on the Hausdorff paradox.

Henry Perigal, Jr. FRAS MRI was a British stockbroker and amateur mathematician, known for his dissection-based proof of the Pythagorean theorem and for his unorthodox belief that the moon does not rotate.

The Banach–Tarski Paradox is a book in mathematics on the Banach–Tarski paradox, the fact that a unit ball can be partitioned into a finite number of subsets and reassembled to form two unit balls. It was written by Stan Wagon and published in 1985 by the Cambridge University Press as volume 24 of their Encyclopedia of Mathematics and its Applications book series. A second printing in 1986 added two pages as an addendum, and a 1993 paperback printing added a new preface. In 2016 the Cambridge University Press published a second edition, adding Grzegorz Tomkowicz as a co-author, as volume 163 of the same series. The Basic Library List Committee of the Mathematical Association of America has recommended its inclusion in undergraduate mathematics libraries.

Combinatorial Geometry in the Plane is a book in discrete geometry. It was translated from a German-language book, Kombinatorische Geometrie in der Ebene, which its authors Hugo Hadwiger and Hans Debrunner published through the University of Geneva in 1960, expanding a 1955 survey paper that Hadwiger had published in L'Enseignement mathématique. Victor Klee translated it into English, and added a chapter of new material. It was published in 1964 by Holt, Rinehart and Winston, and republished in 1966 by Dover Publications. A Russian-language edition, Комбинаторная геометрия плоскости, translated by I. M. Jaglom and including a summary of the new material by Klee, was published by Nauka in 1965. The Basic Library List Committee of the Mathematical Association of America has recommended its inclusion in undergraduate mathematics libraries.








