
Radar is a system that uses radio waves to determine the distance (ranging), direction, and radial velocity of objects relative to the site. It is a radiodetermination method used to detect and track aircraft, ships, spacecraft, guided missiles, motor vehicles, map weather formations, and terrain.

In electronics, acoustics, and related fields, the waveform of a signal is the shape of its graph as a function of time, independent of its time and magnitude scales and of any displacement in time. Periodic waveforms repeat regularly at a constant period. The term can also be used for non-periodic or aperiodic signals, like chirps and pulses.

A multistatic radar system contains multiple spatially diverse monostatic radar or bistatic radar components with a shared area of coverage. An important distinction of systems based on these individual radar geometries is the added requirement for some level of data fusion to take place between component parts. The spatial diversity afforded by multistatic systems allows different aspects of a target to be viewed simultaneously. The potential for information gain can give rise to a number of advantages over conventional systems.

Synthetic-aperture radar (SAR) is a form of radar that is used to create two-dimensional images or three-dimensional reconstructions of objects, such as landscapes. SAR uses the motion of the radar antenna over a target region to provide finer spatial resolution than conventional stationary beam-scanning radars. SAR is typically mounted on a moving platform, such as an aircraft or spacecraft, and has its origins in an advanced form of side looking airborne radar (SLAR). The distance the SAR device travels over a target during the period when the target scene is illuminated creates the large synthetic antenna aperture. Typically, the larger the aperture, the higher the image resolution will be, regardless of whether the aperture is physical or synthetic – this allows SAR to create high-resolution images with comparatively small physical antennas. For a fixed antenna size and orientation, objects which are further away remain illuminated longer – therefore SAR has the property of creating larger synthetic apertures for more distant objects, which results in a consistent spatial resolution over a range of viewing distances.
In pulsed radar and sonar signal processing, an ambiguity function is a two-dimensional function of propagation delay and Doppler frequency , . It represents the distortion of a returned pulse due to the receiver matched filter of the return from a moving target. The ambiguity function is defined by the properties of the pulse and of the filter, and not any particular target scenario.
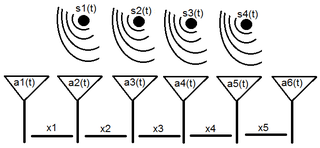
Array processing is a wide area of research in the field of signal processing that extends from the simplest form of 1 dimensional line arrays to 2 and 3 dimensional array geometries. Array structure can be defined as a set of sensors that are spatially separated, e.g. radio antenna and seismic arrays. The sensors used for a specific problem may vary widely, for example microphones, accelerometers and telescopes. However, many similarities exist, the most fundamental of which may be an assumption of wave propagation. Wave propagation means there is a systemic relationship between the signal received on spatially separated sensors. By creating a physical model of the wave propagation, or in machine learning applications a training data set, the relationships between the signals received on spatially separated sensors can be leveraged for many applications.

Beamforming or spatial filtering is a signal processing technique used in sensor arrays for directional signal transmission or reception. This is achieved by combining elements in an antenna array in such a way that signals at particular angles experience constructive interference while others experience destructive interference. Beamforming can be used at both the transmitting and receiving ends in order to achieve spatial selectivity. The improvement compared with omnidirectional reception/transmission is known as the directivity of the array.
Passive radar is a class of radar systems that detect and track objects by processing reflections from non-cooperative sources of illumination in the environment, such as commercial broadcast and communications signals. It is a specific case of bistatic radar – passive bistatic radar (PBR) – which is a broad type also including the exploitation of cooperative and non-cooperative radar transmitters.
Pulse compression is a signal processing technique commonly used by radar, sonar and echography to either increase the range resolution when pulse length is constrained or increase the signal to noise ratio when the peak power and the bandwidth of the transmitted signal are constrained. This is achieved by modulating the transmitted pulse and then correlating the received signal with the transmitted pulse.
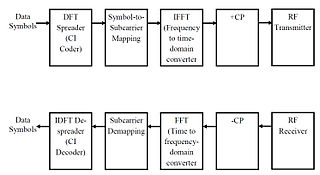
Carrier Interferometry(CI) is a spread spectrum scheme designed to be used in an Orthogonal Frequency-Division Multiplexing (OFDM) communication system for multiplexing and multiple access, enabling the system to support multiple users at the same time over the same frequency band.
Active sensory systems are sensory receptors that are activated by probing the environment with self-generated energy. Examples include echolocation of bats and dolphins and insect antennae. Using self-generated energy allows more control over signal intensity, direction, timing and spectral characteristics. By contrast, passive sensory systems involve activation by ambient energy. For example, human vision relies on using light from the environment.

Peter (Petre) Stoica is a researcher and educator in the field of signal processing and its applications to radar/sonar, communications and bio-medicine. He is a professor of Signals and Systems Modeling at Uppsala University in Sweden, and a Member of the Royal Swedish Academy of Engineering Sciences, the United States National Academy of Engineering, the Romanian Academy, the European Academy of Sciences, and the Royal Society of Sciences in Uppsala. He is also a Fellow of IEEE, EURASIP, IETI, and the Royal Statistical Society.

Shannon D. Blunt is an American radar engineer and the Roy A. Roberts Distinguished Professor of Electrical Engineering & Computer Science at the University of Kansas (KU) in Lawrence, KS. He is Director of the KU Radar Systems & Remote Sensing Lab (RSL) and the Kansas Applied Research Lab (KARL).

Sergio Barbarossa is an Italian professor, engineer and inventor. He is a professor at Sapienza University of Rome, Italy.

Multiple-input multiple-output (MIMO) radar is an extension of a traditional radar system to utilize multiple-inputs and multiple-outputs (antennas), similar to MIMO techniques used to increase the capacity of a radio link. MIMO radar is an advanced type of phased array radar employing digital receivers and waveform generators distributed across the aperture. MIMO radar signals propagate in a fashion similar to multistatic radar. However, instead of distributing the radar elements throughout the surveillance area, antennas are closely located to obtain better spatial resolution, Doppler resolution, and dynamic range. MIMO radar may also be used to obtain low-probability-of-intercept radar properties.
SAMV is a parameter-free superresolution algorithm for the linear inverse problem in spectral estimation, direction-of-arrival (DOA) estimation and tomographic reconstruction with applications in signal processing, medical imaging and remote sensing. The name was coined in 2013 to emphasize its basis on the asymptotically minimum variance (AMV) criterion. It is a powerful tool for the recovery of both the amplitude and frequency characteristics of multiple highly correlated sources in challenging environments. Applications include synthetic-aperture radar, computed tomography scan, and magnetic resonance imaging (MRI).
Vishal Monga is an Indian American electrical engineer, researcher and academic. He is a professor of Electrical Engineering at the Pennsylvania State University.

Daniel W. Bliss is an American professor, engineer, and physicist. He is a Fellow of the IEEE and was awarded the IEEE Warren D. White award for outstanding technical advances in the art of radar engineering in 2021 for his contributions to MIMO radar, Multiple-Function Sensing and Communications Systems, and Novel Small-Scale Radar Applications. He is a professor in the School of Electrical, Computer and Energy Engineering at Arizona State University. He is also the director of the Center for Wireless Information Systems and Computational Architecture (WISCA).
Jian Li is a Chinese-American electrical engineer known for her research in signal processing, spectral density estimation, and MIMO radar, and for her books on engineering She is a professor of electrical and computer engineering at the University of Florida, where she directs the Spectral Analysis Laboratory.

Athina Petropulu is a Greek electrical engineer, researcher and academic. She is Distinguished Professor in the Electrical and Computer Engineering (ECE) Department at Rutgers, The State University of New Jersey. She has made contributions in signal processing, wireless communications and networks, and radar systems. She received many awards for her work in these areas.












