
In mathematics and physics, a soliton or solitary wave is a self-reinforcing wave packet that maintains its shape while it propagates at a constant velocity. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium. Solitons are the solutions of a widespread class of weakly nonlinear dispersive partial differential equations describing physical systems.
The sine-Gordon equation is a nonlinear hyperbolic partial differential equation in 1 + 1 dimensions involving the d'Alembert operator and the sine of the unknown function. It was originally introduced by Edmond Bour (1862) in the course of study of surfaces of constant negative curvature as the Gauss–Codazzi equation for surfaces of curvature −1 in 3-space, and rediscovered by Frenkel and Kontorova (1939) in their study of crystal dislocations known as the Frenkel–Kontorova model. This equation attracted a lot of attention in the 1970s due to the presence of soliton solutions.
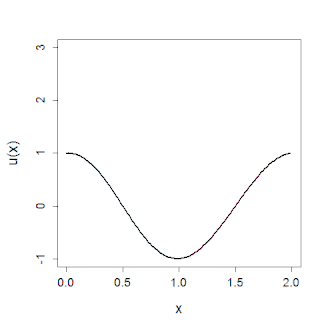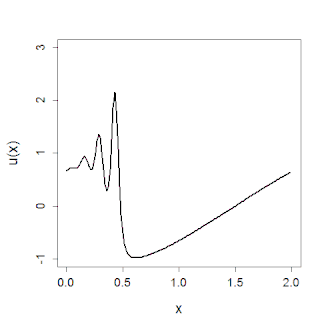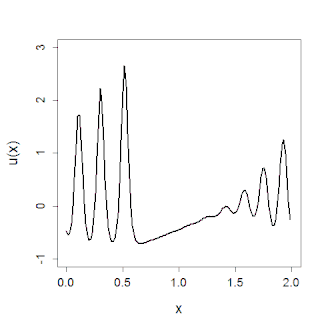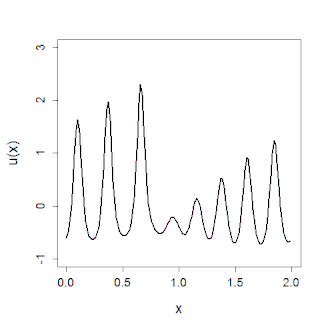
In mathematics, the Korteweg–De Vries (KdV) equation is a mathematical model of waves on shallow water surfaces. It is particularly notable as the prototypical example of an exactly solvable model, that is, a non-linear partial differential equation whose solutions can be exactly and precisely specified. KdV can be solved by means of the inverse scattering transform. The mathematical theory behind the KdV equation is a topic of active research. The KdV equation was first introduced by Boussinesq and rediscovered by Diederik Korteweg and Gustav de Vries (1895).

Martin David Kruskal was an American mathematician and physicist. He made fundamental contributions in many areas of mathematics and science, ranging from plasma physics to general relativity and from nonlinear analysis to asymptotic analysis. His most celebrated contribution was in the theory of solitons.
The Bôcher Memorial Prize was founded by the American Mathematical Society in 1923 in memory of Maxime Bôcher with an initial endowment of $1,450. It is awarded every three years for a notable research memoir in analysis that has appeared during the past six years in a recognized North American journal or was authored by a member of the Society. This provision, introduced in 1971 and modified in 1993, is a liberalization of the terms of the award. The current award is $5,000.

In theoretical physics, the (one-dimensional) nonlinear Schrödinger equation (NLSE) is a nonlinear variation of the Schrödinger equation. It is a classical field equation whose principal applications are to the propagation of light in nonlinear optical fibers and planar waveguides and to Bose–Einstein condensates confined to highly anisotropic cigar-shaped traps, in the mean-field regime. Additionally, the equation appears in the studies of small-amplitude gravity waves on the surface of deep inviscid (zero-viscosity) water; the Langmuir waves in hot plasmas; the propagation of plane-diffracted wave beams in the focusing regions of the ionosphere; the propagation of Davydov's alpha-helix solitons, which are responsible for energy transport along molecular chains; and many others. More generally, the NLSE appears as one of universal equations that describe the evolution of slowly varying packets of quasi-monochromatic waves in weakly nonlinear media that have dispersion. Unlike the linear Schrödinger equation, the NLSE never describes the time evolution of a quantum state. The 1D NLSE is an example of an integrable model.
In mathematics, integrability is a property of certain dynamical systems. While there are several distinct formal definitions, informally speaking, an integrable system is a dynamical system with sufficiently many conserved quantities, or first integrals, such that its behaviour has far fewer degrees of freedom than the dimensionality of its phase space; that is, its evolution is restricted to a submanifold within its phase space.

In mathematics and physics, the Kadomtsev–Petviashvili equation is a partial differential equation to describe nonlinear wave motion. Named after Boris Borisovich Kadomtsev and Vladimir Iosifovich Petviashvili, the KP equation is usually written as:
In mathematics, the inverse scattering transform is a method for solving some non-linear partial differential equations. It is one of the most important developments in mathematical physics in the past 40 years. The method is a non-linear analogue, and in some sense generalization, of the Fourier transform, which itself is applied to solve many linear partial differential equations. The name "inverse scattering method" comes from the key idea of recovering the time evolution of a potential from the time evolution of its scattering data: inverse scattering refers to the problem of recovering a potential from its scattering matrix, as opposed to the direct scattering problem of finding the scattering matrix from the potential.
In mathematical physics, the Degasperis–Procesi equation

In fluid dynamics, the Camassa–Holm equation is the integrable, dimensionless and non-linear partial differential equation
Dispersionless limits of integrable partial differential equations (PDE) arise in various problems of mathematics and physics and have been intensively studied in recent literature. They typically arise when considering slowly modulated long waves of an integrable dispersive PDE system.
In mathematics, the Novikov–Veselov equation is a natural (2+1)-dimensional analogue of the Korteweg–de Vries (KdV) equation. Unlike another (2+1)-dimensional analogue of KdV, the Kadomtsev–Petviashvili equation, it is integrable via the inverse scattering transform for the 2-dimensional stationary Schrödinger equation. Similarly, the Korteweg–de Vries equation is integrable via the inverse scattering transform for the 1-dimensional Schrödinger equation. The equation is named after S.P. Novikov and A.P. Veselov who published it in Novikov & Veselov (1984).
In mathematical physics, the Whitham equation is a non-local model for non-linear dispersive waves.

Alessio Figalli is an Italian mathematician working primarily on calculus of variations and partial differential equations.
Frank Merle is a French mathematician, specializing in partial differential equations and mathematical physics.
The Kundu equation is a general form of integrable system that is gauge-equivalent to the mixed nonlinear Schrödinger equation. It was proposed by Anjan Kundu as
Monica Vișan is a Romanian mathematician at the University of California, Los Angeles who specialized in PDE and is well known for her work on the nonlinear Schrödinger equation.

Joris van der Hoeven is a Dutch mathematician and computer scientist, specializing in algebraic analysis and computer algebra.
The Centre de mathématiques Laurent-Schwartz (CMLS) is a joint research unit of France's Centre national de la recherche scientifique (CNRS) and the École Polytechnique. It is located on the site of École Polytechnique in Palaiseau.











